F1.2 Estimate and calculate the cost of transactions involving multiple items priced in whole-dollar amounts, not including sales tax, and the amount of change needed when payment is made in cash, using mental math.
Skill: Estimating and Calculating the Cost of Transactions Involving Multiple Items (Without Tax)
In the early junior grades, students build on prior knowledge to estimate and calculate transactions involving multiple items with dollar values, excluding sales tax. Estimating and calculating the costs of cash transactions requires the application of addition, subtraction, and mental math strategies, as well as a good knowledge of math facts.
When purchasing multiple items, students round up the price of each item and then add them up to get a general idea of what the transaction will cost.
Students then compare their estimation of the transaction with the exact cost. In this way, they can check whether their answer is plausible.
A culturally relevant and responsive environment helps develop an understanding of how money is used in everyday life.
Teachers are encouraged to provide multiple opportunities for students to apply mental math in realistic learning situations, which develops their ability to recall math facts and strengthens their knowledge and understanding of operations. These opportunities provide a meaningful context in which students apply mental math strategies to significantly improve the efficiency and accuracy of their calculations.
Example
Hamzah is making a list of gym equipment with her classmates. They need to find five play items that students use at recess. Their teacher has given them a budget of $300. In searching, these students found a basketball for $29, jump ropes for $7, a play parachute for $59, a set of flying discs for $54, soccer nets for $89. Did the students spend more or less than the allotted budget?
Strategy
First, I need to estimate the total cost of the chosen equipment.
I round each price to the nearest 10 and add them up to get my estimation.
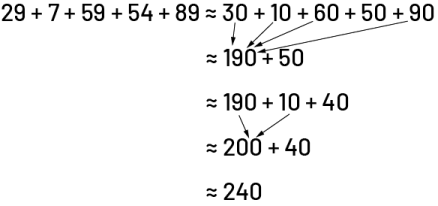
29 plus, 7 plus, 59 plus, 54 plus, 89 plus or minus equals 30 plus, ten plus, 60 plus, 50 plus 90. All numbers to the right of equal plus or minus have an arrow pointing to 190 plus 50. More or less equals 190 plus ten plus 40. More or less equals 200 plus 40. More or less equal to 240.
\(\displaylines{\begin{align} 29 + 7 + 59 + 54 + 89 &\approx \underline{30 + 10 + 60 + 90} + 50 \\ &\approx 190 + 50 \\ &\approx \underline{190 + 10 } + 40 \\ &\approx 200 + 40 \\ &\approx 240 \end{align}}\)
According to my estimation, there should be enough money for all the items.
Now I calculate the actual cost of the transaction using decomposition.
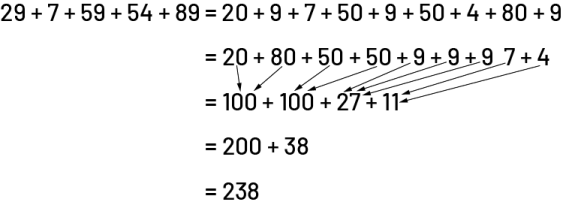
29 plus 7 plus, 59 plus 54 plus 89 equals 20 plus, 9 plus 7 plus, 50 plus, 9 plus, 50 plus, 4 plus 80 plus, 9 Equals 20, plus 80 plus, 50 plus, 50 plus 9 plus, 9 plus, 9 plus 7 plus 4. Equals 100 plus 100 plus 27 plus 11. The top row digits have been added by two. Equals 200 plus 38. Equal 238
\(\displaylines{\begin{align} 29 + 7 + 59 + 54 + 89 &= 20 + 9 + 7 + 50 + 9 + 50 + 4 + 80 + 9 \\ &=\underline{20 + 80 }+\underline{50 + 50} +\underline{9 + 9 + 9 } +\underline{7 + 4} \\ &= + 100 + 100 + 27 + 11 \\ &= 200 + 38 \\ &= 238 \end{align}}\)
The cost of the transaction is $238. If the budget is $300, there is enough money to buy all the items on the list and there will be money left over.
I can calculate the change to be given using mental math.
I know that 300 minus 200 is 100. Then I know that 100-30 is 70. Finally, I subtract 8 from 70, which gives me 62.
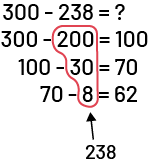
300 minus 238 equals unknown 300 minus 200 equals 100 100 minus 30 equals 70 70 minus 8 equals 62 The 200, 30 and 8 are circled and added together, we get 238.
There is $62 left in the budget. Students could find another item under $62.
Knowledge: Transactions
Transactions are payments in cash or other methods of payment in exchange for certain goods or services.
Transactions can be made through a variety of methods of payment, including cash, cheque, credit or debit card, electronic transfer, gift card and online payment services.
Only cash transactions are rounded to the nearest multiple of 5 cents, since the 1¢ coin is no longer in circulation.
Knowledge: Cash
Money in the form of cash (bills or coins) is one way to pay for goods or services. There are $2, $1, 25¢, 10¢ and 5¢ coins, and there are $100, $50, $20, $10 and $5 bills.
Most merchants accept cash to pay for goods and services. Cash purchases can be more confidential because there is no disclosure of financial information, such as debit or credit card numbers. However, if the cash is lost or stolen, the chances of getting it back are slim.
