C2.3 Identify and use equivalent relationships for whole numbers up to 100, in various contexts.
Skill: Determining and Using Equivalent Relationships Involving Whole Numbers up to 100
Developing algebraic thinking is a complex process that relies on three fundamental processes: abstracting, generalizing and operating on the unknown.
Although the properties of operations (for example, commutativity) are explored in the Numbers strand, the process of generalizing the properties requires algebraic thinking.
Generalize
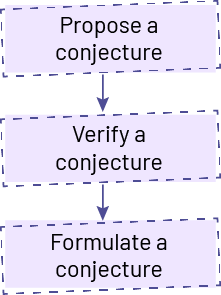
In situations of equality, students can formulate a generalization when it follows a process of proposing and verifying a conjecture.
A conjecture is the expression of an idea that is perceived to be true in all similar situations.
When students see a recursive phenomenon while exploring various equality situations, they are able to propose a conjecture; for example, they might say that if you add the number 0 to any number, the initial quantity does not change.
Students should then check whether their conjecture is valid in other similar situations. For example, in the situation in the previous example, the conjecture could be tested using various numbers as well as concrete materials.
When a conjecture seems to apply to all similar situations, students formulate a generalization in words or using symbols.
Example 1
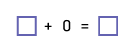
There are three important steps in the generalization process.
In the primary grades, conjectures are usually expressed in words by students. They may also be represented using concrete or visual (semi-concrete) materials to illustrate mathematical reasoning as clearly as possible.
It is important to expose students to a variety of problem situations that encourage them to practice the skill of making and testing a conjecture; for example, present them with the number sentence 50 + 6 - 6 = 50, and ask them what they notice. Then, propose the following conjecture to them: When you add and subtract the same number in a number sentence, is it the same to add or subtract zero. Then invite students to discuss this conjecture and determine if it is still true.
Students test this conjecture with other number sentences. They may not be convinced that it applies to any number sentence or to all numbers, especially large numbers. In the course of the discussion, they can propose their own conjectures as illustrated below.

After a check of various number sentences, students conclude that the conjecture is true and formulate a generalization.
Example 2

Since students' vocabulary in elementary school is not yet very developed and precise, the first conjectures usually need to be reformulated or clarified. It is therefore ideal to practice formulating a conjecture as a class, as shown in the example below. In the discussion, students can point out the limitations of someone else's conjecture and contribute to the formulation of a clearer and more relevant common conjecture. It is important, however, to establish a learning environment in which students perceive the questions of others as positive interactions that can fuel the exchange.
Example 3
Present students with the number sentence 54 + 0 = 54 and ask them if it is true or false.
- Student: The number sentence is true.
- Faculty: How can you tell?
- Student: When a zero is added to a number, it doesn't actually add anything, so we get the starting number.
Present the students with other similar number sentences. After several such exchanges, ask them to formulate a conjecture.
- Student: All numbers added with a zero remain the same.
- Another student presents a counterexample: No, 10 + 10 = 20. The numbers 10 and 10 have zeros in them. When added together, they do not remain the same.
After further discussion, a student formulates another conjecture:
- Student: When you add a zero to another number, you get the other number.
- Other student: That's not true.
- Teacher: So, are you referring to the number that is right next to a zero?
- Student: No, added to another number.
After much discussion, the following formulation is adopted: Zero, added to another number, is equal to that number. When students see that this conjecture applies to all numbers, they can generalize.
Source: translated from Guide d’enseignement efficace des mathématiques de la maternelle à la 3e année, Modélisation et algèbre, Fascicule 2, Situations d'égalité, p. 8-11.
When numbers are decomposed, the sum of the parts is equivalent to the whole.
Remark: Commutativity and associativity allow students to see the equality of a number sentence, demonstrate their understanding of these properties, and see the equality of a mathematical expression without performing calculations.
Students are not expected to be able to name or define these properties, but to use them appropriately.
Source: translated from Curriculum de l'Ontario, Programme de mathématiques de la 1re à la 8e année, 2020, Ministère de l'Éducation de l'Ontario.
Skills Related to Equivalent Relationships
In the primary grades, students develop the ability to recognize, explain, create, re-establish, and maintain equivalent relationships through the application of strategies and models (for example, ten frames, number line). These skills are to be developed in each grade using progressively larger numbers, consistent with curriculum requirements.
Initially, equalities and inequalities are explored primarily verbally and with concrete materials. Later, students are gradually exposed to symbolic representation; however, the use of concrete materials remains just as important and must be used in conjunction with the more abstract representations.
Skill: Recognizing an Equivalent Relationship
In problem solving, the use of concrete and visual (semi-concrete) materials, as well as models, allows students to recognize and represent situations of equality and inequality.
Examples
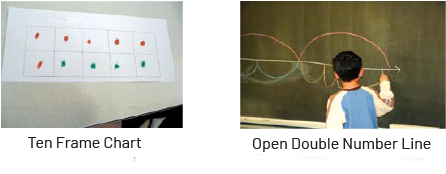
It is only after having manipulated various models several times with the same goal, that is, to recognize a situation of equality or inequality, that students are able to approach the purely symbolic representation (the mathematical expression) of this situation. Furthermore, in order to determine the nature of the relationship between the quantities, students need to understand that the items on either side of the equal sign are data to be analyzed, not just expressions to be calculated.
Example 1
Exploring the commutativity property allows students to see the equality of the number sentence 43 + 24 = 24 + 43. It is important, through interventions, to encourage students to observe that the same numbers are on both sides of the equality symbol and that the addition terms are simply switched.
Example 2
The strategy of canceling terms or equal expressions allows students to see the equality of a mathematical expression. Canceling terms involves crossing out the identical terms that appear on either side of the equal sign. Crossing out or canceling terms that are on either side of the equal sign makes it easier to establish relationships between the remaining terms and helps students develop their algebraic reasoning. For example:

Source: translated from Guide d’enseignement efficace des mathématiques de la maternelle à la 3e année, Modélisation et algèbre, Fascicule 2, Situations d'égalité, p. 39-41.
Skill: Explaining a Situation of Equality
In order to develop their ability to explain a situation of equality, students need to address different steps. The transfer from concrete to symbolic representation is easier when the equality relationship is constructed by following these different steps.
- Explore using concrete or visual (semi-concrete) materials.
The student demonstrates, using toys, the following situation: adding 0 toys to 10 toys. - Describe using words and materials.
Student says, "If I add 0 toys to 10 toys, the quantity will not change, since I am not adding anything. It will still equal 10 toys." - Represent using symbols.
The student expresses equality symbolically by writing the number sentence 10 + 0 = 10. - Generalize to all numbers
Appropriate questions in other similar situations lead the student to generalize that adding 0 to any quantity does not change the quantity.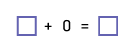
Students also develop their ability to explain a situation of equality by using models. Indeed, the use of models allows students to communicate their reasoning effectively.
Example
To explain the expression 27 + 5 - 5 = 27, students can use an open number line to support their reasoning: "I take a leap 27 and add a leap of 5. I take another leap of 5 in the other direction, so I am back to 27. It is as if I never added a leap of 5."

Source: translated from Guide d’enseignement efficace des mathématiques de la maternelle à la 3e année, Modélisation et algèbre, Fascicule 2, Situations d'égalité, p. 41-42.
Skill: Creating a Situation of Equality
To get students to achieve equality, it is important to initially present them with a situation in which concrete materials are used. Ask them to represent it with a number sentence, compare the different expressions proposed by the other students and determine if they are all true.
Examples
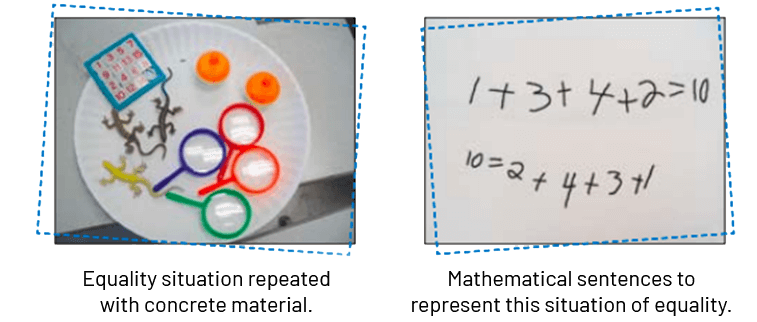
Afterwards, students can create their own equality situation. They should be given the opportunity to write number sentences and represent them with concrete materials. Students should have the opportunity to create equality situations represented by number sentences made up of large numbers in order to be able to use the properties of operations or strategies instead of calculation.

Source: translated from Guide d’enseignement efficace des mathématiques de la maternelle à la 3e année, Modélisation et algèbre, Fascicule 2, Situations d'égalité, p. 42-43.
Knowledge: Equivalent Relationships
A relationship that compares quantities to show that they have the same value.
Example
352 is equivalent to 350 and 2.
Source: translated from En avant, les maths! 3e, CM, Algèbre, p. 2.
Examples
- Represent a number in different ways using rods.
- Represent a number in different ways using base 10 materials.
- Represent a number in different ways using a Rekenrek.
- …
Knowledge: Equality
Relationship between two equal quantities.
Knowledge: Number Sentence
A symbolic representation that represents a relationship. In a number sentence, there is no unknown or variable.
Example
True (equal) sentences \(75 + 5 = 5 + 75\) or \(50 = 20 + 20 + 10\)
False sentence (inequal) \(100 = 95 - 5\) or \(45 + 10 = 15 + 45\)
