La vue d'ensemble

At a Glance...

The Guides for Effective Instruction in Mathematics Revised

FrançaisEnglish

C1.1 Identify and describe repeating elements and operations in a variety of patterns, including patterns found in real-life contexts.

Skill: Recognizing Elements and Operations that are Repeated in Various Patterns

Repeating Patterns

The non-numeric repeating pattern is the simplest form of pattern. To recognize it, you have to look for the pattern core. It is created when the elements that make up the pattern are repeated in the same order. Students must learn to identify the beginning and end of the pattern core. For example, in the picture below, the student who created this pattern shows by leaving a space between the pattern cores that the orange bead indicates the beginning of the pattern core and the red bead, the end.

A sequence of wooden beads makes a necklace. From left to right: orange, green, red, repeated 3 times.

In addition, it is important for students to "read" the pattern by naming and touching each consecutive element of the pattern to identify the repetition.

In the Primary Division, students explore various types of patterns. In the junior grades students start to classify patterns.

Here is a suggested approach to teaching repeating patterns:

  • Step 1: Observe a pattern with a single attribute and a two-element core.

First explore a pattern that has only one attribute and whose core consists of only two elements. The attribute could be motion, position, sound, shape, or colour.

Example 

6 children create a sequence with their bodies. The pattern is kneeling, standing, repeated 2 times.

In the pattern shown above, the attribute is position: one element on all fours and one element standing with arms facing forward constitute the pattern core.

Note: Colour change is easier to recognize and describe when a pattern is constructed with concrete materials, especially using objects that all have the same shape such as beads on a necklace, algebraic tiles, or nesting cubes.

  • Step 2: Change the attribute.

Then explore patterns with another attribute such as shape or size, while still having two elements in the pattern.

Example 

A sequence of a non-numeric repeating patterns: sailboat, beach ball, boat, beach ball, sail boat, and beach ball.

In the pattern shown above, the attribute is the shape. The two elements, the sailboat and the beachball, constitute the pattern core.

  • Step 3: Modify the structure of the pattern.

Explore more complex patterns by adding more elements to the pattern or more attributes. Students will then face a cognitive challenge that will lead to new learning.

Example

The following pattern has two attributes, shape and colour, and a three-element core, blue rectangle, blue rectangle, yellow triangle. Its structure is AAB.

A sequence of a non-numeric repeating pattern with forms: 2 blue rectangle, one orange triangle, repeated 4 times.

Continue by introducing a third shape or colour; for example, the following pattern also includes two attributes (shape and colour) and a three-element pattern (blue rectangle, red triangle, yellow oval). However, its structure is more complex, because there are three colours instead of two and three shapes instead of two. The pattern core is ABC.

A sequence of a non-numeric repeating pattern with forms: one blue rectangle, one red triangle, and one yellow circle., repeated 3 times.

Note: At the beginning of the math period, introduce patterns that have cores already explored so that students can locate them more easily and effectively.

  • Step 4: Change the mode of representation.

Present patterns that have the same structure but are constructed with different representations, and then test whether students recognize that they have the same structure. For example, present two patterns composed of different concrete materials, such as beads and interlocking cubes, or, as in the pictures below, two patterns, one of which is a concrete representation (a pattern of positions) and the other a visual or semi-concrete representation (a pattern of drawn objects). When students can justify that the two patterns have the same structure, they are at a higher level of abstraction in their algebraic reasoning.

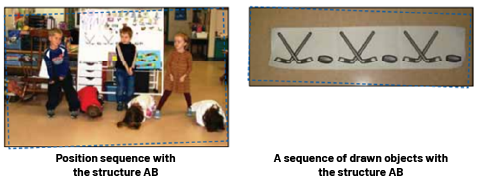
Six children make a repeating pattern with their body. They alternate between a standing and kneeling position on the floor, repeated 3 times. ball position. ‘’The legend illustrates ‘’ pattern with a position of ‘’A’’ ‘’B’’. A drawing of a series of objects, 2 crossed hockey sticks and a puck, repeated 3 times. The legend illustrates ‘’pattern of objects drawn with a structure ‘’A’’ ‘’B’’.
  • Step 5: Identify false leads.

It is important for students to recognize which attribute(s) a pattern is based on.

Examples

Pattern A:

A non-numeric repeating pattern with two elements: triangle and circle, repeated 3 times.

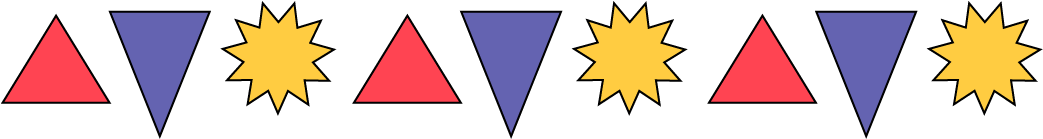
In this pattern, the colours used are not an attribute of the pattern. Therefore, students should ignore the colour attribute and stick to the shape attribute (triangle or circle).

Pattern B:

A non-numeric repeating pattern with a purple pentagon, a red rectangle positioned vertical, a yellow rectangle position horizontal, one red square position vertical, a green trapezoid position horizontal, and a blue square position vertical.

In this pattern, the different shapes and colours create false leads that must be ignored in order to discover the attribute, that is the position of the base (shape placed on a flat side or on a vertex).

Observing similarities and differences between certain patterns reinforces students' learning and develops their communication skills. 

During mathematical exchanges, teachers should engage students in comparing the special characteristics of patterns such as:

  • the attributes;
  • the pattern core;
  • the structure of each pattern..

Example

Pattern 1

Pattern 2
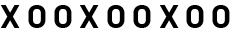A non-numeric repeating pattern with forms: one yellow circle and two blue trapezoids. The pattern is repeated 3 times. A repeating pattern, ‘’X’’, ‘’O’’, and ‘’O’’, repeated 3 times.

Attributes: Shape and Colour

Attribute: Symbols

Pattern core: one yellow circle and two blue trapezoids

Pattern core: one x symbol and two o symbols

Structure: ABB

Structure: ABB

Understanding the pattern core is essential to being able to extend and create patterns and, subsequently, to create new ones.

To get students to create a mental image of a pattern, teachers can also have students compare patterns using examples and non-examples of patterns. This strategy allows students to recognize a pattern, identify the pattern core, describe it and justify their reasoning while using appropriate mathematical vocabulary.

Example of a Pattern with a Repeating Pattern Core

A non-numeric repeating pattern with forms: one yellow circle and two blue trapezoids. The pattern is repeated 3 times.

Non-Example of a Pattern - No Pattern Core

A non-numeric repeating pattern with forms: one yellow circle, one blue trapezoid, one yellow circle, 3 blue trapezoids, one yellow circle, and two blue trapezoids.

Source: translated from Guide d’enseignement efficace des mathématiques de la maternelle à la 3e année, Modélisation et algèbre, Fascicule 1, Régularités et relations, p. 30-34.

Patterns with a Repeating Operation

A repeating operation in a pattern can involve addition, subtraction, multiplication or division.

Examples

  1. An example of an increasing sequence: Position one, one element, of one green trapezoid, position 2, shows 2 elements, 2 green trapezoids, and position 3, shows 3 elements, 3 green trapezoids.
  2. A sequence of a repetitive pattern with cubes: Position one has two cubes, position two have 3 cubes, and position 3 has 4 cubes.
  3. An example of an increasing sequence: Position one, 3 cubes. Position two, 6 yellow cubes. Position 3, 9 yellow cubes.

Source: translated from Guide d’enseignement efficace des mathématiques de la maternelle à la 3e année, Modélisation et algèbre, Fascicule 1, Régularités et relations, p. 40-41.

Skill: Describing Elements and Operations that are Repeated in Various Patterns

Repeating Elements

To help students establish an intuitive understanding of the structure of the pattern, teachers encourage them to verbalize the elements of the repeating pattern. For example, to describe the pattern of movements snap, snap, raise your arm in front of you… , they ask them to say aloud, "snap, snap, raise…"; to describe a pattern of beads forming a necklace, they ask them to touch the beads while naming them aloud: "blue bead, purple bead, red bead…" When students describe their string, it is important that they explain the relationship between the patterns (the elements of the pattern are represented in the same order) and use appropriate math vocabulary.

Sample Questions

  • What attributes are needed to pay attention to in this pattern?
  • What are the elements that make this a pattern?
  • What pattern(s) do you notice?

As an example, they may describe the following pattern as follows: "The attributes are shape and position. The pattern has three elements: a triangle pointing up, followed by a triangle pointing down, followed by a sun. The three elements of the pattern always repeat in this order."

A repeating patterns of 3 elements. A triangle pointing towards the top, triangle pointing towards the bottom, and sun, repeated 3 times.

Students can also record their understanding. Examples of information to record include:

Image A pattern of a repetitive patterns of 3 elements. The pattern has a red triangle, purple triangle pointing downwards, and a sun, repeated 3 times. The 9 elements are numbered from one to 9. The elements are also represented by letters, ‘’A’’, ‘’B’’, ‘’C’’ the letters represent a structure of the pattern, repeated 3 times.A tracing waves line is placed under each motif. The pattern rule: a triangle pointing towards the top, triangle pointing towards the bottom, and a sun is represented always in the same order.

Source: translated from Guide d’enseignement efficace des mathématiques de la maternelle à la 3e année, Modélisation et algèbre, Fascicule 1, Régularités et relations, p. 37-38.

Repeating Operation

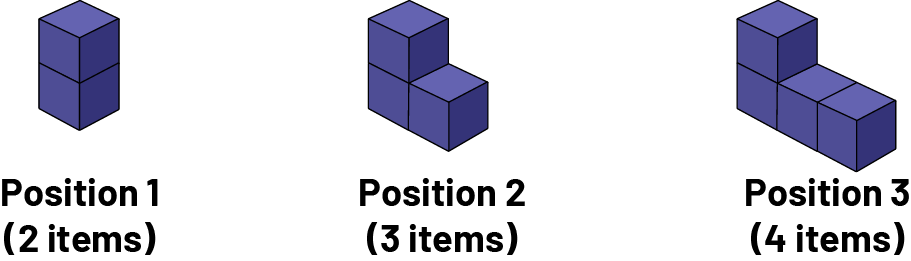
When a pattern has the number of shapes increasing or decreasing consistently from one position to the next, students can describe that change as a repeat operation. For example, students might describe the repeat operation below as follows:

Pattern A:

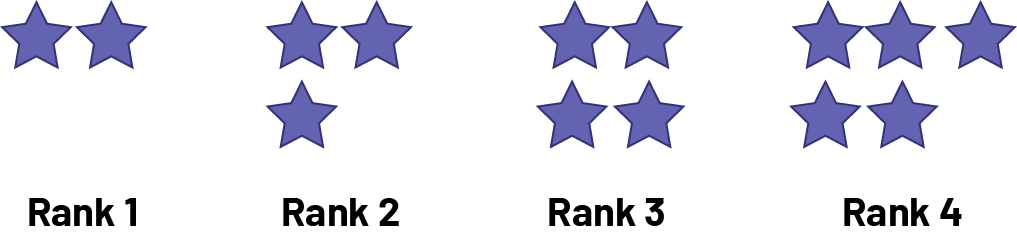
Non numeric increasing patterns. Position one: two stars.Position two: 3 stars.Position 3: 4 stars.Position 4: 5stars.

"This pattern rule consists of adding one star to the shape in the previous position."

Pattern B:

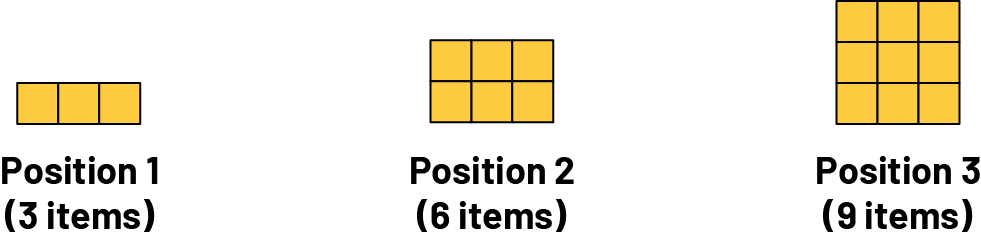
Position one: 3 yellow cubes.Position two: 6 yellow cubes.Position 3: 9 yellow cubes.

"In this pattern three squares are added below the squares in the previous position."

Note: The pattern is described according to the number of elements and not according to the attributes.

The arrangement of the elements for each position can help students see the operation that is being repeated. To help them, ask questions such as:

  • What will the elements in the 3rd position in Pattern A look like? In Pattern B?
  • How can you use the construction of elements from the 3rd position to help you with constructing the 4th position of Pattern B? 5th position?
  • Does this pattern have repeating elements or a repeating operation?

Vocabulary Related to Patterns

Patterns with Repeating Elements

Patterns with Repeating Operations

Pattern A

Non numeric repetitive patterns: Sequence A: position one to 9, rectangle, trapezoid, triangle, repeated 3 times.

Pattern B

Non numeric sequence with increasing patterns. Sequence ‘’B’’. Position one: one square.Position two: two squares.Position 3: 3 squares.

Pattern C

Sequence ‘’C’’Position one: two cubes.Position two: 3 cubes.Position 3: 4 cubes.

Attribute: a characteristic that describes an object that we observe or manipulate. In Pattern A, the attributes that describe the pattern are shape and colour.

When the repeat operation in a pattern is being considered, the attribute is no longer important.

Position Number and Elements: each element whether it is a shape, object, or motion is assigned a position number based on its order. In Pattern A, the blue rectangle is in position 1, the green trapezoid is in position 2, the orange triangle is in position 3, etc.

In Pattern B, one square is in Position 1 and in Pattern C, two cubes are in Position 1.

Pattern core: the smallest part of a pattern which identifies the elements that repeat.

The amount of elements from one position change based on the type of repeat operation, whether it is addition, subtraction, multiplication or division. 

In Pattern A: a blue rectangle followed by a green trapezoid and then an orange triangle.

In Pattern B: a square is added to the previous shape.
In Pattern C: a cube is always added to the bottom term of the shape in the previous position

Structure: letters representing the elements in the pattern rule of a repeating pattern core.

In Pattern A, each element of the pattern can be identified by a letter: blue rectangle (A), green trapezoid (B), orange triangle (C). The structure of Pattern A is therefore ABC.

Position: Where each term is located in a pattern, indicated by a number. It is used to help describe the functional relationships in a pattern and to predict the next terms in the pattern without having to extend it.

In Pattern A, there is a blue rectangle in the 1st, 4th and 7th positions, a green trapezoid in the 2nd, 5th and 8th position, etc.

In patterns B and C, each shape has its position: the first shape is in the 1st position, the second shape is in the 2nd position, etc.

Source: translated from Guide d’enseignement efficace des mathématiques de la maternelle à la 3e année, Modélisation et algèbre, Fascicule 1, Régularités et relations, p. 29.

Skill: Recognizing and Describing Patterns Found in Everyday Life

Young children have a natural curiosity and interest in the patterns around them. Patterns can be literary, artistic, musical, scientific or numeric. Patterns are found in many events, such as the succession of seasons and days of the week, the growth of living things, and daily activities; in rhymes and songs, such as rhythm, rhymes, and number of syllables; in stories with repeated structures, such as Goldilocks and the Three Bears; in music, such as the different sounds of instruments; in gestures, such as standing, crouching, or sitting; and in the world we have built, such as traffic lights, odd and even house numbers. Repeating patterns used for decorating objects, such as vases, clothing, and jewelry, from various cultures are also excellent examples of repeating patterns

Source: translated from Guide d’enseignement efficace des mathématiques de la maternelle à la 3e année, Modélisation et algèbre, Fascicule 1, Régularités et relations, p. 26.