C1.3 Determine pattern rules and use them to extend patterns, make and justify predictions, and identify missing elements in patterns that have repeating elements, movements, or operations.
Activity 1: Steamboat (Extend, Predict and Justify)
Ask students to build a steamboat using a red trapezoid and an orange square. Have them add a blue diamond to represent the steam.
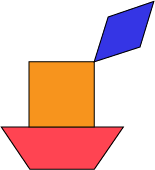
Present the following problem:
How many pattern blocks are needed to build 10 steamboats?
When solving this problem, students discover the relationship between the number of pattern blocks that are needed to build them. Have students solve this problem by creating the steamboats and filing in a table of values. After the first three steamboats are recorded, ask students if they see a pattern rule that they can use to determine the number of pattern blocks for 10 steamboats.
Example of a Table of Values
| Number of boats | 1 | 2 | 3 | … |
|---|---|---|---|---|
| Pattern blocks | 3 | 6 | 9 | … |
Source: translated from Guide d’enseignement efficace des mathématiques de la maternelle à la 3e année, Modélisation et algèbre, Fascicule 1, Régularités et relations, p. 48.
Activity 2: The Pattern in My Name
Give students a 5-by-5 grid. Ask them to write their first name as in the grid below and to colour the first letter of their first name each time it appears. Have them discover that some names form a pattern in a 5-by-5 grid and others do not.

Ask questions to bring out the relationship between the dimensions of the grid and the number of letters composing a given name. For example:
- What will happen if you colour the first letter of your name throughout the grid?
- What will happen if you write your first and then your last name? only your last name?
- Is there a pattern rule? What is it?
- Are the pattern rules the same?
For example, if you write the six letters of the name Sandra in the 5 by 5 grid, you can see patterns for the arrangement of each letter in the grid. Each letter is arranged on a diagonal line (for example, the letter S). If we were to write a 4-letter name like Mary in a grid of the same dimensions, we would also observe a pattern vertically. On the other hand, if we write a seven letter name like Martine in a 5 by 5 grid, each letter would form a line less clear than the vertical line. It is important to note that the dimensions of the grid as well as the number of letters in the name influence the pattern formed by the repetition of the name.
Source: translated from Guide d’enseignement efficace des mathématiques de la maternelle à la 3e année, Modélisation et algèbre, Fascicule 1, Régularités et relations, p. 47.
