GEEM - Teaching and Learning

D1.1 Sort sets of data about people or things according to two attributes, using tables and logic diagrams, including Venn and Carroll diagrams.

Activity 1: Classify

Teachers can ask students to classify the same attribute blocks according to a combination of the two attributes using the linking word and.

sort attribute blocks according to an attribute (for example, colour)
Image Two containers of logic blocks, one for Reds and one for Non-reds: the first contains a redcircle, a red square, a red rectangle, a red triangle and a red hexagon, and the second contains a blue circle,two blue rectangles, a yellow triangle and a yellow square.
classify the same attribute blocks according to a new attribute (for example, thickness).
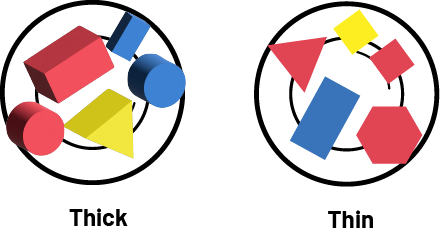Image Two containers of logic blocks, one for Thick and the other for Thin: the first one containsa red and thick circle, a blue and thick circle, a red and thick rectangle, a blue and thick rectangle, and ayellow and thick triangle, and the second one contains a red and thin triangle, a yellow and thin square, a redand thin square, a blue and thin rectangle, and a red and thin hexagon.
classify the same attribute blocks according to a combination of the two attributes using the linking word and.
Image Two containers of logic blocks, one for Red and Thick and the other for Non-Red and Thick:the first contains a Red and Thick circle, and a Red and Thick rectangle, and the second contains a Blue and Thickcircle, a Blue and Thick rectangle, and a Yellow and Thick triangle. 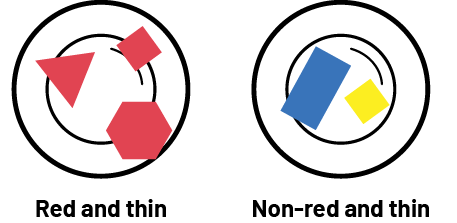Image Two containers of logic blocks, one for Red and thin and the other for Non-red and thin: thefirst contains a red and thin square, a red and thin triangle, and a red and thin hexagon, and the second containsa blue and thin rectangle, and a yellow and thin square.

Source: translated from Guide d’enseignement efficace des mathématiques, de la maternelle à la 3^e année, Traitement des données et probabilité, p. 37.

Activity 2: Attributes

Students need to understand that every object has many attributes. To facilitate this understanding, teachers should provide students with a variety of activities in which they seek to describe objects with an emphasis on observing and analyzing the similarities and differences between objects. They can then note the proposed characteristics and model a way to organize this information in a table. An example of a table is shown below:

Attributes	Characteristics
material	plastic, metal
size	small, big
shape	round, square

Source: translated from Guide d’enseignement efficace des mathématiques, de la maternelle à la 3^e année, Traitement des données et probabilité, p. 38.

Look-Fors During an Attribute Search Activity

Observe if students:

explain the differences between the objects;
note the details;
compare the characteristics;
create sets;
examine objects that are not part of a class to determine the characteristics of the set;
define the attribute;
consider more than one attribute at a time;
sort and classify the same objects according to several attributes.

Source: translated from Guide d’enseignement efficace des mathématiques, de la maternelle à la 3^e année, Traitement des données et probabilité, p. 42.

To encourage them to classify the same objects by another attribute or by two attributes using the conjunction and (for example, size and colour), ask questions such as:

Can we group these objects in another way?
If I remove these objects, how could we classify the remaining ones? Why?
If these two objects were placed in the same category, what would we name this new set?

Source: translated from Guide d’enseignement efficace des mathématiques, de la maternelle à la 3^e année, Traitement des données et probabilité, p. 45.

Activity 3: Classify Using a Venn Diagram

Teachers can introduce students to classification using a Venn diagram, for example, by asking them to indicate whether they like:

pasta;
vegetables;
pasta and vegetables;
neither pasta nor vegetables.

Teachers place two hoops as in the picture below and add a label under each (vegetables, pasta) and ask students to represent their response by placing their name (or picture) in the appropriate place inside or outside the hoops.

Thus, from this representation, we see that:

eight students like pasta;
six students like vegetables;
two students like pasta and vegetables (Zahir and Ines);
one student does not like pasta nor vegetables (Pascal).

Source: translated from Guide d’enseignement efficace des mathématiques, de la maternelle à la 3^e année, Traitement des données et probabilité, p. 47-48.

To help students understand the concept of intersection of two sets, teachers can:

prepare a number of objects to be classified according to two different characteristics;
make sure that some of the objects have both characteristics and others do not;
draw a rectangle with wool or lay a tablecloth or tarp on the floor to represent the reference set;
place all the objects to be classified in a corner of the rectangle;
place two different coloured hoops side by side and identify them according to the chosen characteristics;
have students place objects in the appropriate hoops until they come across an object that can be placed in both;
then ask questions such as: 'Where do we place the object? Do you have any suggestions?';
use students' responses to prompt them to arrange the hoops so that one overlaps a section of the other, as in the previous diagram;
place the object in the region corresponding to the intersection of the two hoops and ask students to describe the objects that should be placed in that region;
continue the activity until all the objects have been classified and ask students to justify their classification;
ask students to explain why certain objects must be placed outside the hoops.

Source: translated from Guide d’enseignement efficace des mathématiques, de la maternelle à la 3^e année, Traitement des données et probabilité, p. 51.