D2.1 Use mathematical language, including the terms “impossible”, “possible”, and “certain”, to describe the likelihood of complementary events happening, and use that likelihood to make predictions and informed decisions.
Activity 1: Complementary Events
Present the image below to the students.
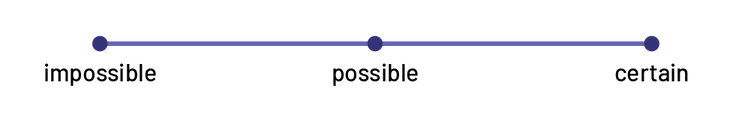
Ask students the probability of eating a carrot and the probability of eating a tomato. Have them demonstrate the probability of these two events occurring on the probability line below, and discuss the fact that these two events are complementary (if it is certain to eat a tomato, then it is necessarily impossible to eat a carrot).
Activity 2: Memory Game (Making and Verifying Predictions)
Materials
Appendix 2.5 (one copy per pair)
Summary
Students describe, using appropriate vocabulary, the probability of turning over two identical cards in a memory game.
Directions
Form teams of two and give each team a copy of Appendix 2.5. Present the cards. Point out that the giant panda, harp seal, elephant and monkey are on two cards, while the American bison is on one card. Have students cut out the cards, shuffle them, and place them face down in rows on the table. Explain that the game is to try to turn over two identical cards at the same time with as few attempts as possible.
Ask questions such as:
- Is it certain that you will be able to turn over two identical cards on the first try?
- What are the possibilities of turning over two identical cards if you turn over the card with the American bison on it?
- Can you turn over the two cards with the elephant on the first try?
- How many tries do you think it will take to find all the identical cards?
Have students take turns performing the experiment, making sure to record the number of tries required to find the four identical pairs of cards.
Ask questions such as:
- Is it easier to find the second pair than the first? Why?
- Why is this game called a “memory game”?
Source: translated from Guide d’enseignement efficace des mathématiques, de la maternelle à la 3e année, Traitement des données et probabilité, p. 228.
Activity 3: Use of the Terms Impossible, Possible and Certain (Probability Line)
The teacher posts the labels impossible, possible, and certain on the board. The teacher presents students with a series of index cards with a different event written on them, for example, 'My grandmother is on the moon,' 'A dog eats an apple.' The teacher asks each student to choose an event and to describe the likelihood of this event happening. To represent this description, the student must stand in front of the corresponding label.
In photo 1, we see that the third student from the left understands the idea that the word possible represents the continuum from impossible to certain, and therefore can stand closer to one end of that continuum than the other.
Image In a classroom, six students stand under one of three labels, Impossible, Possible and Certain.
Each student holds a poster in front of their face with an impossible, possible or certain statement. Label
Impossible: It snows in July. My grandmother is on the moon. I will get a dog for my birthday. Label Possible: A dog
is eating an apple. Label Certain: My mother makes dinner every day. My friend plays tennis.
Photo 1
The probability line model is an effective visual way to represent this continuum.
Note: As illustrated in Photo 2, it may be helpful to begin by asking students to classify an event based on only two characteristics, such as impossible and certain.
Image Two groups of students sitting one behind the other, in two columns, according to two
statements: 'It is impossible that I will play sports after school' and 'It is possible that I will play sports after
school”.
Photo 2
Source: translated from Guide d’enseignement efficace des mathématiques, de la maternelle à la 3e année, Traitement des données et probabilité, p. 55-56.
To further develop the concept of complementary events, take the opportunity to present cards with complementary events on them (for example, the probability of being in Grade 2 now and being in Grade 3 now, the probability of being in the current season and the probability of being in another season).