D2.1 Use mathematical language, including the terms “impossible”, “unlikely”, “equally likely”, “likely”, and “certain”, to describe the likelihood of events happening, and use that likelihood to make predictions and informed decisions.
Skill: Using Mathematical Vocabulary to Express the Probability of an Event Occurring
In the primary grades, students develop probabilistic thinking on a daily basis through the learning of certain words or phrases that describe the frequency or likelihood of certain events, for example:
- We always have Saturday and Sunday off from school.
- It's possible I'll play ball at recess.
- It's unlikely to rain today.
Students also develop an understanding of the concepts of variability and chance by conducting simple probability experiments. Although their understanding of theoretical probability is still in its beginning stages, they can recognize, for example, that by spinning the arrow on a spinner that is divided into thirds, there is a 1 in 3 chance that the arrow will land on the third that is red.
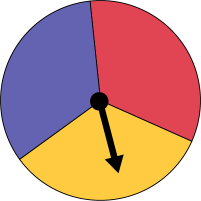
Source: translated from Guide d’enseignement efficace des mathématiques, de la 4e à la 6e année, Traitement des données et probabilité, p. 128.
In Kindergarten, one of the first manifestations of the meaning of variability occurs when pupils are able to indicate whether it is certain, possible or impossible that a given event will occur (for example, It is impossible that cows start to fly). Some young students find it very difficult to do this. For many, what is possible becomes certain. For example, if the teacher tells them that it is possible that they will bring their dog to class the next day, the students will be very disappointed if this does not happen, since in their minds, it is certain that the teacher will bring it. They must understand that if an event is possible, it is equally likely to occur or not occur. Other students have difficulty distinguishing between what has never happened and what may never happen. For them, if an event has never happened, it is impossible. For example, they may think it's impossible for them to go a whole night without sleep because they've never done it. However, such an event is not impossible.
Source: translated from Guide d’enseignement efficace des mathématiques, de la 4e à la 6e année, Traitement des données et probabilité, p. 132-133.
Probability can be represented on a continuum from impossible to certain, each term qualifying the degree of probability: impossible, unlikely, equally likely, very likely and certain.
Skill: Making Predictions and Informed Decisions
In the Data strand, the inquiry process is the preferred problem-solving approach. This approach fosters critical probabilistic thinking by encouraging students to formulate conclusions from data collected in probability experiments and to question their intuition about the probability of the outcomes. Such an approach helps to avoid the development of some of the misconceptions about probability that are too often found in both students and adults.
Source: translated from Guide d’enseignement efficace des mathématiques, de la 4e à la 6e année, Traitement des données et probabilité, p. 128.
Knowledge of concepts related to probability helps students better understand all kinds of everyday situations, such as understanding weather forecasts, the possible outcomes of an experiment, or the probability of winning a raffle. The big idea of Probability emphasizes the importance of probabilistic thinking in informing decision making in situations where the outcome is uncertain due to the fact that it is related to chance.
Source: translated from Guide d’enseignement efficace des mathématiques, de la 4e à la 6e année, Traitement des données et probabilité, p. 127.
Concept of Probability
Probability is used to describe the degree of certainty with which a certain outcome or event can be predicted to occur in a situation of variability. Developing an understanding of the concept of probability is a long process that begins in the early grades. However, it is very important to build on an intuitive understanding of the concept first. In the primary grades, students are able to intuitively recognize that in a given situation, certain events are possible and that the degree of certainty that they will occur is on a continuum from impossible to certain. The probability line model is an effective visual means of describing this continuum.
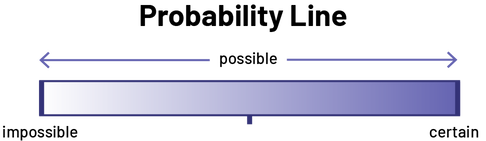Image Under the title "Probability Line", the word "possible" appears between two arrows pointing to the extremes of the progressive bar below. The bar starts with white and gradually turns blue. On the far left is the word "impossible", and on the far right is the word "certain".
Students place a point on this continuum to describe how certain they are that a particular event will occur. The further to the right of the line, the greater their degree of certainty. If they place it in the middle, it means that they believe the event is as likely to occur as not.
By the end of the primary grades, students begin to replace the word possible with the word likely. They can then label an event as unlikely or very likely (for example, It is unlikely to rain today) and represent it as a point on a probability line. In the junior grades, students continue to develop their understanding of the concept of probability by using the terms impossible, unlikely, equally likely, very likely, and certain to place events on a probability line. At this stage of the development of the concept of probability, there is still no question of quantifying probability precisely, so events are placed approximately on the line.
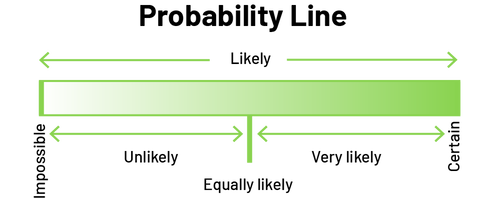Image Under the title "Probability Line", the word "likely" appears between two arrows pointing to the extremes of the progressive bar below. The bar starts with white and gradually turns green. On the far left is the word "impossible", on the far right is the word "certain", and in the middle is the word "equiprobable". Between "impossible" and "equiprobable", which are connected by a two-way arrow, we find the expression "somewhat likely". Between "equiprobable" and "certain", which are connected by a two-way arrow, we find the expression "very likely".
Students also begin to pay more attention to the possible outcomes of a given situation. Teachers should present them with simple situations that use concrete materials and that encourage them to list these outcomes and compare their probability.
Example
Teachers present students with the three spinners below and ask them to compare the probability of some of the outcomes.
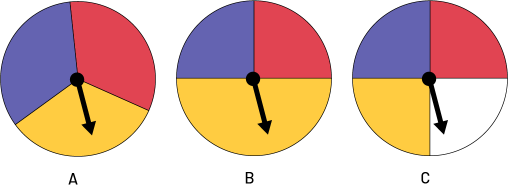Image Three wheels with a needle are placed side by side. Roller A is separated into three equal parts of different colors: one purple, one red and one yellow. The needle points in the yellow part. Wheel B is separated into three parts: the yellow part takes half, while the red and purple parts each take a quarter. The needle points in the yellow part. Wheel C is separated into 4 equal parts: red, purple, yellow and white. The needle points in the white part.
Here are some possible answers:
- Using Spinner B, it is more likely to obtain the result yellow than to obtain the result blue or the result red.
- Using Spinner A, the blue, red and yellow results are equally likely.
- Using Spinner C, the four outcomes are also equally likely.
- It is more likely to get the red result with Spinner A than with Spinner C.
Teachers can use this scenario to encourage students to think about the concept of equally likely outcomes, which is fundamental to the development of an understanding of theoretical probability. They can point out that spinners A and B each have three possible outcomes (red, blue and yellow) and ask them to explain why the outcomes of spinner A are equally likely and those of spinner B are not. This type of questioning allows teachers to support students in understanding the importance of always challenging their intuition and thinking things through when analyzing a situation.
Teachers can then ask students to perform a small probability experiment in teams, spinning each spinner a number of times (for example, 100 times) and recording the results. The teacher should then ask whether the results support the previous ones. Teachers use the variability of the results obtained by the different teams to re-emphasize the fact that in any probability situation, the results are random and cannot be determined with certainty.
Probability experiments are used to support or challenge intuition and are central to the development of probabilistic thinking.
Source: translated from Guide d’enseignement efficace des mathématiques, de la 4e à la 6e année, Traitement des données et probabilité, p. 134-136.
As students gain experience with situations involving variability, some tend to develop misconceptions. One of the most common misconceptions relates to the imposition of implicit limits on variability. For example, in a game of heads or tails, students get tails five times in a row. If they are then asked to predict the result of the next toss, many will tend to indicate that it will certainly correspond to the heads side because their intuition leads them to believe that there is a limit to chance favouring the tails side. Teachers should help them understand that chance has no memory, that is, each result is independent of previous results and cannot be predicted with certainty. It is therefore just as likely to get tails as to get heads on the next roll. This kind of misunderstanding persists among many adults who believe, for example, that certain numbers have a better chance of being part of the winning lottery numbers simply because they have come up more often in the past. A good understanding of the concept of variability makes it possible to recognize that this is not the case. To help students fully grasp this concept, teachers should introduce them to many simple probability experiments and encourage them to question themselves and remain objective with regard to their intuition.
Source: translated from Guide d’enseignement efficace des mathématiques, de la 4e à la 6e année, Traitement des données et probabilité, p. 133-134.