D1.2 Collect data, using appropriate sampling techniques as needed, to answer questions of interest about a population, and organize the data in relative-frequency tables.
Skill: Collecting Data
Planning and conducting data collection provides meaningful data.
The inquiry process is a comprehensive one that involves four steps: identifying the situation, collecting the data, organizing the data, and interpreting the results.
Once students have clarified the problem and formulated one or more questions of interest, they should plan and carry out a data collection. It is important to consider the various types of inquiries, the different kinds of data, and the differences between population and sample during data collection planning. By actively involving students in data collection planning, teachers encourage them to make thoughtful choices and look critically at the entire inquiry process.
Source: translated from Guide d’enseignement efficace des mathématiques, de la 4e à la 6e année, Traitement des données et probabilité, p. 44.
Questions to Ask
It is important to provide students with a variety of opportunities to plan a data collection. Teachers will question students throughout this stage to help them understand the importance of choosing the right type of inquiry and data for the question of interest, as well as the importance of identifying the population and, if necessary, the sample. This will encourage students to develop the critical thinking skills that will be most useful in the fourth stage of the inquiry process.
Here are some suggested questions teachers can ask students to guide them through the data collection planning process:
Type of Inquiry:
- What type of inquiry is most appropriate for your question of interest? Why?
Type of Data:
- What kind of data will you collect?
- Does this kind of data lend itself well to your question of interest? Why or why not?
- If you are going to use secondary data, where will it come from? Is this source reliable?
The Target Population:
- What is your target population?
- Is this the group that is the focus of your inquiry?
- Will your inquiry be conducted with the entire population or only a portion of the population?
Sample Size:
- What will your sample size be and how will you determine it?
- Using a sample of this size, will the results be representative of the target population? Why?
- Do you think the results would be similar if the sample size were smaller, larger, and why?
Sample Composition:
- Is the sample composition free of bias? Convince me.
- How will you randomly select your sample?
- Does your sample need to be stratified? Why or why not?
- What strata do you plan to use in your sample composition and what will be the size of each stratum?
The Modalities (Where, When, How):
- Where will you conduct your inquiry?
- When are you going to conduct your inquiry? Why is this a good time? If the inquiry were conducted at another time, would the results be the same?
- How will you go about getting the data you need?
- How will you record the results of your inquiry?
Source: translated from Guide d’enseignement efficace des mathématiques, de la 4e à la 6e année, Traitement des données et probabilité, p. 57-58.
Skill: Organizing Data
Once students have identified the situation and collected data, they need to organize the data.
Why Organize the Data
Gal (2002) indicates that data are organized to better analyze or communicate information. Since the purpose of the inquiry is to find an answer to one or more questions of interest, it is very difficult to base that answer on data that are presented in a disorganized fashion. By organizing the data collected, they can be presented in a way that summarizes them, highlights some of the information they contain, communicates their main characteristics, and facilitates their interpretation.
Source: translated from Guide d’enseignement efficace des mathématiques, de la 4e à la 6e année, Traitement des données et probabilité, p. 62.
Knowledge: Types of Inquiry
Data Collection by Observation
In data collection by observation, one records what one sees or does.
Examples
- Count the number of birds seen in the schoolyard at specific times.
- We count the number of cars passing through an intersection during a given time interval.
- We count the number of times we go to the sports center in a month.
- Every day for a week, we note the time at which we go to bed and the time at which we get up.
In planning to collect data using observations, it is necessary to plan where, when, what, and sometimes how to observe (for example, how to distinguish between a car making an incomplete stop at an intersection and a car making no stop). It is also possible to plan whether all observations will be made by one person or whether they will be made by several people at the same time to ensure better reliability.
Data Collection by Means of Taking Measurements
When collecting data by taking measurements, measurements are taken in situations that involve a limited number of variables, as is the case in an experiment.
Examples
- We measure the time taken by Grade 4 students to read a given text.
- We measure the amount of rain, in millimeters, that falls each day in May.
When planning to collect data by measurement, it is important to consider where, when and how to collect the measurements, and whether all measurements will be collected by one person or by several people at the same time to ensure greater reliability.
Data Collection by Means of an Experiment
When collecting data by experiment, the data are derived from a manipulative, scientific activity that requires adherence to certain preset parameters and, often, the use of precise measurement techniques and tools.
Examples
- At specific intervals, plants are measured for growth, with some receiving a small amount of nutrients, some receiving a larger amount, and some receiving none, to test if nutrients are contributing significantly to plant growth.
- Every 30 seconds, we take the temperature of any liquid that has been heated to 100°C and left to cool down. We repeat the experiment with different liquids in order to compare the speed at which they cool down.
When planning an experiment, the scientific approach must be used and the reliability of the data collection method must be ensured. Variables that may render the results invalid must be controlled for and neutralized.
Survey
When conducting a survey, data are collected by asking a number of individuals about a particular topic. The questions often take the form of a questionnaire that can be answered in writing or orally.
Examples
- Students in the class are asked how many hours they spend watching television each week.
- Grade 6 students are asked to name their favourite genre of music.
In planning a survey, it is important to write the survey questions well to ensure that they are clear and objective. It is also important to anticipate the responses that may be given and sometimes to group them into categories.
Secondary Data Collection
During secondary data collection, the data is usually found in an electronic database such as a website, or in a printed document such as a book, magazine, or encyclopedia.
Examples
- We want to compare the population of Canadian provinces and territories.
- We want to compare the subject preferences of elementary school students to middle school students across Canada.
When planning an inquiry using secondary data, it is important to check whether the data are available, where and how to obtain them, and to ensure that the source is reliable.
Source: translated from Guide d’enseignement efficace des mathématiques, de la 4e à la 6e année, Traitement des données et probabilité, p. 46-48.
Knowledge: Question of Interest
A question of interest is a self-selected question for which data should be collected. The question may not only deal with preference, but also with size, quantity or general information.
Source : The Ontario Curriculum. Mathematics, Grades 1-8 Ontario Ministry of Education, 2020.
Knowledge: Primary Source
Primary-source data are data collected by the person conducting the inquiry. They are well suited for questions of interest about objects and people in the students' immediate environment. They are ideal for introducing students to data management, since students are usually more interested in the data they have collected.
When students know the range of possible responses, they can facilitate the recording of data using a frequency table. For example, in a survey situation where a population is asked to identify the preferred type of soup, students can record a tally in the row corresponding to each response in a table such as the one below. This counting strategy is called tallying. Every fifth tally is drawn at an angle to the previous four, making it easier to count the results later. The Frequency column shows the total number of tallies in every row.
Favourite Soups
| Type | Count | Frequency |
|---|---|---|
| Chicken Noodle Soup |  |
7 |
| Broccoli Soup |  |
4 |
| Cream of Mushroom soup | 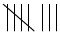 |
8 |
| Vegetable Soup | 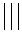 |
3 |
| Tomato Soup | 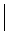 |
1 |
Source: translated from Guide d’enseignement efficace des mathématiques, de la 4e à la 6e année, Traitement des données et probabilité, p. 50-51.
Knowledge: Secondary Source
Secondary data are data collected by an individual or organisation (such as a researcher, a company, or an association) other than the person conducting the inquiry. These data can be found in books, encyclopedias, magazines, newspapers, and/or on the Internet. They are particularly useful for answering questions of interest for which primary data are difficult or impossible to collect; for example, what was the size of the Francophone population in Canada's major cities in recent years? They can also be used to interpret other data to which they are related. Since students are exposed to secondary data on a daily basis, teachers should help students develop the ability to judge its reliability, validity, and relevance. In order to do so, teachers must continually raise student awareness of the importance of verifying the reliability, validity, and relevance of the data they encounter.
Inquiry Process and the Internet
Access to the Web gives students the opportunity to participate in national and even international projects that place them in authentic data collection and sharing situations, while fostering collaboration among students from different countries. The Census at School project, for example, "[…] is an international online project that introduces students in grades 4 to 12 to the world of surveys and statistics. The project originated in the United Kingdom in 2000, and schools in Australia, Canada, New Zealand and South Africa are now participating. Young people from these countries anonymously complete a questionnaire in class. They provide non-confidential information such as their height, travel time to and from school, and their favourite subject. The responses are entered into a national database, which is then added to an international database maintained in the United Kingdom" (Statistics Canada, Census at School Canada. Accessed June 23, 2022).
Since students need to recognize the difference between primary and secondary data, starting with such projects is an interesting way to investigate a topic about which they care and are passionate.
Source: translated from Guide d’enseignement efficace des mathématiques, de la 4e à la 6e année, Traitement des données et probabilité, p. 51-52.
Knowledge: Population
In statistics, the set of objects, events or persons that one wishes to study is called the population. When planning data collection, the target population for the inquiry must be defined. The choice of the population is partly dictated by the inquiry's intent and the statement of the question of interest.
Examples of Populations in Statistics
- The people of Canada
- Baseball fans
- Grade 4 students from the school
- Primary school students
- Parents of middle school students
Source: translated from Guide d’enseignement efficace des mathématiques, de la 4e à la 6e année, Traitement des données et probabilité, p. 52.
Knowledge: Relative-Frequency Table
A relative-frequency table shows the fraction, decimal number, or percentage of data values for each category of data. The sum of the relative frequencies is 1 or 100%.
Example
The number of responses for each category is expressed as a proportion of the total number of responses (50) and as a percentage.
Taking Action for the Environment
| Measure | Number of Votes | Relative Frequency |
|---|---|---|
| Creating a butterfly garden | 25 | \(\frac{25}{50}=0.50=50 \%\) |
| Campaign to reuse items | 10 | \(\frac{10}{50}=0.20=20 \%\) |
| Turn off lights and electronics for one hour each school day | 15 | \(\frac{15}{50}=0.30=30 \%\) |
Source: Grade 5 students from School B
Source : The Ontario Curriculum. Mathematics, Grades 1-8 Ontario Ministry of Education, 2020.
