B1.7 Recognize that one half and two fourths of the same whole are equal, in fair-sharing contexts.
Skill: Recognizing Equivalence
To recognize the equality of two fractions is to recognize that both fractions represent the same quantity.
The concept of equivalent fractions is introduced for the first time in Grade 1. It is sometimes difficult for students to grasp that a quantity can have more than one name and be represented by more than one number; for example, one half represents the same quantity as two fourths; therefore, it is safe to say that \(\frac{1}{2}\ = \frac{2}{4}\).
Understanding equivalent fractions is connected to proportional reasoning which students will continue to develop over the next few years.
Determining equivalent fractions means determining fractions that represent the same quantity. We then look for a number of "small parts" of a whole that correspond to a particular number of "large parts" of the same whole; for example, if we are looking for the number of fourths that correspond to a half, we can represent the two fractions using identical area patterns in order to recognize the equivalence:
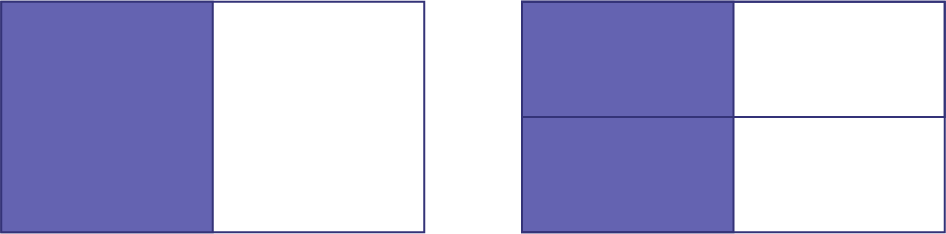
The equivalence relationship can also be explored with respect to fractions of a set. In the picture below, students can easily see that \(\frac{2}{4}\) of the candy is wrapped in purple wrappers. However, they may have difficulty determining the equivalent fraction\(\frac{1}{2}\) . It is important to provide activities where students can manipulate the parts of the set. In this way, students can group them into sets of two and determine the equivalent fraction.

\(\frac{2}{4}\)Candies are wrapped in purple wrappers.
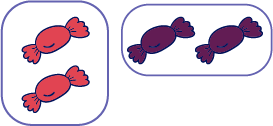
\(\frac{1}{2}\)Candies are wrapped in purple wrappers.
Source: adapted from Guide d’enseignement efficace des mathématiques de la 4e à la 6e année, Numération et sens du nombre, Fascicule 2, Fractions, p. 50-52.
Knowledge: Fractions
Understanding the representation of quantities by fractions helps to deepen the concept of quantity. It is important to understand that fractions, such as one half, one third, or one fourth, represent different quantities depending on whether they refer to a part of a whole (a length, area, or object) or a part of a set; for example, one third of a chocolate bar (part of a whole) represents a quantity of chocolate based on the size of the original bar. However, one third of a dozen eggs (part of a set) represents four eggs.

Source: translated from Guide d’enseignement efficace des mathématiques de la 1re à la 3e année, Numération et sens du nombre, p. 48.
Provide students with opportunities to discover:
- that when the fraction represents a region (area model), the area of each part must be of equivalent size;
- that when fractions are used to describe sets (set model), the objects making up the sets can be different sizes (if the fruit bowl is said to be made up of apples, the other fruit bowl can be made up of grapes that are smaller than the apples, for example);
- that the fraction represents a part of a whole.
Source: translated from Guide d’enseignement efficace des mathématiques de la 1re à la 3e année, Numération et sens du nombre, Document d'appui, p. 30.
Knowledge: Halves
When a whole is divided into two equivalent parts, each part is half of the original quantity. Two halves make a whole.
Note: In Grade 1, students should learn terminology related to the terms half and fourth. Half of a half is a fourth.
Source: translated from En avant, les maths! ML, Nombres, p. 2.
Knowledge: Fourths
When a whole is divided into four equivalent parts, each part is a fourth of the original quantity. Four fourths make a whole.
Note: Using unit fractions improves understanding of quantity. When students count by naming unit fractions, it helps them see the parts of the fraction during the composition and decomposition of fractions. So, they should have experience reading fractions as 1 one-fourth, 2 one-fourths, 3 one-fourths, 4 one-fourths*.
*Fractions Learning Pathways, Unit D - Use unit fractions to name and count fractional amounts.
Connais Source: translated from En avant, les maths! ML, Nombres, p. 2.
Knowledge: Equivalent Fractions
Two fractions are equivalent if they represent the same quantity.
Note: In Grade 1, it is important to emphasize that these are fractions of the same whole. The same part of a whole can be represented by different fractions; for example, one half and two fourths (2 one fourths) are equivalent fractions.
Note: Four-person sharing problems with a remainder of 2 provide an opportunity to show that one half and two fourths are equivalent.
Source: translated from En avant, les maths! ML, Nombres, p. 3.
