B2. Operations
Use knowledge of numbers and operations to solve mathematical problems encountered in everyday life.
Learning Situation 1: Two by Two
Duration: approximately 2 hours
| Overall Expectation | Specific Expectations |
| B2. Operations Use knowledge of numbers and operations to solve mathematical problems encountered in everyday life. |
B2.2 Recall and demonstrate addition facts for numbers up to 20, and related subtraction facts.
B2.3 Use mental math strategies, including estimation, to add and subtract whole numbers that add up to no more than 50, and explain the strategies used. B2.4 Use objects, diagrams, and equations to represent, describe, and solve situations involving addition and subtraction of whole numbers that add up to no more than 100. |
Learning Goals
The purpose of this learning situation is to allow the student to:- establish relationships between numbers;
- estimate the sum of an addition of two two-digit numbers;
- develop a variety of counting strategies to mentally add two-digit whole numbers;
- solve problems related to a number sentence.
| Learning Context | Prerequisites |
|---|---|
| When it comes to basic mathematical operations, students are too often presented with a specific algorithm rather than being given the freedom to do it their way. For example, when adding two-digit numbers, the tradition has been to add the ones column first and then the tens column. When students are allowed to solve the problems in their own way, some students start with the tens column and then move to the ones because it seems easier.
In Grade 2, students should be able to understand the concepts and procedures associated with mathematical operations and apply them in a problem-solving context. They need to grasp the concepts related to grouping and sharing and to discover the patterns and relationships between operations. A student who mechanically applies a procedure to solve the problem 100 - 1 = ? is not using their own reasoning. By allowing them to create their own algorithms, students learn to think, to devise their own strategies, and to develop good number sense. |
In this learning situation, students will:
|
Materials
Main Activity
- manipulatives
- large sheet of paper or interactive whiteboard
- place value mat
- counters (one per student or in pairs)
Mathematical Vocabulary
strategy, approach, solution, algorithm
Before Learning (Warm-Up)
Duration: approximately 40 minutes
Group students into pairs.
Present the following problem to students.
Next Friday is pizza lunch. The last time we had pizza, we ran out. So we need to order a little more. We ordered 48 pieces of pizza. We need 13 more. How many pieces of pizza should we order this time?
Ask a few students to take turns explaining the problem in their own words. Help them express themselves by asking the following questions:
- What do you already know?
- What do you want to know?
Allow students time to reflect on the problem so that the team members can agree on a strategy to solve the problem.
Observe students as they work and note the various strategies used.
Circulate around the classroom and observe the teams. Ask students the following questions:
- How can you use what you already know to solve the problem?
- Why did you choose these manipulatives?
- How did you solve the problem?
- Could you use another strategy to solve the problem?
- Can you demonstrate that your solution is correct?
Provide enough time so that students can try more than one strategy.
Ask students to bring in their manipulatives or worksheets and sit in a circle.
Invite teams, in turn, to explain how they solved the problem.
Encourage students to explain their strategy using the materials they used or the calculations they made.
Encourage other students who used the same materials but a different strategy to explain their own approach.
As you go along, make a list of strategies on a large piece of paper and illustrate the steps for each solution. Display this list of strategies in the classroom.
Examples of counting strategies to solve the following problem:
\(38 + 13 \)
Counting Strategy 1
\(30 + 10 = 40\) (add tens);
\(8 + 2 = 10\) (make a ten);
\(40 + 10 = 50\);
Add the remaining 1 from the 13;
\(50 + 1 = 51\).
Counting Strategy 2
\(38, 39, 40\) (add 2 from the 13 to get 40, which leaves 11);
\(40 + 10 = 50\) (add 10 from the 11);
\(50 + 1 = 51\) (add 1 remaining).
Counting Strategy 3
\(38 + 10 = 48\) (add tens);
\(48 + 3 = 51\) (add on the ones).
Ask students the following questions:
- What other strategies could you have used?
- How would you explain your strategy to someone who was not present today?
Active Learning (Exploration)
Duration: approximately 40 minutes
Present the following problem to students. Ask them to solve it individually.
We need books to research animals. We found 36 books about animals in the classroom library. We also found some in the school library. We have 72 books in total to research animals. How many books did we find in the school library?
Give students time to think about this problem and choose the strategy that works for them.
Encourage students to refer to the list of strategies displayed and to use the ones they have mastered.
Circulate around the classroom and ask students questions to stimulate their thinking; for example:
- What do you already know?
- What do you want to know?
- How can you use what you already know to solve the problem?
- Why did you choose these manipulatives?
- What strategy will you use to solve the problem?
- Can you use another strategy to solve the problem?
Invite students to share their approach with the class.
Check off the strategies used that are on the list and add new ones, if applicable.
Ask students the following question:
- How many more books do we need to get 100?
Follow the same steps as above.
Consolidation of Learning
Duration: approximately 40 minutes
Bring the students together to share the results.
Ask a few students to take turns solving the problem on the board. Be sure to choose students who did not use the same strategy.
Ask students the following questions:
- Did you use the same strategy to solve both problems? Why or why not?
- What strategy did you choose to solve the first problem? the second problem?
- Why did you choose these strategies?
- How is your second strategy similar to your first? different from your first?
- How did your knowledge of ones and tens help you solve the problems?
Examples of Success Criteria
The student:
- solves problems and explains their strategy;
- justifies the strategy used to solve the problem;
- adds and subtracts two-digit numbers with and without combining.
Differentiated Instruction
The activity can be modified to meet the needs of the students.
| To Facilitate the Task | To Enrich the Task |
|
|
Follow-Up at Home
Show Me What You Know!
Communicate, in a newsletter to parents and tutors, all the adding strategies learned in class. Provide some examples of problems that students can try to solve at home using different strategies.
Source: translated from Guide d’enseignement efficace des mathématiques de la 1re à la 3e année, Numération et sens du nombre, p. 142.
Extension 4
The Mystery Number
Give a copy of the blank chart (Appendix 2D.1) and a counter to each student or pair.
Ask students to place their counter on a starting number, such as 54.
Draw an arrow or a series of arrows on a blank chart.
Invite students to move their counters, one square at a time, in the order of the arrows to determine the mystery number chosen by the teacher.
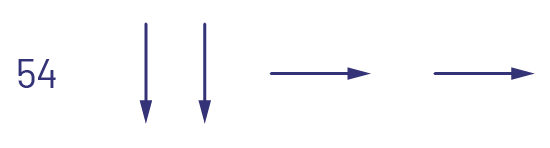
Note: The first arrow indicates that you need to move down one square, which increases the number by 10, to get to 64. Then move down another square to 74, then move one square to the right to 75, and then another square to 76, which is the mystery number. The first attempt may require a demonstration on a hundreds mat or chart.
Have students choose a mystery number, write it on a piece of paper, and provide clues, in the form of arrows, for their partner to discover.
Ask students to write the number sentence that corresponds to the path taken to discover the mystery number (for example, 54 + 10 + 10 + 1 + 1 = 76).
Source: translated from Guide d’enseignement efficace des mathématiques de la 1re à la 3e année, Numération et sens du nombre, p. 144.
Learning Situation 2: I Hit the Target!
Duration: approximately 2 hours
| Overall Expectation | Specific Expectations |
| B2. Operations Use knowledge of numbers and operations to solve mathematical problems encountered in everyday life. |
B2.1 Use the properties of addition and subtraction, and the relationships between addition and multiplication and between subtraction and division, to solve problems and check calculations. B2.2 Recall and demonstrate addition facts for numbers up to 20, and related subtraction facts. |
Learning Goals
The purpose of this learning situation is to allow the student to:
- discover the relationship between addition and subtraction;
- use addition to perform subtraction.
| Learning Context | Prerequisites |
|---|---|
|
To understand addition and subtraction, and the relationships between them. When students master counting and decomposing numbers, it is easier for them to understand the relationships between addition and subtraction. Students who know how to decompose numbers can make the connection between addition and its inverse operation, subtraction; for example, to calculate 15 - 8, students can ask themselves "15 is 8 plus what?" This strategy encourages the student to use known addition facts to determine the unknown quantity in a subtraction. A clear understanding of the relationship between addition and subtraction is essential to understanding the connections between operations and mastering basic number facts. |
In this learning situation, the student should be able to:
|
Materials
Main Activity
- Appendix 2Rel.1 (Shake and Spill!) (one copy per student or per pair)
- large sheets of paper
- manipulatives (for example, interlocking cubes, wooden sticks)
- dice (two per student or in pairs)
- two-coloured counters
- paper cups (one per student or in pairs)
- number cards (four of each number from 0 to 9) or playing cards (a suitable set for pairs or groups of four)
Extension 1
- sheets of paper or problem-solving sheets (one per student) or math journal
- a variety of manipulatives (raisins, counters, wooden sticks, etc.) (14 per student)
Mathematical Vocabulary
addition, subtraction, sum, difference, relationship
Before Learning (Warm-Up)
Duration: approximately 30 minutes
Ask students to complete the following sentences:
- Adding is…
- Subtracting is…
Invite students to share their answers and record them on the board or on a large piece of paper.
Have students discuss situations involving addition and subtraction, and think about the similarities and differences between them.
Examples
- When I play marbles, if I win I add the number of marbles to the ones I already have, and if I lose, I subtract the number of lost marbles from my marble set.
- At the market, I add up the prices of the items I buy to determine how much I have to pay. When I pay, I subtract that amount from the amount of money I pay with.
On the board or on a large piece of paper, write three numbers that have a relationship between each other (for example, 5, 7 and 12).
Ask students to think about the relationships between these numbers and how to represent this relationship using addition and subtraction (for example, 5 + 7 = 12, 7 + 5 = 12, 12 - 7 = 5, 12 - 5 = 7).
Encourage students to use manipulatives to explore and represent these relationships.
Provide students with other similar examples and encourage them to write addition and subtraction facts that demonstrate the relationships between the three given numbers.
Active Learning (Exploration)
Duration: approximately 60 minutes
Organize the games below to allow students to discover the relationships between addition and subtraction.
Shake and Spill!
Have students do this activity individually or in pairs.
Provide each student or team with a copy of Appendix 2Rel.1, a cup, two dice, and two-coloured counters.
Explain to students the steps to follow:
- Roll the dice and find the sum of the two numbers.
- Place the same number of two-coloured counters in a cup.
- Shake the cup and toss the counters to obtain a combination of two colours (for example, 11 counters: 4 red side-up and 7 yellow side-up).
- Write four number sentences on your worksheet to describe the resulting colour combination (for example, 7 + 4 = 11, 4 + 7 = 11, 11 - 7 = 4, 11 - 4 = 7).
Ask students to explain what they learned from this activity.
Hit the Target
Prepare the material required for the activity, which is a set of 40 number cards (4 cards of each number from 0 to 9) per team. It is also possible to use a deck of cards and adapt it as follows: use aces as 1 and jacks as 0.
Group students into teams of two or four.
Have a student deal six cards to each member of their team and place the remaining cards face down in the centre of the table.
Explain to students the steps to follow:
- One student rolls the dice and finds the sum of the two numbers. This number becomes the target for the round.
- Each student takes a turn and tries to find two number cards that can be added or subtracted to get the target number.
- A student who can "hit the target" shows their two cards to the others and explains how to add or subtract them to get the target number. If all team members agree, the student sets the two cards in their discard pile and replaces them with two more from the centre pile. If a student cannot "hit the target" with their cards, the turn passes to the next player.
- When everyone has had a turn, students keep their cards and the next player rolls the dice to determine a new target number.
- The game continues until there are no more cards in the centre of the table.
Circulate around the classroom and observe students playing.
Guide students who are struggling to find addition or subtraction with their cards with questions or prompts, such as:
- I see you have a 5. What number can you add to it to get 9?
- Can you use the 8 and subtraction to reach the target number?
- Redo your calculation to see if this sum or difference is correct.
Consolidation of Learning
Duration: approximately 30 minutes
Gather the students and ask them the following questions:
- Was it difficult to think about both addition and subtraction? Why or why not?
- Was it easier to think about addition or subtraction? Why or why not?
- What is the relationship between addition and subtraction?
- As the game progressed, did the rounds get faster or slower? Why or why not?
Invite students to record their thoughts in their math journals.
Examples of Success Criteria
The student:
- explains the relationship between addition and subtraction;
- uses addition to perform a subtraction;
- explains the strategy(ies) that allowed them to solve the various operations.
Differentiated Instruction
The activity can be modified to meet the needs of the students.
| To Facilitate the Task | To Enrich the Task |
|
Use unnumbered dice on which larger numbers can be written (for example, 4, 5, 6, 7, 8, 9). |
