B1.5 Describe what makes a number even or odd.
Skill: Describing the Characteristics of Even and Odd Numbers
Divisibility relationships between two numbers are relationships based on division. For example, there is a divisibility relationship between 6 and 2, since 6 is divisible by 2. Studying this characteristic of whole numbers helps students develop their number sense and better use numbers in everyday situations.
With students, however, it is important to avoid focusing on "tricks", terminology and definitions. What is
important for students is to develop a conceptual understanding of this characteristic through activities that focus
on recognizing divisibility relationships.
If a whole number is divisible into two equal parts, it is an even number; otherwise, it is an odd number. The
definition of even and odd numbers is therefore associated with the relation of divisibility or non-divisibility by
two.
It is in the context of activities that students should be led to note that all whole numbers with the digit 0, 2, 4, 6 or 8 in the ones position are even numbers, while those with the digit 1, 3, 5, 7 or 9 in the ones position are odd numbers. These observations allow them to quickly recognize whether a number is even or odd without having to check the divisibility by two.
Source: Guide d'enseignement efficace des mathématiques de la 4e à la 6e année, p. 55 et 56.
Students can use manipulatives to discover the connection between even numbers and divisibility by two (for example, by attempting to evenly divide an even number or an odd number of cubes between two students).
Source : Guide d’enseignement efficace des mathématiques de la 1re à la 3e année, p. 30.
Example
Karim says that the number 26 is an even number. Is he right? How do you know that?
Strategy 1
Divide a quantity into two equal groups using manipulatives
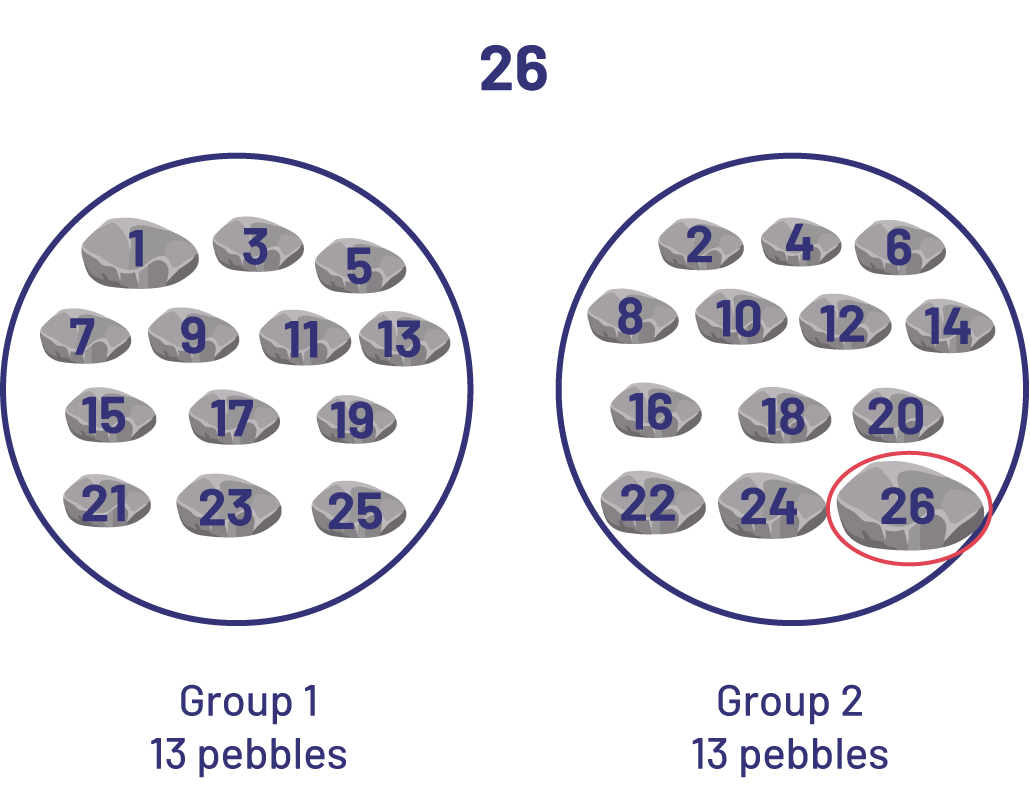
I start by counting 26 pebbles. I place one pebble in group 1 and another in group 2. I continue to divide the pebbles into each group in turn until they are all placed. I see that there are 13 pebbles in each group, which is an equal amount. By dividing 26 objects into two equal groups, I see that 26 is an even number.
Strategy 2
Use even and odd number patterns and a number line
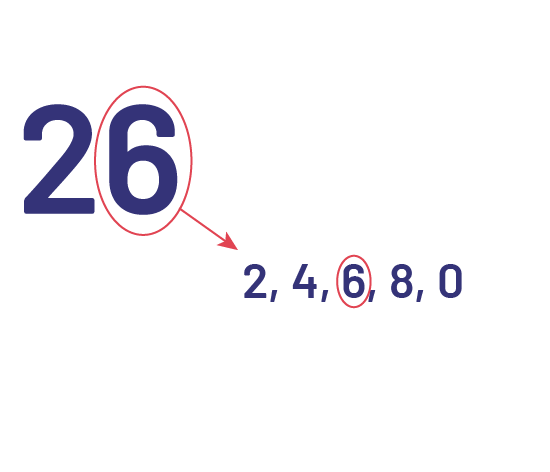
I know that numbers that have 2, 4, 6, 8 or 0 in the ones position are even numbers. In the number 26, the number 6 is in the ones position, so 26 is an even number. To check, I use a number line and count by 2s.
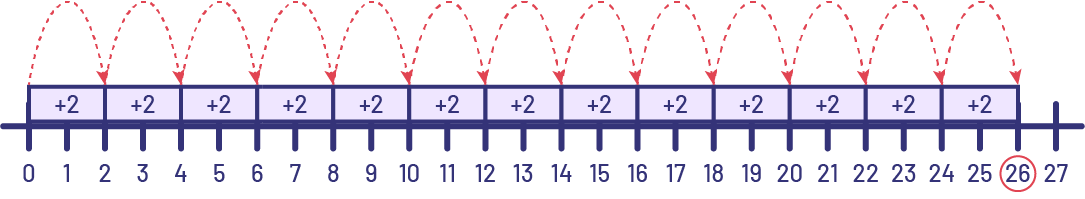
Source: En avant les maths! 2e année, CM, Nombres, p. 3.