B2.4 Use objects, diagrams, and equations to represent, describe, and solve situations involving addition and subtraction of whole numbers that add up to no more than 100.
Skills: Representing, Describing and Solving Situations
In addition to teaching students mathematical operations through strategies and reasoning, it is important to introduce students to operations through problem solving contexts. When solving problems, it is important to encourage students to use what they already know and to make connections to new learning. These connections can be weak if the students are not presented with a diverse range of problems. Students who do not learn to calculate in problem solving contexts may also have great difficulty making these connections later. Their understanding of the abstract concept of number and its application may be blurred, and some may not be able to use computational strategies effectively to solve problems.
The use of problem solving contexts is equally important for operations involving multi-digit numbers. When students are presented with problems and encouraged to come up with flexible strategies, they are given the opportunity to deepen their understanding of mathematical operations. In the interest of efficiency and in the belief that they are doing the right thing, teachers often teach students an algorithm to perform operations on multi-digit numbers. Because this method is presented to them as the "right way" to solve the problem, students work hard to understand and remember this procedure. However, this teaching often results in inefficient use of the algorithm, lack of accuracy, and limited understanding. If, instead, students are encouraged to make sense of the problem and develop their own strategies for solving it, they will show more fluency and accuracy in their work with operations.
Source: translated from Guide d'enseignement efficace des mathématiques de la maternelle à la 6e année, Fascicule 5, p. 5.
In this regard, the use of problems, namely, can help to develop understanding of operations and when to use them.
Problems Involving Addition and Subtraction
Students develop an understanding of addition and subtraction and number relationships by solving problems. For example, Pascale has some marbles; someone gives her 3 more and she now has 8. How many did Pascale have originally? While an adult may not see any difficulty in solving this type of problem, a child may. By using modelling to represent the problem and then attaching an operation to it, teachers help students make connections between conceptual understanding and procedural mastery in problems of varying degrees of complexity.
The following types of problems presented (with examples) can help students envision basic addition and subtraction facts in a variety of ways: joining, separating, part-part-whole and comparing. Using problems to introduce basic number facts compels students to reason their way to solutions and to make a connection to how math is used in everyday life.
Source: translated from Guide d’enseignement efficace des mathématiques de la maternelle à la 6e année, Fascicule 5, p. 8.
Joining and separating problems are perceived by students as active situations, easier to model and "see" as the start quantity increases or decreases. Part-part-whole problems assume a static situation, as no action or change occurs, making them more abstract and difficult to understand. In contrast, comparing problems deal with the difference between two quantities—there’s no action, just a comparison of one quantity to another.
Source: translated from Guide d’enseignement efficace des mathématiques de la 4e à la 6e année, Numération et sens du nombre, Fascicule 1, Nombres naturels, p. 83.
Joining Problems
The part-whole model can be useful for representing known and unknown values in joining problems. The set model is also useful for representing the addition of a quantity.
- Joining: Result Unknown
- Jamil has 6 candies. He buys 5 more. How many pieces of candy does Jamil have now?
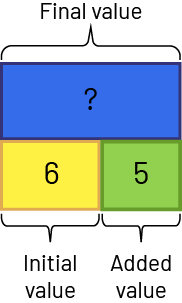
\(\ 6 + 5 = \mathord{?}\)
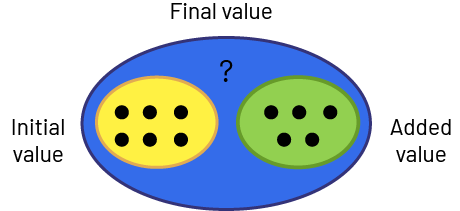
\(\ 6 + 5 = \mathord{?}\)
- Joining: Start Unknown
- Joining: Start unknown. Jamil has some candy. He buys 5 more. He now has 11. How many candies did Jamil have initially?
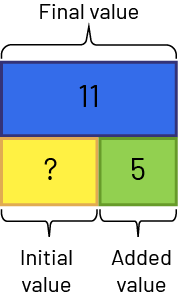
\(\mathord{?} + 5 = 11\)
- Joining: Change Unknown
- Jamil has 6 candies. He buys a few more. He now has 11 pieces. How much candy did Jamil buy?
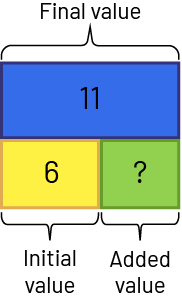
\(\ 6 + \mathord{?} = 11\)
Separating Problems
The part-whole model can be useful for representing known and unknown values in separating problems. The set model is also useful for representing the removal of a quantity.
- Separating Unknown: Result Unknown
- Nadia has $15. She gives $5 to her brother. How many dollars does she have left now?
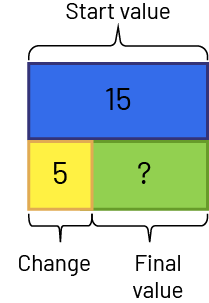
\(\displaylines{\begin{align}15 - 5 &= \mathord{?} \\ 15 - 5 &= 10\end{align}}\)
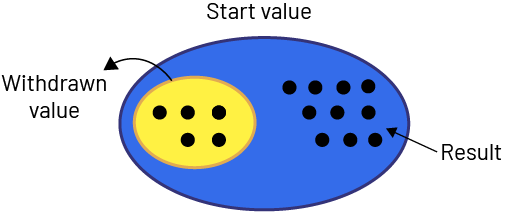
\(\displaylines{\begin{align}15 - 5 &= \mathord{?} \\ 15 - 5 &= 10\end{align}}\)
- Separating: Change
- Nadia has $15. She gives a few dollars to her brother. She now has $10 left. How many dollars did Nadia give to her brother?
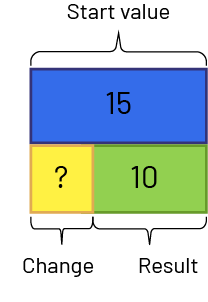
\(\displaylines{\begin{align}15 - \mathord{?} &= 10 \\ 15 - 10 &= 5 \mathord{, \ donc} \ 15 - 5 = 10\end{align}}\)
- Separating: Start Unknown
- Nadia had a certain number of dollars. She gave her brother $5. She now has $10 left. How many dollars did Nadia have initially?
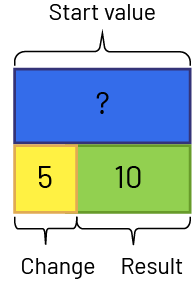
\(\displaylines{\begin{align}\mathord{?} - 5 &= 10 \\ 10 + 5 &= 15 \mathord{, \ donc\ }15 - 5 = 10\end{align}}\)
Part-Part-Whole Problems
The part-whole model can be useful for representing known and unknown parts or the known and unknown whole in part-part-whole problems.
- Part-Part-Whole: Part Unknown
- Sonia has 8 coloured pencils. Three of these crayons are red. The remaining crayons are blue. How many blue crayons does Sonia have?
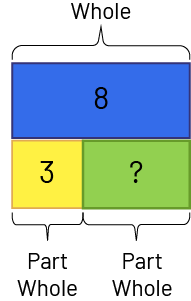
\(\displaylines{\begin{align}8 - 3 &= \mathord{?} \\ 8 - 3 &= 5\end{align}}\)
- Part-Part-Whole: Whole Unknown
- Sonia has 3 red crayons and 5 blue crayons. How many crayons does Sonia have?
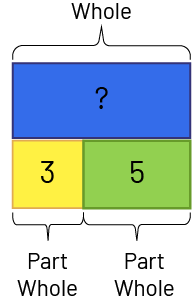
\(\displaylines{\begin{align}\mathord{?} - 3 &= 5 \\ 5 + 3 &= 8 \mathord{, \ donc\ }8 - 3 = 5\end{align}}\)
Comparing Problems
A linear model can be useful for representing the difference between two numbers in comparing problems. In this example, we use the relational rods and a double number line.
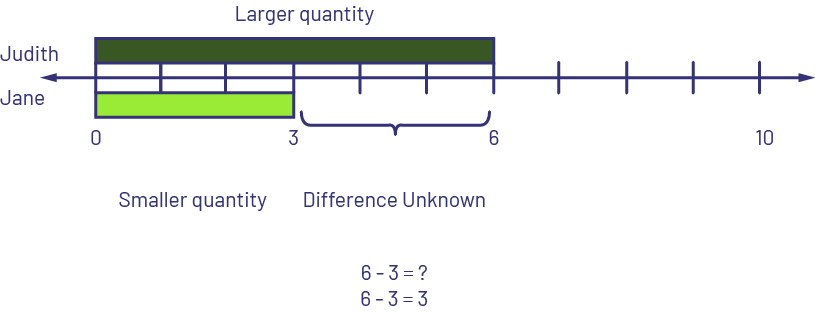
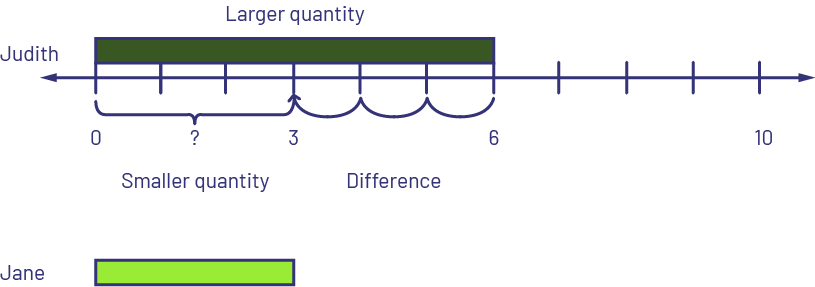
- Comparing: Difference Unknown
Judith has $6 and Jane has $3. How many more dollars does Judith have than Jane? or
Judith has $6 and Jane has $3.
How many dollars does Jane have less than Judith?
I know that the dark green rod represents 6, so I placed it at the top of the number line starting at 0. I know that the lime green rod represents 3, so I placed it below the number line at 0. I compared the two rods and saw that the lime green rod is 3 less than the dark green rod. I found the difference or gap between the two quantities. There is a difference of $3. Jane has $3 less than Judith.
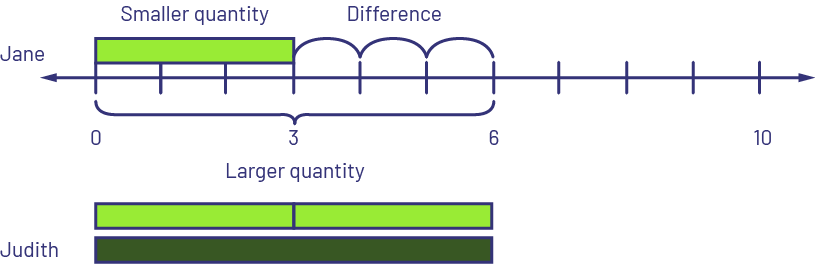
- Comparing: Larger Quantity Unknown
Judith has $3 more than Jane. Jane has $3. How many dollars does Judith have? or
Jane has $3 less than Judith.
Jane has $3. How many dollars does Judith have?
I know that the lime green rod represents 3, so I placed it at the top of the number line starting at 0. I took another lime green rod and placed it under the number line at 0. I added another lime green rod, since Judith has $3 more than Jane. I replaced the two lime green rods with the dark green rod that represents 6. Judith has $6.
- Comparing: Smaller Quantity Unknown
Judith has $6 and Jane has $3 less than Judith. How many dollars does Jane have? or
Jane has $3 less than Judith.
Judith has $6. How many dollars does Jane have?
I know that the dark green rod represents 6, so I placed it at the top of the number line at 0. On the number line, I counted backwards three jumps and come up with 3 to represent that Jane has $3 less than Judith. I took the lime green rod because it went from 0 to 3 and represents how much Jane has. Jane has $3.
Source: translated from Guide d’enseignement efficace des mathématiques de la maternelle à la 6e année, Fascicule 5, p. 9-10.
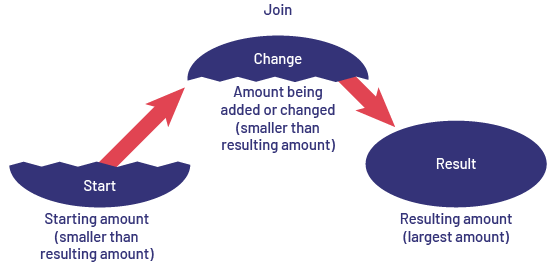
Knowledge: Join Problem
In joining problems (with whole numbers), the result is the largest number.
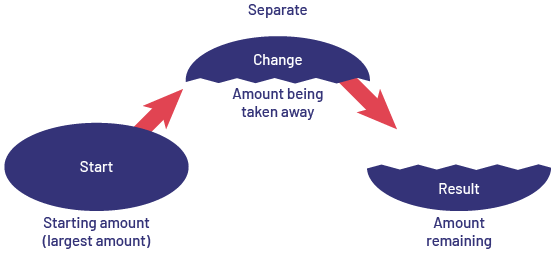
Knowledge: Separate Problem
In separate problems with whole numbers, the first amount is the largest.
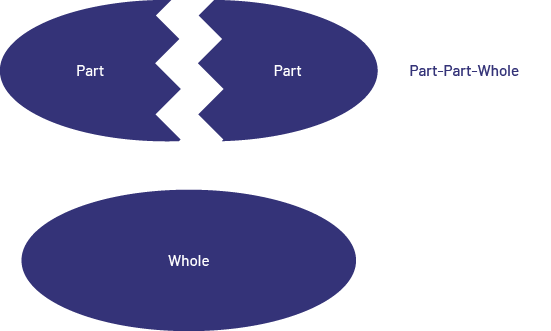
Knowledge: Part-Part-Whole Problems
Problems that focus on the part-part-whole relationship include two parts that are joined together as a whole.
Knowledge: Comparing Problems
In comparing problems, two quantities are compared. The third quantity is the difference.
Source: translated from Guide d’enseignement efficace des mathématiques de la maternelle à la 6e année, Fascicule 5, p. 9.
