B2.5 Represent multiplication as repeated equal groups, including groups of one half and one fourth, and solve related problems, using various tools and drawings.
Skill: Representing and Solving Problems Involving Multiplication as Repeated Addition
Modelling and Counting
Initially, students usually need to model the facts using their fingers or concrete materials. In the case of multiplication, students model these operations with concrete materials, tallies, or drawings that represent objects organized into groups. They often then count these objects to find an answer. Students use these models to find a solution to problems such as "Three bowls contain apples. Each bowl contains 5 apples. How many apples are there in total?". Students can draw the bowls and count the apples drawn to determine the total number of apples.
Source: translated from Guide d’enseignement efficace des mathématiques de la maternelle à la 6e année, Fascicule 5, p. 12.
To understand multiplication, students need to make connections to other mathematical concepts. In particular, students need to know that multiplication can be interpreted as repeated addition. Students also need to be able to create groups or sets of equal size.
Source: translated from Guide d’enseignement efficace des mathématiques de la maternelle à la 6e année, Fascicule 5, p. 80.
Effect of Operations
Each operation has an effect on the quantities involved. Depending on the operation, certain quantities increase or decrease. They may increase or decrease by a lot or a little. Tracking the effect of operations on numbers allows students to make connections between operations and to anticipate the outcome of an operation.
For example, we can compare the effect produced by addition on whole numbers with that produced by multiplication on whole numbers. Compared to multiplication, addition increases a number by a small amount. When the number 2 is multiplied by 8, the result is 16, whereas if you add 8, the result is only 10. People with good operational sense recognize the effect of operations on whole numbers, but care must be taken when generalizing, as operations on decimal numbers or fractions may have different effects than operations on whole numbers. In some cases, the effect may even be the opposite. For example, if you multiply one whole number by another whole number, the product is larger than both factors (for example, if you multiply 3 by 6, the product 18 is larger than both 6 and 3), whereas if you multiply a proper fraction by a whole number, the product is smaller than either factor (for example, if you multiply one half by 6, the product 3 is smaller than 6).
Source: translated from Guide d’enseignement efficace des mathématiques de la 4e à la 6e année, Numération et sens du nombre, Fascicule 1, Nombres naturels, p. 90-91.
Initially, multiplication is presented as two factors that result in a product, such as:
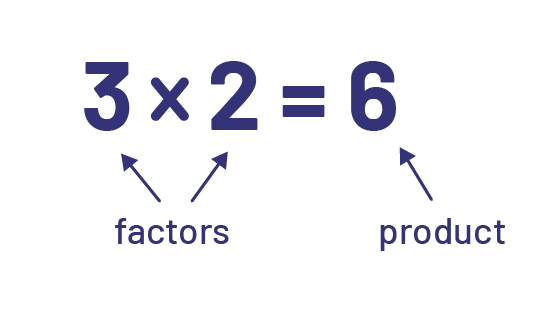
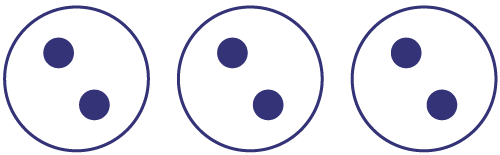
There are two interpretations that students should understand. One is that the first factor represents the number of groups, while the second factor represents the number in each group. For this example, there are three groups of two.
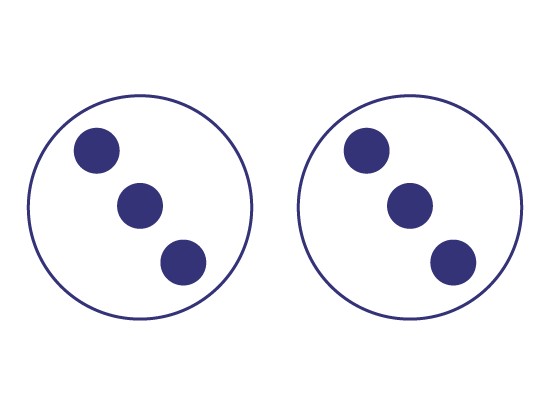
The same number sentence 3 × 2 can also be interpreted using the words "multiplied by". Then 3 × 2 reads "three multiplied by two", which creates the image of groups of three, twice.
Both ways of interpreting the number sentence are correct. The context from which the number sentence comes helps to determine the best representation.
Source: translated from Guide d'enseignement efficace des mathématiques de la 4e à la 6e année, Numération et sens du nombre, Fascicule 1, Nombres naturels, p. 80.
Types of Problems Related to Multiplication
Students develop an understanding of multiplication as well as number relationships by solving problems. The types of problems presented below, with examples, can help students see the basic multiplication facts in a variety of ways, using equal group problems. Using problems to present basic number facts compels students to reason their way to solutions, which helps them develop a better sense of operations.
The sample written problems below contain one-digit numbers. The structures of the 3 types of written problems also lend themselves to multi-digit numbers. The problems are represented using relational rods.
Equal-Group Problems
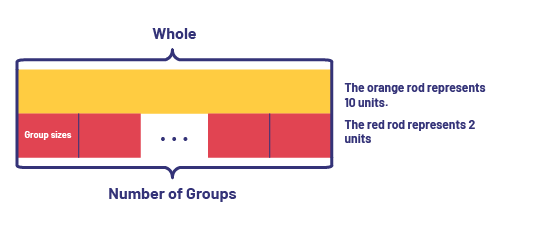
- Equal Groups: Whole Unknown (multiplication)
Julie bought 5 books for her classmates. Each book cost her $2. How much did Julie spend on all these books?
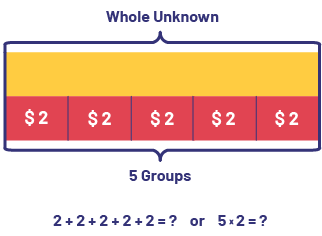
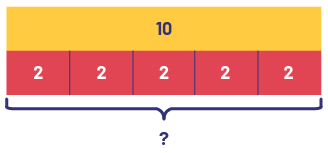
- Equal groups: Number of Groups Unknown (Equal Distribution or Partitive Division)
Julie has bought 10 books for her classmates and is making gift bags. She puts 2 books in each bag. How many gift bags did Julie use?
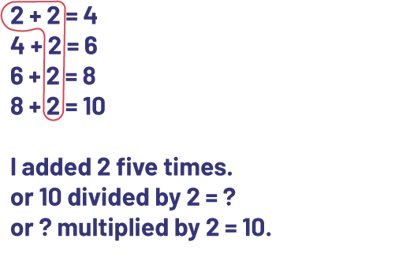
- Equal Groups: Size of Groups Unknown (Sharing or Measurement Division)
Julie has 10 books. She wants to give them to 5 of her classmates. How many books will each classmate receive?
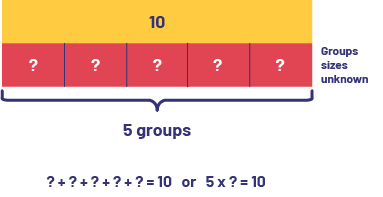
Source: translated from Guide d’enseignement efficace des mathématiques de la maternelle à la 6e année, Fascicule 5, p. 10-11.
Knowledge: Equal Group Problems
With equal group problems, a group of a given size is repeated a certain number of times to create a whole. Sometimes the size of each group is unknown, sometimes the number of groups is unknown, and sometimes the whole is unknown.
