B1. Number Sense
Demonstrate an understanding of numbers and make connections to the way numbers are used in everyday life.
Learning Situation: The Cubit
Total duration: approximately 1 hr 30 mins
| Overall Expectation | Specific Expectations |
|
B1. Number Sense Demonstrate an understanding of numbers and make connections to the way numbers are used in everyday life. |
B1.7 Read, represent, compare, and order decimal tenths, in various contexts.
B1.9 Describe relationships and show equivalences among fractions and decimal tenths, in various contexts. |
Learning Goals
The purpose of this learning situation is to allow students to:
- establish the relationship between fractions with a denominator of ten and their representation in decimal notation;
- understand the meaning of a decimal number;
- develop and use problem-solving strategies.
| Learning Context | Prerequisites |
|---|---|
| In this learning situation, students discover the relationship between fractions and decimal numbers by measuring paper strips using a non-standard unit of measurement. Later, students represent decimal tenths in a variety of ways. | To be able to complete this learning situation, students must be able to:
|
Mathematical Vocabulary
fraction, denominator, numerator, decimal number, tenth, integer, ten, parts of a whole, decimal part
Material
- Appendix 4.1 (Measurement of Strips) (1 copy per team of two)
- kits (1 per pair, see Before Learning)
- play money
- scissors
- construction paper
- relational rods
- base ten blocks
- interlocking cubes
- grid paper
Before learning (Warm-Up)
Duration: about 40 minutes
Prepare kits containing five labelled paper strips ("Cubit", "Strip A", "Strip B", "Strip C" and "Strip D") and a copy of Appendix 4.1. Provide one kit for each team of two students.
Note: Paper strips, which can be cut from unlined sheets of chart paper must be exactly the lengths specified in the table below.
| Strip | Length |
|---|---|
| Cubit | 50 cm |
| Strip A | 15 cm |
| Strip B | 40 cm |
| Strip C | 60 cm |
| Strip D | 70 cm |

Present the cubit as a unit of measurement as follows:
Today, we're going to use a very old unit of measurement, one that was used by the Romans over 2000 years ago. This unit of measurement is called the "cubit". Do you have any idea what it represented? Originally, the cubit represented the distance between the elbow and the end of the hand. Consequently, its length varied from person to person. That's why, over time, the cubit was standardized to match this length (show cubit).
Estimate the dimensions (length, width, or height) of some objects or the height of some students using this unit of measurement (for example, the desk is about 1.5 cubits wide; Sergio is about 3 cubits tall).
Group students in pairs and distribute a kit to each team. Explain that the first task is to measure Strips A, B, C and D with the cubit and record the result on the Measurement of Strips - First Attempt chart in Appendix 4.1. Review the chart with them to ensure that they understand the task at hand and invite them to begin taking measurements. Clarify that they are not to use a ruler. When the teams have completed the task, do a quick share by compiling some of the measurements in the first table in Appendix 4.1 on the whiteboard. Ask students to explain the measurement strategies used.
Example
| Strip | Measurement in Cubits | ||
|---|---|---|---|
| A | \(\frac{1}{{ 4 }}\) | \(\frac{1}{{ 3 }}\) | \(\frac{2}{{ 5 }}\) |
| B | \(\frac{3}{{ 4 }}\) | \(\frac{7}{{ 8 }}\) | \(\frac{5}{{ 6 }}\) |
| C | \(1 \frac{1}{5} \) | \(1 \frac{1}{4} \) | \(1 \frac{2}{10} \) |
| D | \(1 \frac{2}{5} \) | \(1 \frac{1}{2} \) | \(1 \frac{2}{4} \) |
Note: In this task, the goal is not to determine the exact measurement of the strips, but rather to encourage students to evaluate each measurement in cubits and express it using a fraction.
Ensure that students have understood the importance of fractions, by asking questions such as:
- How did you measure the strips since they were smaller or larger than the cubit? (Estimations were made or the strip was folded into equal parts to determine the fraction of the cubit)
- Why did you use fractions?
- Why are there so many different answers? (Not all teams chose the same fraction or it is difficult to get accurate measurements because the cubit is not graduated into smaller units of measurement)
- What could make the task easier? (Equally partition the cubit like a ruler.) Invite students to divide the cubit into 10 equal parts, or 10 sections of 5 cm. Allow them to use a ruler to make this division.

Ask them to take the measurement of each strip using the cubit divided into tenths and record it in the second column of the table Measurements of Strips - Second Attempt in Appendix 4.1.
Once the work is complete, invite students to propose their measurements in cubits and record them in a second table in Appendix 4.1.
Example
| Strip | Measurement in Cubits | My Measurement |
|---|---|---|
| A | \(\frac{3}{{10}}\) | |
| B | \(\frac{8}{{10}}\) | |
| C | \(1 \frac{2}{10} \) | |
| D | \(1 \frac{4}{10} \) |
Point out that the measurements taken by the different teams are now very similar, since they were taken with cubits divided into 10 equal parts, or tenths. Point out that the more the unit of measurement is partitioned, the more precise the measurement (for example, if the cubit were divided into hundredths, the measurement would be even more accurate) and that to describe the measurement consistently, the partitioning into fractional parts must be standardized (for example, into tenths).
Point out the importance of the decimal system and its notation. For example, tell students:
- During this second attempt, you measured the strips with the cubit divided into tenths. These fractions are so important that mathematicians have created a special notation to represent measurements without using fractional notation. Before examining this notation, enter your measurements in the third column of your table by inventing your own notation. If some already know the notation agreed by mathematicians to represent tenths, you can also use it.
Invite a few students to share their notation suggestions with the class (for example, Strip C: 1*2, 1#2, 1^2). If no team used decimal notation, add the measurements in decimal notation (for example, Strip C: 1.2). Take the measurements written in decimal form to explain how decimal notation works.
Note: In French, the comma is used to separate the integer part of a number from the decimal part, whereas in English, the period (.) is used. The digit immediately to the right of the decimal point in a decimal number is the numerator of a fraction expressed in tenths.
Example

Point out to students that the number 1.8 reads "one and eight tenths" and not "one point eight". Point out the difference between "ten" (ten times larger than the unit) and "tenth" (ten times smaller than the unit) by pointing out their phonetic similarity.
Active Learning (Exploration)
Duration: approximately 30 minutes
Group students into pairs and ask them to represent the numbers 0.7 and 1.3 in two different ways without using the same materials. Explain that they can represent them using concrete materials or drawings.
Provide students with the necessary materials (for example, rulers, plastic coins, graph paper, construction paper, interlocking cubes, base ten blocks). Allow sufficient time for them to complete the task. Circulate around the classroom and observe the strategies used by students. Provide support as needed, without explicitly showing students how to do it. Specify that they must be able to explain their representation. Examples of representations that students could propose include the following:
Representations of 0.7
Example 1
If the cubit represents the whole (unit), the seven purple squares represent seven tenths.

Example 2
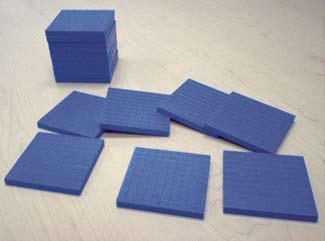
If the large cube represents the whole (unit), seven flats represent seven tenths.
Representations of 1.3
Example 1
If the dollar represents the whole (unit), the following coins represent one and three tenths.

Example 2
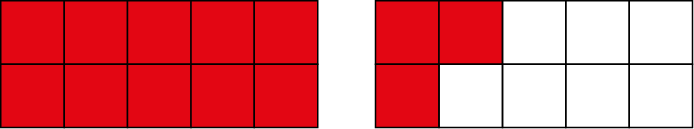
If the rectangle represents the whole (unit), the red represent one and three tenths.
Example 3
If the orange rod represents the whole (unit), the following represents one and three tenths.

| Possible Observations | Possible Interventions |
| A team doesn't know where to start. | Ask students to explain what they are representing. Ensure that students pronounce the numbers correctly (for example, seven tenths). Repeat, emphasizing the word "tenths" so that students can make the connection between the term "seven tenths", the notation 0.7 and the visualization of 7 parts of a whole divided into 10 parts. Suggest wholes they could use. |
| A team represents 0.7 using 7 objects. | Have them recognize that they need to identify the whole, or unit. Discuss with them, for example, "I see seven cubes, so you have represented seven ones. Explain to me why you say it's seven tenths (It is seven tenths of a set of ten small cubes or it is seven tenths of a base ten rod.) |
Consolidation of Learning
Duration: approximately 20 minutes
Place two tables in front of the class, one for each number (0.7 and 1.3), and invite each team to place one of their representations on the corresponding table. Ask students to circulate around the tables to see the representations.
Choose some of the representations of the first number (0.7) and invite the teams that created them to take turns explaining them.
Facilitate the discussion by asking questions such as:
- Have other teams used this representation?
- Does this representation really show seven tenths?
- Can you justify the accuracy of this representation?
- What is the unit or whole in this representation?
- What is similar between the representations?
Do the same for representations of the number 1.3. Be sure to make the following points during the math discussion:
- The decimal part of a decimal tenth can be represented by a fraction whose denominator is 10 \(0.7 \ is \ \frac{7}{10} \ and \ 1.3 \ is \ 1 \ and \frac{3}{10}\);
- The decimal point (period) separates the whole number part from the decimal part;
- A decimal number is read using the word "and" and not the word "decimal" (for example, 1.4 is read "one and four tenths");
- When using a decimal number, it is important to specify the whole or the unit.
Differentiated Instruction
The activity can be modified to meet the needs of the students.
| To Facilitate the Task | To Enrich the Task |
| Provide the whole to be used for the representations. |
|
Source: translated from Guide d’enseignement efficace des mathématiques de la 4e à la 6e année, Numération et sens du nombre, Fascicule 3, Nombres décimaux et pourcentages, p. 133-144.
