B1.3 Round whole numbers to the nearest ten, hundred, or thousand, in various contexts.
Skill: Rounding Whole Numbers to the Nearest Ten, Hundred or Thousand
Rounding a number can make it easier to work with. This method is often used to make estimates, measurements and quick comparisons.
A rounded number remains close to the original number depending on the unit it is being rounded to. The larger the unit, the broader the approximation; the smaller the unit, the more precise.
Whether a number is rounded up or down depends on the context. When paying by cash in a store, the amount owing is rounded to the nearest five cents (or nickel). Contrarily, if they are looking at a pile of coins, they may want to round down to ensure they have enough money.
In the absence of a context, numbers are typically rounded on a midpoint, for example:
- Rounding 1237 to the nearest 10 becomes 1240, since 1237 is closer to 1240 than to 1230.
- Rounding 1237 to the nearest 100 becomes 1200, since 1237 is closer to 1200 than to 1300.
- Rounding 1237 to the nearest 1000 becomes 1000, since 1237 is closer to 1000 than to 2000.
- If a number is exactly on the midpoint, convention rounds the number up (unless the context suggests differently). So, 1235 rounded to the nearest 10 becomes 1240.
With the elimination of the penny, cash transactions are now rounded to the nearest five cents (or nickel).
Source: Ontario Curriculum, Mathematics Curriculum, Grades 1-8 , 2020, Ontario Ministry of Education.
Rounding
The skill of rounding up requires analysis and reflection. The operation is not limited to rounding a given number to a certain unit; it also requires the evaluation of the context in which the number is found. The activities that target the ability to round must therefore be done in context so that they reflect an authentic use of rounding, and give meaning to this learning, a meaning that will be transferred to the cognitive foundation of the students.
In everyday life, the decision to round comes from the interpretation of a situation, not from a received instruction. Therefore, the situation and the number to be rounded must be evaluated to designate a rounding unit (for example, should we round to the nearest thousand or to the nearest hundred?). Benchmark numbers can be considered based on the chosen unit (for example, to round 3620 to the nearest hundred, some students will recognize mentally or on a number line the progression of hundreds - 3000, 3100, 3200, 3300, 3400, 3500, 3600, 3700, while others will see immediately that 3620 is between 3600 and 3700). By recognizing the number closest to the number in question (3620), students will be able to determine the rounded value of the number. Eventually the goal is that this thinking is done intuitively and almost automatically. This fluency comes specifically from number sense and the knowledge one has to support this process.
In the classroom, many decisions have often already been made for students. This is when students are involved, for example, in rounding a number like 2365 to the nearest ten, hundred, and thousand, or simply determining whether 365 is closer to 300 or 400. It would be beneficial to their learning if students had the opportunity to make the full range of decisions surrounding the rounding of a number. This helps develop their critical sense of number use and deepens their sense of rounding.
The following is a situation that illustrates reasoning when rounding. The additional information offers some pedagogical insights.
There are usually between 3000 and 4000 people in attendance at "The Scene". After the show, the box office clerk counted the number of spectators and determined that there were 3736. He meets with management and decides to give them an overview of the evening's sales. What will he tell management?
| Reasoning During Rounding | Reasoning of the Clerk |
|---|---|
| Decide to round up
It makes sense to round up if the goal is to communicate a quantity using a number that can quickly become meaningful to the recipient. |
In the example, if the clerk wants to communicate the magnitude of the sales and not the exact quantity, then the number should be rounded.
However, if the clerk were to enter the numbers in the accounting ledger, then exact numbers should be used. |
| Determine at which unit the rounding will take place
There are no rules for choosing the place value at which to round. However, the choice is not arbitrary, as it depends on the interpretation of the situation and the individual's sense of number. It is important not to systematically impose on students to round to a certain place value, but to discuss different scenarios with them by giving examples and counter-examples so that they understand how to make a wise choice. |
As the sales were typically between 3000 and 4000, the clerk could round up to the nearest thousand to show that sales were in the normal range. If the clerk wanted to give a higher degree of accuracy, then the rounding should be to the nearest 10. |
|
Determine benchmark numbers based on the choice of unit Using benchmark numbers helps students see a number in relation to other numbers. Using number lines helps students visualize the relative magnitude of the number. Identifying benchmarks that relate to a number of numbers allows one to determine an appropriate interval to complete the rounding. This involves recognizing that a number such as 3736 is located between 37 hundreds and 38 hundreds. 
Identifying benchmarks can also be supported by finding a set of values related to the chosen place value (for example, if rounding to the nearest thousand, thinking about 3000 or 4000). Note: It's very important that students consider the whole number. For example, when trying to round to the nearest hundred, some students might focus only on the hundreds and say, for example, that 3736 lies between 7 hundreds (700) and 8 hundreds (800) and that the number is closer to to 700 (instead of 3700). |
In the example, the clerk determines that 3736 is between 3700 and 3800. 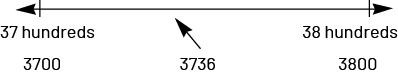 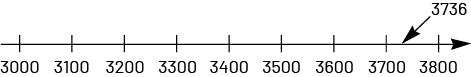 |
| Round the number
Students should understand that rounding is performed using values closest to the number. For example, when rounding to the nearest hundred, 3736 is rounded 3700, as it is closer to 3700 than to 3800. Note: If a number is exactly at the midpoint between two values, the convention is to round up. For example, when rounding to the closest hundred, 3750 is rounded 3800. |
 
In the example, the clerk needs to understand that 3736 is between 3700 and 3800, but that it is closer to 3700. Therefore, the rounding results with 3700. |
| Communicating the rounding
Since rounding is context-bound, communication must alkso include context. |
The clerk might mention to management that there were about 3700 spectators, a little over 3700 spectators or even close to 3700 spectators.
Note: It would be wrong to say that there were 3700 spectators. |
Classroom activities should allow students to develop the full range of rounding skills. It is important that teachers nuance their words during rounding activities so as not to direct students' thinking toward a particular strategy or answer. So far, rounding has been discussed to within one unit. However, the action of "rounding up" on a daily basis can be done in a broader sense. For example, a fundraiser raised $14 345, and the newspaper article will headline, "What a success, event raises nearly $15 000!" In this case, the roundig was used to "round up" an amount of money so that the information would be understood quickly. Examples of possible rounding strategies are included in the chart below.
| Strategies | Examples |
|---|---|
| Define an appropriate range | If there are 3736 people at a show, we can round up and say that there are between 3700 and 3800 people. |
| Round up to a benchmark number | If each apple costs 44 ¢ and we bought a dozen, we can round up the price to 50 ¢ per apple. |
| Rounding by thinking about the effect of rounding on the quantity | If you are preparing small gifts for each participant in a contest, it is best to buy a little more than the number of participants. For example, if there are currently 63 participants, you may choose to round up to 70 and purchase 70 gifts to ensure that you have enough for all participants. |
Source: translated from Guide d'enseignement efficace des mathématiques de la 4e à la 6e année, Numération et sens du nombre, Fascicule 1, Nombres naturels, p. 38-41.
Knowledge: Approximation (Estimating and Rounding)
Numbers represent quantities with a high degree of accuracy. They provide a precision that terms like "more", "some", "many" and "few" do not. However, they can also be used to show an order of magnitude for that quantity. In this case, the number is used to approximate the quantity (for example, about 200 people were at the party does not mean that there were exactly 200 there). In general, the approximation is a magnitude that is close enough to a known (rounding) or unknown (estimating) magnitude.
The terms "rounding" and "estimating" are often, and incorrectly, used interchangeably. The fundamental difference between these two concepts lies in how the number is generated. Estimating uses the relationship between an unknown quantity and prior knowledge, usually in the form of benchmark numbers. Rounding, on the other hand, uses the relationship between a known number (precise or approximate) and its relative proximity to other numbers. Generally, estimating and rounding are used to visualize the quantity in question and to get a sense of the magnitude of the quantity. The following table demonstrates this distinction.
| Rounding a Number | Estimate a Quantity | |
|---|---|---|
| Definition | Replace a number by an approximate value of that number appropriate to the situation, using some predefined or personal criteria. | Roughly calculate or make a reasonable guess. |
| Example | If the list price of a new car is $18 753, it costs about $19 000. | Walking through a parking lot, you notice a car and having just seen an ad for it, you estimate its price at $ 20 000. |
| Explanation | The actual price (known number) has been rounded to the nearest thousand. | The price is based on prior knowledge. |
Source: translated from Guide d'enseignement efficace des mathématiques de la 4e à la 6e année, Numération et sens du nombre, Fascicule 1, Nombres naturels, p. 35-36.
