B1.5 Using drawings and models to represent, compare, and order fractions representing the individual portions that result from two different fair-share scenarios involving any combination of 2, 3, 4, 5, 6, 8, and 10 sharers.
Skill: Using Diagrams and Models to Represent Fractions Representing Individual Portions, from Two Fair-Share Scenarios
Different models can be used to represent fractions:
Set models include collections of objects (for example, beads in a bag, stickers in a sticker book), where each object is considered an equal part of the set. The attributes of the set (for example, colour, size, shape) may or not be considered. Either each item in the set can be considered one whole or the entire set can be considered as the whole, depending on the context of the problem. If the entire set is the whole it will be important that the tool used can be easily partitioned. For example, concrete pattern blocks are difficult to partition; however, paper pattern blocks could be cut.
Linear models include number lines, the length of relational rods, and line segments. It is important for students to know the difference between \(\frac{3}{4}\) of a line segment and \(\frac{3}{4}\) as a position on a number line. Three fourths of a line segment treats the fraction as an operator and the whole is represented by the entire length of the line segment; for example, if the whole line segment represent 8 apples, then \(\frac{3}{4}\) would be positioned at the 6. Three fourths as a position on a number line treats the fraction as a part-whole relationship where the number 1 on the number line is 1 whole, the number 2 on the number line is 2 wholes, and so on. So as a position, \(\frac{3}{4}\) is located three fourths of the way from 0 to 1.
Other measurement models include area, volume, capacity, and mass. Area is the most common model used with shapes like rectangles and circles. Circles are difficult to partition when the fractions are not halves, fourths, or eighths, so providing models of partitioned circles is imperative. Making connections to the analog clock may also be helpful; for example, \(\frac{1}{4}\) past the hour.
Source: Ontario Curriculum, Mathematics Curriculum, Grades 1-8 , 2020, Ontario Ministry of Education.
Skill: Using Drawings and Models to Compare and Order Fractions Representing Individual Portions from Two Different Fair-Share Scenarios
Effective, balanced instruction allows students to develop a variety of strategies for comparing numbers. Similarly, it should allow students to develop and deepen their understanding of the value of fractions. Through concrete experiences and representations, students learn to visualize the value of a fraction and develop strategies for ordering, comparing, and relating them to other numbers.
Source: translated from Guide d'enseignement efficace des mathématiques de la 4e à la 6e année, Numération et sens du nombre, Fascicule 2, Fractions, p. 44.
In order to estimate a quantity, students must first understand the context and use their analytical skills and personal experiences to do so. This background often takes the form of a mental representation and acts as a visual benchmark. The importance of constantly building on this visual reference base by providing students with a variety of situations in a variety of contexts cannot be overemphasized. Students who are exposed to visual benchmarks and manipulatives, such as fraction circles, counters, paper strips and rulers, are more likely to develop a sense of magnitude, which is essential for estimating the quantity represented by a fraction.
Example
Ask students to estimate the fraction of the pie that is left.
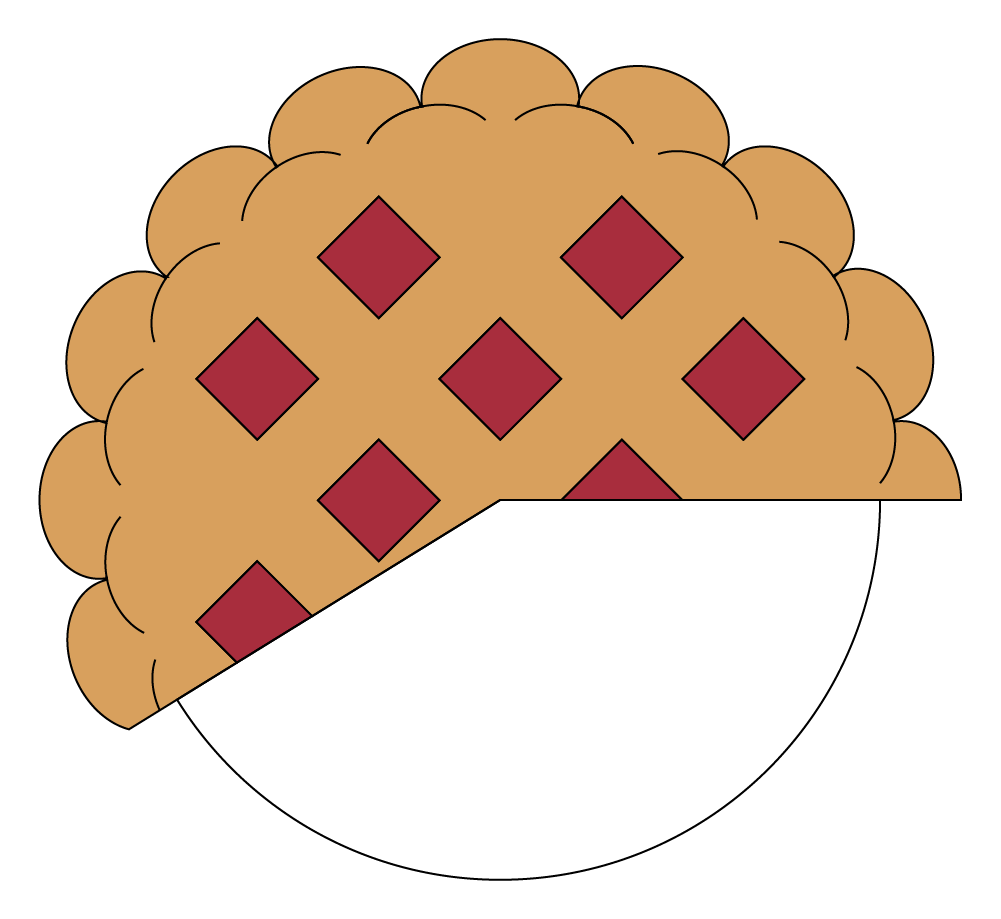
Example of student reasoning include:
- I looked at the pie and imagine it split in two. I recognized that there is more than one half left.
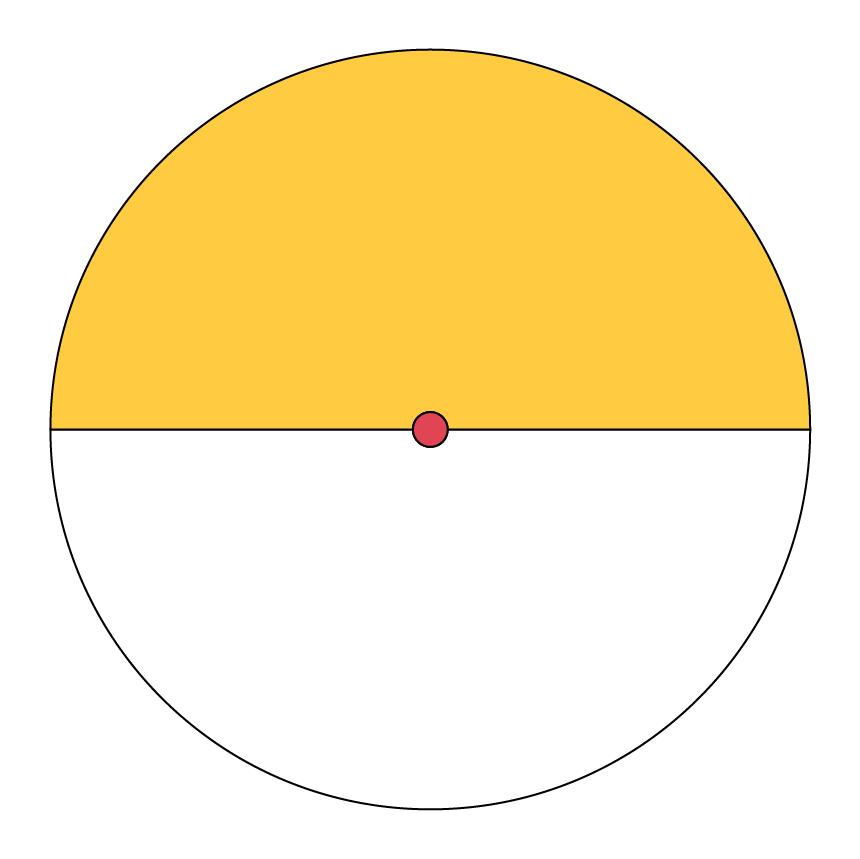
- If it was split into three equal pieces, I see that there is less than two thirds left.
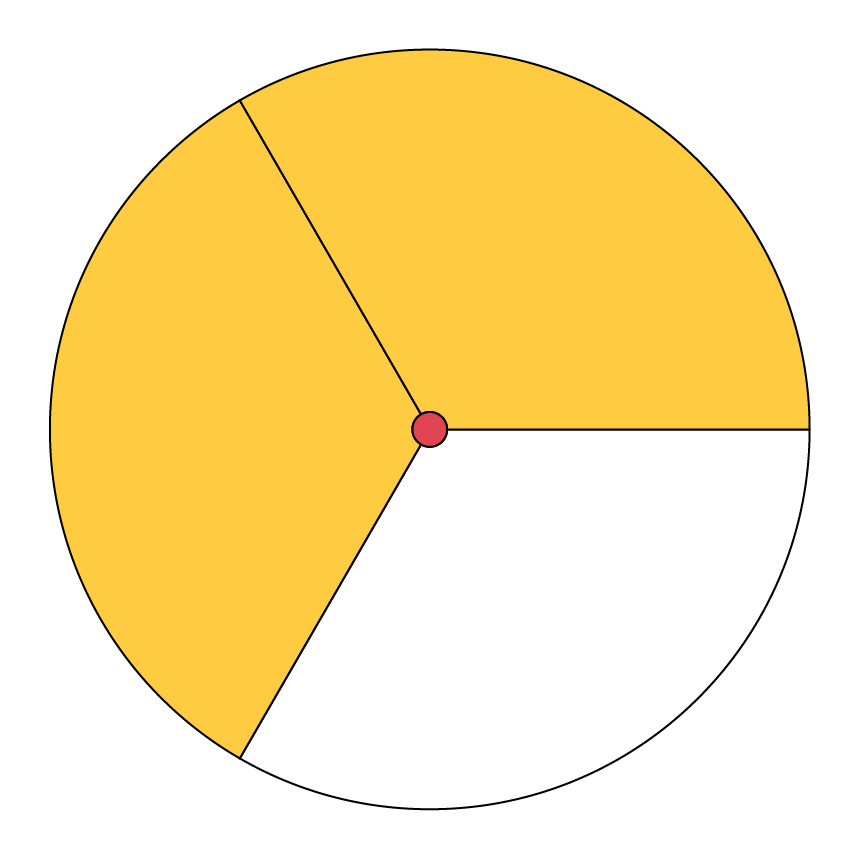
- But if it's split into five equal pieces, I think it's very close to the three pieces.
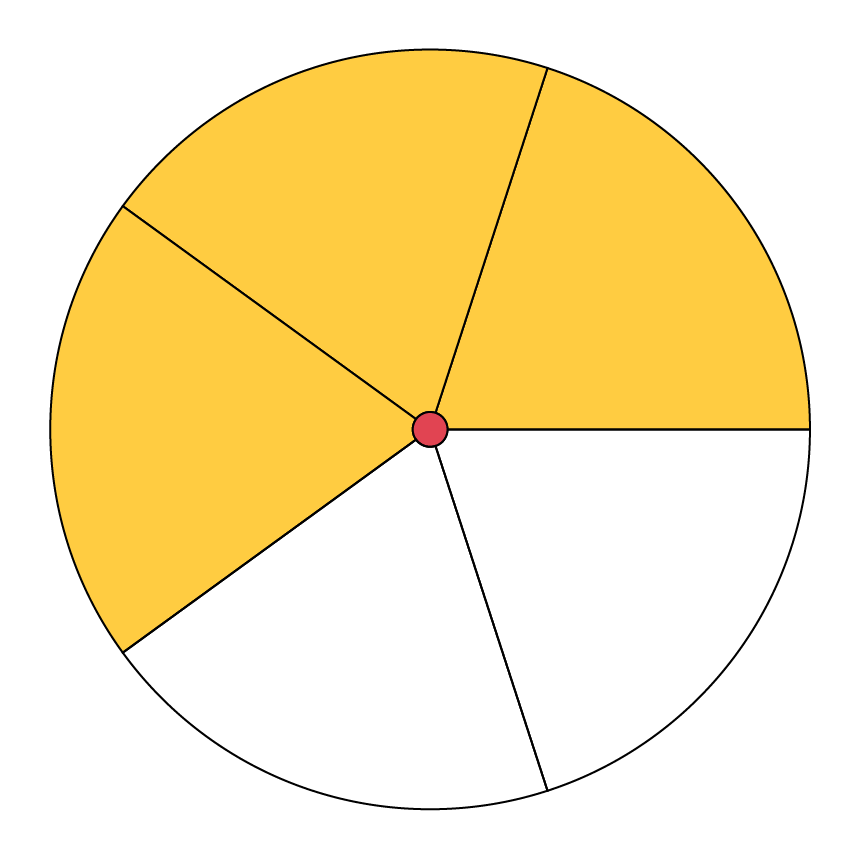
- There's about \(\frac{3}{5}\) of the pie left.
Source: translated from Guide d'enseignement efficace des mathématiques de la 4e à la 6e année, Numération et sens du nombre, Fascicule 2, Fractions, p. 38-39.
Context for Comparing Fractions
It is important that students learn to always consider the whole. A fraction is meaningless if it is not related to a whole. For example, we cannot compare a half of a pizza and a third of a pizza if they are not the same size unless we are shown the pizzas in question. Teachers need to provide learning situations that allow students to discover that a fraction does not reveal anything about the size of the whole or its parts; it only tells us about the relationship between a whole and its parts.
Source: translated from Guide d'enseignement efficace des mathématiques de la 4e à la 6e année, Numération et sens du nombre, Fascicule 2, Fractions, p. 31.
Order Relationships
It is important that students learn to order fractions by comparing them. The ability to order and the ability to compare should be based on students' constructed representations of fractions. They should arise from problem-solving situations that allow students to develop a sense of the value of a fraction. It is this sense of fraction that allows students to compare two fractions and conclude that one is greater than the other. In contrast, instruction that is done out of context tends to promote learning algorithms that neglect the meaning of the fraction.
Students in the junior grades already have strong ideas about the properties of whole numbers. However, when students begin to work with fractions, they often experience a cognitive imbalance - a sense that what was true is no longer true. For example, students have learned that when there are two whole numbers, the greater number indicates a greater quantity. Students attempt to transfer their knowledge and skills to fractions. For example, in trying to compare \(\frac{1}{6}\) to \(\frac{1}{3}\), some students believe that \(\frac{1}{6}\) is greater than \(\frac{1}{3}\), since 6 is greater than 3. They feel out of balance if they are told that they are wrong. To avoid such situations, learning must be based on an analytical view of the fraction rather than on an automatic or algorithmic approach.
Activities that lead students to compare and order fractions should promote the construction of fraction meaning. To compare fractions, it is rarely necessary to look for a common denominator. In fact, there are many strategies that allow one to compare fractions. Generally, the first strategy learned consists of the use of a concrete or visual (semi-concrete) representation of the fractions being compared. This strategy can be used, regardless of the situation. We can also compare the numerators if the denominators are the same, or compare the denominators if the numerators are the same. Using benchmark fractions is another effective strategy.
It is important to work thoroughly on the comparison of fractions before the equivalence of fractions. This is very complex and the comparison serves as a catalyst for understanding equivalent fractions.
Comparing Fractions by Representing Them Concretely or Visually
This first strategy consists of representing the fractions with a model. For example, the fractions \(\frac{2}{5}\) and \(\frac{3}{8}\) can be compared using fraction strips:
\(\frac{2}{5}\)

\(\frac{3}{8}\)
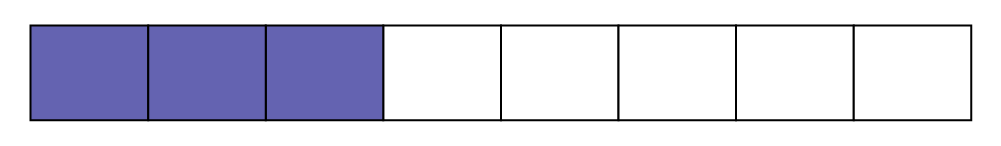
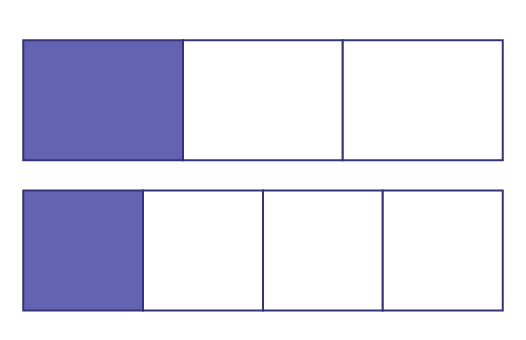
By comparing the two representations, we can conclude that \(\frac{2}{5}\; > \;\frac{3}{8}\). It is very important to emphasize that the same whole was used to represent both fractions. Students sometimes overlook the importance of the whole and do not correctly interpret situations with fractions. For example, in the following situation, students might not consider that the wholes are different, and conclude that \(\frac{1}{3} = \frac{1}{4}\).
\(\frac{1}{3}\)

\(\frac{1}{4}\)

In this situation, it is true that \(\frac{1}{3}\)of the first rectangle corresponds to \(\frac{1}{4}\) of the second rectangle. However, to compare the fractions, they should have been represented using the same whole.
\(\frac{1}{3} > \frac{1}{4}\)
Comparing Fractions with a Common Denominator
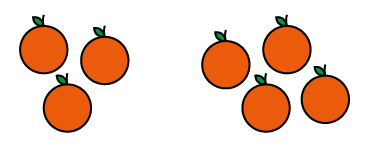
In Grade 4 , students typically draw on their prior knowledge and experience with whole numbers to determine, without difficulty, that \(\frac{4}{5}\) is greater than \(\frac{3}{5}\). However, it often happens that the answer is not motivated by the right reasons. Indeed, many students only compare numerators. Van de Walle and Folk (2005, p. 233) encourage teachers to focus on the parts that are the same size or of the same nature. For example, when comparing \(\frac{4}{5}\) and \(\frac{3}{5}\), they should emphasize that \(\frac{4}{5}\) represents 4 parts of a certain size and \(\frac{3}{5}\) represents 3 parts of that same size. The focus is on comparing elements of the same nature (fifths), like comparing 3 oranges and 4 oranges.
3 fifths and 4 fifths of a whole

3 oranges and 4 oranges
Comparing Fractions with a Common Numerator
In Grade 4, students are able to make sense of the numbers that make up a fraction and begin the reflective process of comparing two fractions with a common numerator, such as \(\frac{5}{9}\) and \(\frac{5}{6}\). In both cases, there are 5 parts of a whole being considered. When the whole is divided into 9 equal parts, these parts are smaller than when the same whole is divided into 6 equal parts. As the ninths are smaller than the sixths 5 of them will be smaller than 5 sixths, in both cases. Thus, \(\frac{5}{9}\) is smaller than \(\frac{5}{6}\).
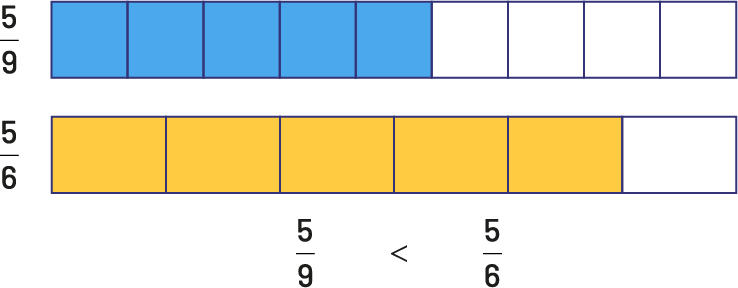
However, it is not uncommon for students to think the opposite, since 9 is greater than 6 and the numerators are the same. Hence the importance of focusing on the meaning of the numerator and denominator and developing the ability to visualize fractions.
Comparing Fractions Using Benchmark Fractions
In Grade 4 , it is suggested that students develop their fraction sense through the use of benchmark fractions. First, students use three benchmarks: 0, \(\frac{1}{2}\), and 1. They then compare given fractions to these benchmarks. The following are some generalizations that students are able to make after completing a number of learning activities.
| \(\ 0\) | \(\frac{1}{2}\) | \(\ 1\) |
|---|---|---|
| If the denominator of a fraction is much larger than the numerator, the fraction represents a small amount and the fraction is close to 0. Thus, \(\frac{3}{25}\) is close to 0. | If the numerator of a fraction is about half of the denominator, the fraction is close to \(\frac{1}{2}\). Thus, \(\frac{3}{8}\) is close to \(\frac{1}{2}\). We could add that \(\frac{3}{8}\) is slightly less than \(\frac{1}{2}\), because \(\frac{3}{8}\) is less than \(\frac{4}{8}\), that is \(\frac{1}{2}\). | If the numerator of a fraction is approximately equal to the denominator, the fraction is close to 1, because it represents almost the whole. Thus, \(\frac{9}{10}\) is close to 1. |
Here is a number line model that illustrates these examples.
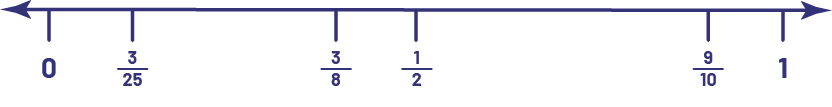
To compare 2 fractions, students can compare each to one of these benchmarks. For example, if we want to compare \(\frac{5}{{20}}\) and \(\frac{7}{8}\), we can determine that \(\frac{5}{{20}}\) represents a quantity between 0 and \(\frac{1}{2}\), while \(\frac{7}{8}\) represents a quantity between \(\frac{1}{2}\) and 1. We can therefore conclude that \(\frac{5}{{20}}\) is less than \(\frac{7}{8}\).
Comparing Fractions Using Fraction Sense
In a problem-based learning environment, students can discover many strategies for comparing fractions based on the fraction sense they have developed.
Example 1
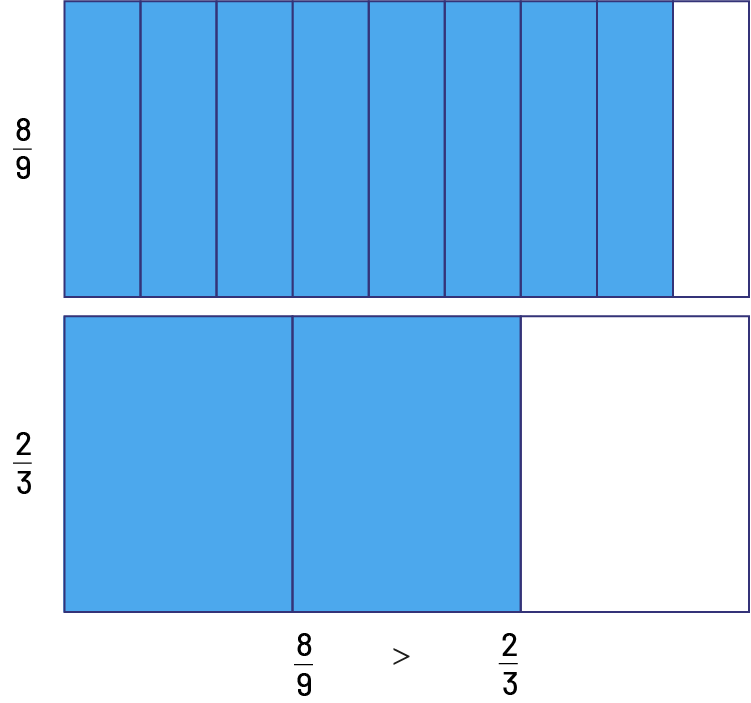
We want to compare \(\frac{8}{9}\) and \(\frac{2}{3}\). Note that each fraction lacks a part to make a whole. We thus recognize that for the fraction \(\frac{8}{9}\), \(\frac{1}{9}\) is missing to make 1, while for the fraction \(\frac{2} {3}\), \(\frac{1}{3}\) is missing. Since the missing part \(\frac{1}{3}\) is larger than the missing part \(\frac{1}{9}\), then \(\frac{8}{9}\) is closer to 1 than \(\frac{2}{3}\). Thus, \(\frac{8}{9}\) is greater than \(\frac{2}{3}\).
Example 2
We want to compare \(\frac{2}{3}\) and \(\frac{7}{{12}}\). Students could use their knowledge of equivalent fractions to conclude that \(\frac{2}{3}\) is greater than \(\frac{7}{{12}}\), because \(\frac{2}{3}\; = \;\frac{8}{{12}}\) and \(\frac{8}{{12}}\) represent more parts of the same size than \(\frac{7}{{12}}\).
As a result of learning the various concepts related to fractions, students can use the full range of knowledge about fractions and the full range of strategies for comparing fractions to solve problems.
Example 3
In some car races (for example, Formula 1), the driver who crosses the finish line first wins, at which point the race is officially over. The ranking of runners is not determined by the order in which they cross the finish line as in traditional races, but rather by the position of the drivers at the end of the course, which is the fraction of the circuit they have covered when the first driver crosses the line. This system allows all drivers to be assigned a position, even those who did not finish the race.
Here is the list of the fractions of the circuit covered by each driver at the end of the race:
Wagner: \(\frac{8}{9}\); Villeneuve: \(\frac{2}{3}\); Trotter: 1; Munch: \(\frac{1}{4}\); Santana: \(\frac{7}{{10}}\); Burk: \(\frac{5}{6}\); Background: \(\frac{3}{4}\); Colman: \(\frac{1}{9}\); Martina: \(\frac{5}{8}\).
Solution: Final ranking of the race
| 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 |
| Trottier | Wagner | Burk | Fround | Santana | Villeneuve | Martina | Munch | Colman |
| \(\;1\) | \(\frac{8}{9}\) | \(\frac{5}{6}\) | \(\frac{3}{4}\) | \(\frac{7}{10}\) | \(\frac{2}{3}\) | \(\frac{5}{8}\) | \(\frac{1}{4}\) | \(\frac{1}{9}\) |
Source: translated from Guide d'enseignement efficace des mathématiques de la 4e à la 6e année, Numération et sens du nombre, Fascicule 2, Fractions, p. 44-49.
Knowledge: Fair Sharing
Fair sharing means that a whole is shared equally. For a whole to be shared equally, it must be partitioned in such a way that each sharer receives the same amount.
Words can have multiple meanings. It is important to be aware that in many situations, fair does not mean equal, and equal is not equitable. Teachers should clarify how they are using the term “fair share” and ensure that students understand that in the math context fair means equal and the intent behind such math problems is to find equal amounts.
Source: Ontario Curriculum, Mathematics Curriculum, Grades 1-8 , 2020, Ontario Ministry of Education.
