B2.7 Represent the relationship between repeated addition of a unit fraction and multiplication of that unit fraction by a whole number, using tools, drawings, and standard fractional notation.
Skill: Representing the Relationship Between Repeated Addition of a Unit Fraction and Multiplication of that Unit Fraction by a Whole Number, Using Tools, Patterns, and Standard Fractional Notation
To count by a fractional amount is to count by a unit fraction. For example, when counting by the unit fraction one third, the sequence is: 1 one third, 2 one thirds, 3 one thirds, and so on. Activities that require counting by unit fractions can reinforce that the numerator is a count and the denominator is the fractional unit. A fractional count equivalent to the unit fraction makes one whole. For example, when a whole is divided into two equal parts, each part is called one half and two halves make a whole. Similarly, when a whole is divided into three equal parts, each part is called one third and when it is divided into four equal parts, each part is called one fourth. When there are four or more equal parts, the suffix th is added to the number of parts to obtain fourths, fifths, twentieths, and so on. A student who has mastered the concept of counting by a unit fraction does not need to recreate the image of the "pie" to understand, for example, that six sixths \(\frac{6}{6}\) form a whole. Thus, in order to identify the fraction shown below, the reasoning might be, "I counted the parts (1, 2, 3, 4, 5, and 6) and concluded that they are sixths. Since five (5) parts are shaded, I can state that 5 one sixths or five sixths (\(\frac{5}{6}\)) of the shape are shaded.
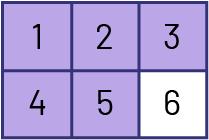
This process also leads them to recognize that a fraction can be the sum of unit fractions. Counting of unit fractions is implicitly the addition of unit fractions.
Example
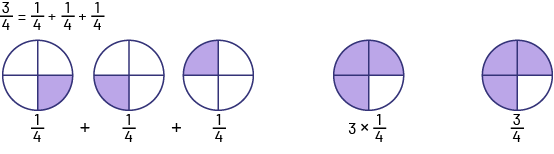
Students who have counted, for example, equivalent parts of a whole can explain that the denominator represents what is being counted (for example, sixths) and the numerator represents how many sixths there are. Van de Walle and Folk (2005, p. 229) raise the interesting point that the terms numerator and denominator are learned words that are likely better understood by adults who see the connections between numeration and numerator and denomination and denominator. They suggest that junior students' knowledge of these terms does not reflect this understanding.
Source: translated from Guide d'enseignement efficace des mathématiques de la 4e à la 6e année, Numération et sens du nombre, Fascicule 2, Fractions, p. 34-35
Fractions are used in a variety of everyday tasks such as in cooking to measure ingredients. However, fraction operations are too often perceived as a set of rules that must be learned and mastered. This perception promotes obedience to rules rather than a true understanding of computations. As a result, students new to fraction computations may learn a list of procedures that they do not understand, and therefore may forget or misapply them. This set of rules and procedures often confuses the meaning of the operation, as demonstrated by this student's question, "Can I add fractions like I do whole numbers?
Source: translated from Guide d'enseignement efficace des mathématiques de la 4e à la 6e année, Numération et sens du nombre, Fascicule 2, Fractions, p. 74.
Learning Basic Math Facts
The acquisition of computational skills must be based on the acquisition of a sense of operations, otherwise these skills remain at a superficial and mechanical level. Van de Walle and Folk (2005, pp. 244-245) suggest the following guidelines for teaching operations with fractions.
Present simple tasks in context
The principles that guide the development of student-generated problem-solving strategies apply equally to fractions and whole numbers. Several studies - notably those of Huinker (1998, pp. 170-182) and Lappan & Bouck (1998, pp. 183-197) - value the informal approaches students use to solve problems. This exploratory phase, rich in learning, serves as a foundation for the acquisition of more standard methods. Moreover, in his writings, Huinker (1998) emphasizes the importance of context and problem solving for the development of student-generated algorithms. However, he points out that a problem does not have to be very elaborate or even difficult. Rather, it should aim to simultaneously deepen students' sense of the fraction and sense of the operation. For example, it may be a problem for which the students do not know in advance how to do it. They solve it and then analyze their approach, which leads them to recognize the operation performed and to represent the situation symbolically.
Make connections between operations on whole numbers and those on fractions
Students have already developed a solid understanding of addition and subtraction and have some knowledge of multiplication and division. It is important to make connections between whole number operations and fraction operations. For example, adding 3 eighths and 2 eighths is the same as adding 3 candies and 2 candies. Only the notation is different and more complex. Similarly, students who have correct representations of multiplication (for example, \(4\; \times \;5\) can be read as "4 times 5" and represented as \(5\; + \;5\; + \;5\; + \;5\)) will understand the multiplication of a fraction by a whole number (for example, \(4\; \times \frac{1}{8}\) can be read "\(4\; \times \; \frac{1}{8}\)" and represented as \(\frac{1}{8} + \frac{1}{8} + \frac{1}{8} + \frac{1}{8}\)). The underlying concept is therefore the same and it is important to make the connection between the two situations.
Source: translated from Guide d'enseignement efficace des mathématiques de la 4e à la 6e année, Numération et sens du nombre, Fascicule 2, Fractions, p. 74-75.
Multiplication
In the junior grades, students already have a wealth of knowledge about multiplication. Since Grade 1, they have been exploring concepts related to multiplication using concrete materials, the calculator, visuals and symbols. In Grade 4 , multiplication of fractions is limited. This type of multiplication can be understood by linking it to repeated addition. For example, students easily grasp that \(\ 3 \times \frac{1}{2}\), that can be read "3 times one-half" is a multiplication that can be represented by repeated addition, that is, \(\frac{1}{2} + \frac{1}{2} + \frac{1}{2}\). However, it is more difficult to make sense of the multiplication of a whole number by a fraction (for example, \(\frac{1}{2} \times 3\)). There is a connection between the concept of multiplication by a fraction and the concept of a fraction of a set.
Source: translated from Guide d'enseignement efficace des mathématiques de la 4e à la 6e année, Numération et sens du nombre, Fascicule 2, Fractions, p. 78.
In the case of multiplying a fraction by a whole number, it is important to emphasize the meaning of multiplication that can be associated with repeated addition. Consider, for example, the following situation:
How many pieces of cardboard will a student need to give half or a piece to four classmates?
Understanding the situation, students recognize that there is a multiplication of a quantity, either \(\ 4 \times \frac{1}{2}\), which can be represented by repeated addtion, or \(\frac{1}{2} + \frac{1}{2} + \frac{1}{2} + \frac{1}{2}\). To find the answer, students can picture the situation mentally or visually, using their prior knowledge of adding halves. Some students may visualize that two equal parts of the first piece of cardboard are given to two classmates and two equal parts of the second piece of cardboard are given to the other two classmates. Therefore, the student needs two pieces of cardboard.
Others may think of the following abstract representation: "I need 4 times half pieces of cardboard. I know that \(\ 4 \times \frac{1}{2}\) is equal to 2, because two halves are 1. Therefore, I need 2 pieces of cardboard." Others may illustrate the problem as follows, then mentally group the halves two at a time to see that they are equal to two full pieces.

Source: translated from Guide d'enseignement efficace des mathématiques de la 4e à la 6e année, Numération et sens du nombre, Fascicule 2, Fractions, p. 86-87.
Knowledge: Unit Fractions
Fraction with a numerator of 1.
Examples
\(\frac{1}{3}\) and \(\frac{1}{9}\)
Source: translated from Guide d'enseignement efficace des mathématiques de la 4e à la 6e année, Numération et sens du nombre, Fascicule 2, Fractions, p. 35.
Knowledge: Fractional Notation
The fractionas \(\frac{a}{b}\) is written using standard fractional notation. Fractional notation \(\frac{a}{b}\) is usually associated with the concept of a part of a whole. The whole can be an element or a set of elements.
Example
- I gave a fourth (\(\frac{1}{4}\)) of my sandwich to Alex.
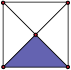
- A fourth (\(\frac{1}{4}\)) of my marbles are blue.

However, fractional notation can also be associated with other concepts such as division, ratio and operator.
Source: translated from Guide d'enseignement efficace des mathématiques de la 4e à la 6e année, Numération et sens du nombre, Fascicule 2, Fractions, p. 36.
Knowledge: Numerator
Number of equal parts of the whole being considered.
Source: translated from Guide d'enseignement efficace des mathématiques de la 4e à la 6e année, Numération et sens du nombre, Fascicule 2, Fractions, p. 34.
