B2.7 Represent the relationship between repeated addition of a unit fraction and multiplication of that unit fraction by a whole number, using tools, drawings, and standard fractional notation.
Activity 1: Multiply a Unit Fraction by a Whole Number
In order to perform a multiplication of a unit fraction by a whole number, students must develop computational strategies using various models. Consider the following situation:
During an activity day, we want the students to experience six different activities, each lasting 15 minutes. How long will all the activities be?
To solve this problem, we can recognize that we can perform the operation \(6 \times \frac{1}{4}\). In order to determine the result, various calculation strategies are possible such as:
- perform the repeated addition;
\(\frac{1}{4} + \frac{1}{4} + \frac{1}{4} + \frac{1}{4} + \frac{1}{4} + \frac{1}{4} = \frac{6}{4}\; so \;1{\frac{2}{4}\; or\; 1\frac{1}{2}}\)
- use a concrete representation;

- use a visual (semi-concrete) representation;
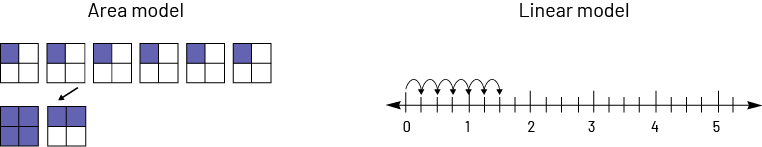
Source: translated from Guide d'enseignement efficace des mathématiques de la 4e à la 6e année, Numération et sens du nombre, Fascicule 2, Fractions, p. 95-96.
Activity 2: How Do You Represent This Statement?
Ask students to represent the following statements using repeated addition and a drawing.
- \(\frac{1}{3}\; \times \;2\)
- \(\frac{1}{5}\; \times \;6\)
- \(\frac{1}{4}\; \times \;8\)
- \(\frac{1}{{10}}\; \times \;5\)
Activity 3: Multiplication
Depending on the context, when you multiply:
- a quantity can be repeated a number of times in order to obtain a new quantity (for example, \(3 \times \frac{1}{2} = \frac{1}{2} + \frac{1}{2} + \frac{1}{2}\));
- we can find a number of "group of" (for example, \(\frac{1}{2} \times 3 = \frac{1}{2}\;{\rm{ group\; of \; }}3\))
Example
In each of the 5 tournaments he has played in, Maurice has won one third of a case of golf balls. How many cases of balls did he win?
Source: Interactive Activity: translated from L'@telier - Ressource pédagogiques en ligne (atelier.on.ca).
