B1.2 Compare and order whole numbers up to and including 100 000, in various contexts.
Skill: Comparing and Ordering Whole Numbers Up to 100 000
Benchmark numbers can be used to compare quantities. For example, 41 320 is less than 50 000 and 62 000 is greater than 50 000, so 41 320 is less than 62 000.
Numbers can be compared by their place value. For example, when comparing 82 150 and 84 150, the greatest place value where the numbers differ is compared. For this example, 2 thousands (from 82 150) and 4 thousands (from 84 150) are compared. Since 4 thousand is greater than 2 thousand, then 84 150 is greater than 82 150.
Numbers can be compared proportionally. For example, 100 000 is 10 times greater than 10 000; it is also 100 times greater than 1000. It would take 1000 hundred dollar bills to make $100 000.
Depending on the context of the problem, numbers can be compared additively or multiplicatively.
Source: Ontario Curriculum, Mathematics Curriculum, Grades 1-8, 2020, Ontario Ministry of Education.
The number line is a model that supports number sense development. A number line does not represent the quantity corresponding to the numbers that are placed on it; but it allows us to visualize numbers in relation to each other. For example, a number line with the numbers 50 001, 50 015, 50 100, and 50 500 on it represents the order relationship between these numbers.
Source: translated from Guide d’enseignement efficace des mathématiques de la 4e à la 6e année, Numération et sens du nombre, Fascicule 1, Nombres naturels, p. 11.

The development of number sense in the junior grades is connected to the recognition of relationships between numbers, including:
- place value relationships;
- order relationships;
- proportionality relationships
Exploring these relationships helps students compare numbers. For example:
If students are asked to find similarities, differences, and connections between the numbers 12, 24, 25, 37, 50, 96, 106, and 120, they may point out:
- that all these numbers except 106 and 120 have two digits, or that 24 and 25 have the same number of tens (a place value relationship);
- that the number 120 is the greatest number, or that 24 is a little less than 25 (an order relationship);
- that 24 is double 12, or that 25 is half of 50 (proportionality relationships).
Order Relationships
The ability to recognize order relationships is acquired by comparing numbers, placing them in ascending and descending order, counting forward/up and backwards/down and analyzing the relative proximity of two numbers.
In the junior grades, students are expected to recognize the order relationship between numbers by comparing them. They can describe the relationship by stating, for example, that 350 is smaller than 432. Examples of strategies to compare large numbers are outlined on the chart below.
| Students can recognize that 23 942 is greater than 12 273: | Example |
|---|---|
| by focusing on the largest of the place values of each number; | Notice that 23 000 is greater than 12 000. |
| by visualizing the important quantities; |
Visualize 23 groups of 1000 and 12 groups of 1000.  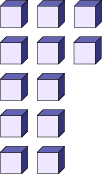 |
| by placing the numbers on a number line; | 
The number 23 942 is located to the right of the number 12 273, which means it is greater. |
| by comparing the digits in the corresponding place value positions starting on the left. | 
The 2 represents 20 000 while the 1 represents 10 000. |
To help students develop the skill of recognizing order relationships among large numbers, teachers may ask them to count up from a certain number by 1 (for example, 12 998, 12 999, 13 000, 13 001…) or by intervals (for example, 32 200, 32 400, 32 600…) and count down by 1 (for example, 26 271, 26 270, 26 269…) or by intervals (for example, 45 650, 45 600, 45 550…).
These activities help students recognize that when counting up by 1s or by intervals, any named number is greater than those before it and less than those after it, whereas when counting down by 1s or intervals, any named number is less than those before it and greater than those after it. While these relationships may seem obvious to adults, students often get them wrong because they do not consider the concept of place value. For example, when asked what number precedes 300, many students tend to spontaneously answer 399 because their attention is focused on the two 0's; they know that a number ending with two 0's is always preceded by a number ending with two 9's, but they forget to take into account the regrouping by hundreds. In contrast, when the same problem is posed in context, students are more likely to give thoughtful answers. For example, in a situation where a student has 300 field hockey cards and loses one of them, the student will easily recognize that they now have 299 cards.
Errors related to the concept of grouping are also common when rounding, where it is necessary to identify a range. For example, some students will say that 12 497 is between 12 490 and 12 400 instead of 12 500. Using a homemade abacus or odometer (created with card stock and the template shown below) helps students compare numbers, count in intervals, and discover changes related to grouping by tens, hundreds…


Once students have mastered the relationships "greater than" and "less than," they need to learn to make these relationships more specific by drawing on their understanding of quantity. They then use expressions such as close to, about, the same as, much more than, and a little less than. For example, students may say that the population of a village of 15 239 is about 15 000; that 304 is a little more than 300; that 12 894 is a little less than 13 000; that 32 523 is about a hundred more than 32 432; and that 620 and 618 are closer together than 630 and 680.
This ability to be more specific when ordering numbers becomes important when students use numbers when problem solving, rounding, estimating and comparing. The following activity provides students the opportunity to demonstrate this skill.
Draw a number line on the board to represent any range (for example, 1000 to 2000). Place some letters (for example, A, B, C, D) in this range as shown below.

Ask a few students to come and locate some numbers on the line (for example, 1873, 1332, 1167). Then ask them to describe the order relationships that exist between the numbers and the letters.
For example, a student might say:
- that 1167 lies between A and B;
- that the letters B and C are between 1167 and 1332;
- that the number 1873 is closer to 2000 than to the letter D;
- that the letter B seems to be more in the centre of the interval between 1000 and 1500 than is the letter C.
Source: translated from Guide d'enseignement efficace des mathématiques de la 4e à la 6e année, Numération et sens du nombre, Fascicule 1, Nombres naturels, p. 43-48.
