B1.5 Read, represent, compare, and order decimal numbers up to hundredths, in various contexts.
Skill: Reading Decimal Numbers to Hundredths
Learning decimal numbers is closely related to understanding decimal notation. Decimal notation is commonly used in the International System of Units (SI) and in the monetary system, among other things. However, despite its frequent use in everyday life and in the classroom, decimal notation is far from being well understood and mastered.
In order to explore learning about decimal numbers, it is important to examine the terminology related to these numbers as well to decimal notation. A decimal number is a number that has a decimal point, such as 3.75. The part before the decimal point represents a whole number amount, and the part after the decimal point represents a value that is less than one. Integers can also be expressed as decimal numbers (for example, 3 = 3.0).
In the junior grades, the study of decimal numbers is linked more specifically to the use of decimal representations to express numbers. In Grade 5, students should understand that a decimal number is composed of two parts, namely the whole number part and the fractional part (including tenths and hundredths).
Example
The number \(8\frac{1}{4}\) is written as 8.25 when represented as a decimal number.
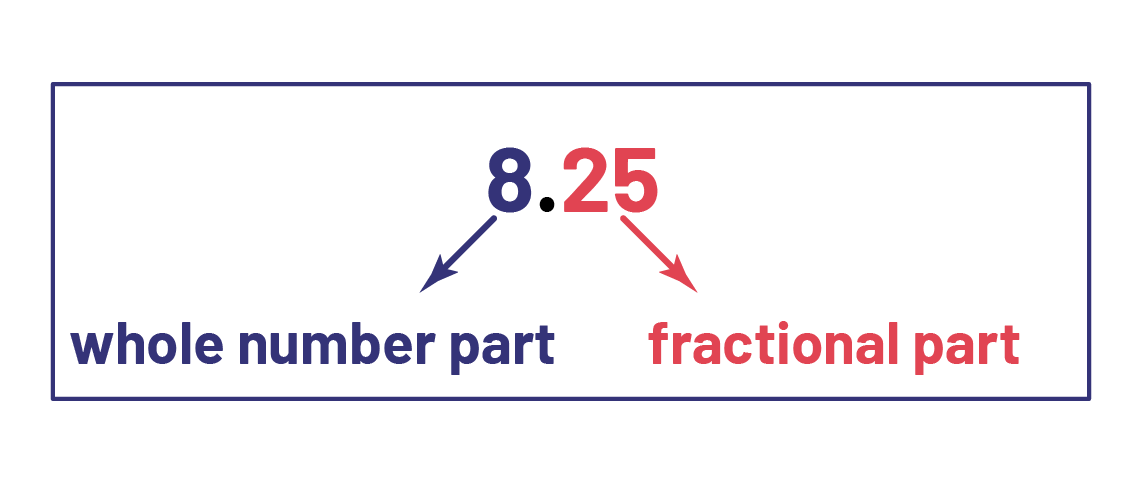
In teaching about decimal numbers and representations, the focus is too often on learning procedures and rules rather than the concepts that support understanding them. This singular focus prevents students from developing conceptual understanding of decimal numbers. Statements such as "you can add 0's after the last decimal place without changing the quantity, for example, undermine understanding the quantity represented by a decimal number. Furthermore, an emphasis on procedures learning to following rules that are, at best, poorly understood. Research emphasizes that effective mathematics instruction should focus on developing an understanding of the concepts, skills and procedures being taught. Decimal numbers are a way of representing numbers with a decimal point. In Grade 5, students will express numbers up to decimal hundredths. In terms of the quantity that a decimal number represents, connections can be made between the decimal part of the number and the concept of a fraction (tenths up to hundredths).
The use of decimal numbers to express a quantity allows greater precision.
Example
Consider a diagonal of a square with sides measuring 1 m. If we try to describe the length of this diagonal using whole numbers, we can only say that it measures 1 m and “part of another metre”.
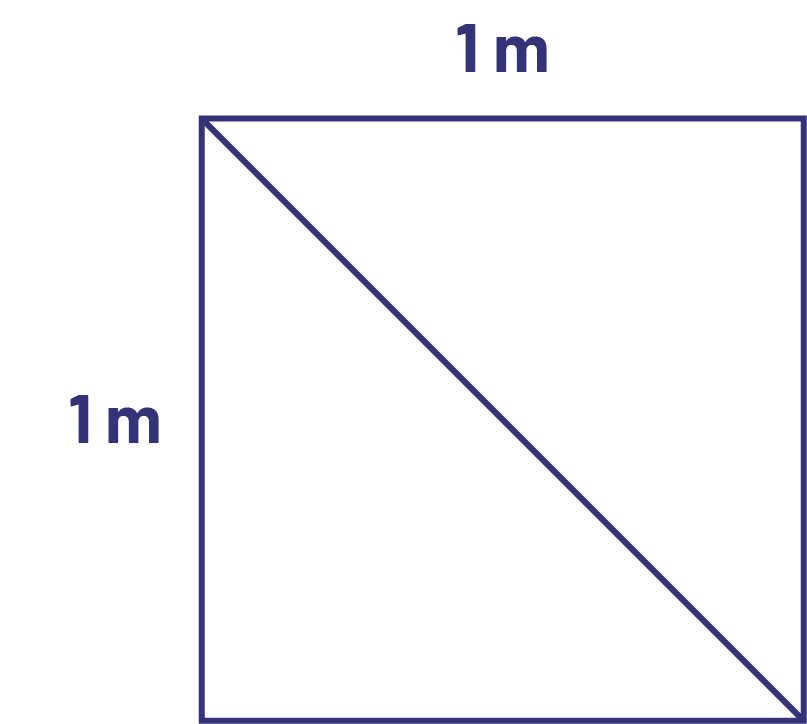
It is possible to provide a more accurate measurements by expressing the length, including the "part of one metre" as a decimal number. Using a ruler graduated in decimetres, we can determine that the diagonal measures about 1.4 m. A measurement of 1.41 m could also be obtained with a ruler graduated in centimetres.
Source: translated from Guide d’enseignement efficace des mathématiques de la 4e à la 6e année, Numération et sens du nombre, Fascicule 3, Nombres décimaux et pourcentages, p. 28-34.
Place Value
The beginning of the junior grades is the first time students study the decimal part of a number. Students will continue to deepen their understanding of the place value and the relationships between place values to the right of the decimal point. Decimal numbers are part of everyday life and understanding the place values to the right and left of the decimal point is essential.
The decimal point plays a significant role when interpreting the value of a decimal number. It separates the whole number part from the fractional part.
However, it is essential that students recognize the position of the ones since it is the ones that defines the whole according to which the tenths, hundredths, tens, hundreds, and thousands are formed. It can therefore be said that the ones, identified by the decimal point, is at the heart of the decimal number.
This recognition of the role of the ones is evidenced by the names given to the place values of the digits on either side of the ones. Thus, tens represent a quantity 10 times greater than one, while tenths represents a quantity that is one tenth of one. Similarly, hundreds are 100 times larger than one, while hundredths are one hundredth of one.
Note: Some students are under the impression that the decimal point is the centre of the decimal number. As a result, they call the first position to the right of the decimal point the "oneths" place rather than tenths place.
It is important that students also grasp the x10 multiplicative relationship that exists between adjacent position values. Students have previously developed an understanding of this relationship in the study of whole numbers, such that each position has a value 10 times greater than the one to its right and 10 times smaller than the one to its left.
This multiplicative relationship is true for all positions.

Students can develop an understanding of this relationship by using base ten blocks. The idea is to demonstrate that just as 10 ones make 1 ten, 10 tenths make 1 one and 10 hundredths make 1 tenth, and so on.
It is common for junior students to write 13 in the tenths column as follows.
0 . 13
However, a decimal number cannot have two digits in one position. Students must recognize that 10 tenths make 1 one, so 13 tenths is equivalent to 1 one and 3 tenths. Therefore, we write 1.3.
Source: translated from Guide d'enseignement efficace des mathématiques de la 4e à la 6e année, Numération et sens du nombre, Fascicule 3, Nombres décimaux et pourcentages, p. 51-53.
Mental Representation
In order to develop a strong sense of number, it is important that students form mental representations of the quantities represented by many different numbers. In the case of decimal numbers, reading them correctly will help students form a better mental representation and draw on their knowledge of fractions (for example, 0.75 is read as "75 hundredths", not "0 point 75"). Students should be encouraged to use multiple models to support the creation of various mental representations.
Example

When representing decimal numbers using previously learned models, there is an adjustment to be made. For example, where the rod was used to represent ten ones when working with whole numbers, it is used to represent 10 tenths when dealing with fractions and decimals. Similarly, the flat was previously used to represent 100 ones and now represents 100 hundredths. Students must understand that the whole has changed and that they must now think about the blocks differently.
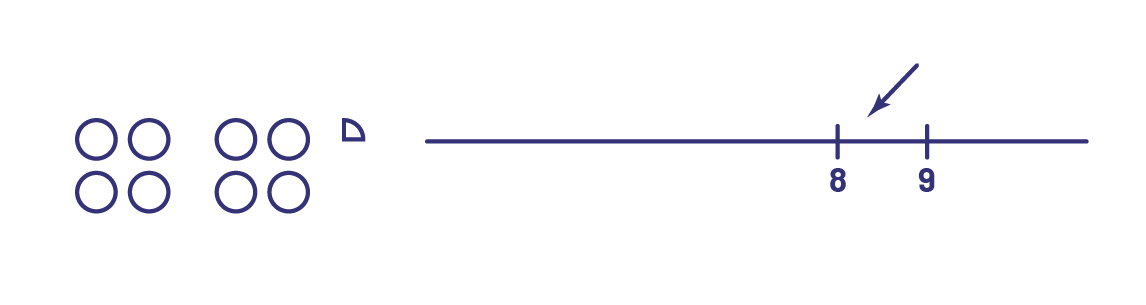
Students must also form a mental representation of decimal numbers greater than 1. When reading such a decimal number, they must imagine the quantity it represents by interpreting each of its two parts: the whole number part and the fractional part. For example, they must recognize that the number 8.24 represents 8 ones and part of another one. They can then visualize a quantity between 8 and 9 that is closer to 8 than 9.
Over time, students are able to mentally picture the quantity taking into account the context. For example, in a situation involving an item that costs $197.98, the student who recognizes that $197.98 is a little less than $200 can then visualize or conceive that in this context, the amount of $197.98 can be represented approximately by 10 $20 bills.
Skill: Representing Decimal Numbers to Hundredths
Students need to learn how to represent decimal numbers in a variety of ways, and recognize them in their multiple representations. These skills help them make connections between numbers, their representations, and the quantities they represent. In some cases, the use of models facilitates the construction of other representations.
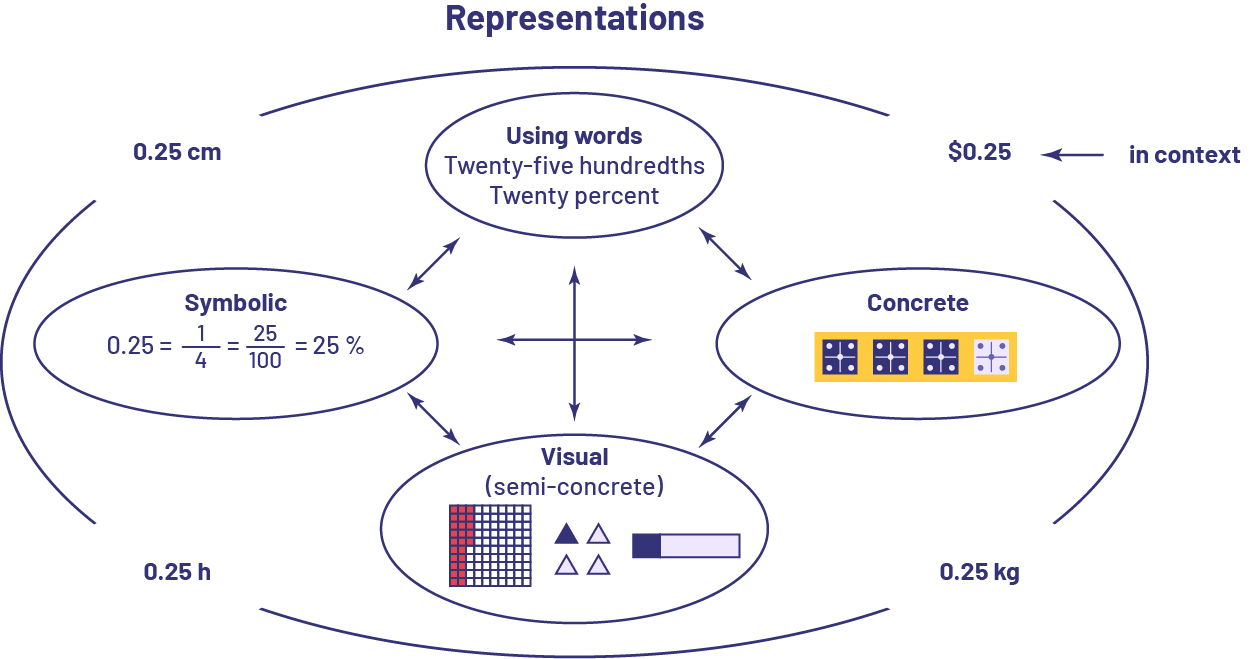
The following figure illustrates various ways of representing 25 cents concretely, visually (semi-concretely), symbolically, and with words. It also demonstrates how 0.25 is used in different contexts. Students develop a better understanding of numbers if they are regularly asked to move from one representation to another in a variety of situations.
Concrete Representations
To fully understand decimal numbers, it is important for students to be able to represent them concretely using manipulatives (for example, counters, base ten blocks). Therefore, in the junior grades, students should have the opportunity to explore decimal numbers using these materials to build conceptual understanding. Before beginning to teach decimal numbers, it is important to have students use manipulatives to show the parts of a whole that are decimal tenths and hundredths (for example, \(\frac{3}{ {10}}\), \(\frac{{12}}{{100}}\)).
Example
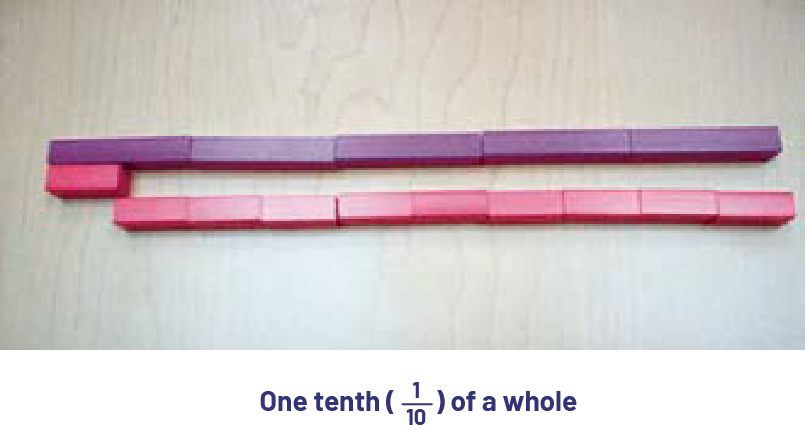
Once students have become accustomed to recognizing and representing tenths and hundredths, they can begin to use concrete materials to represent decimal numbers. Concrete representation of the numbers 2.4 and 0.70, for example, include:


Base ten blocks are excellent tools to represent decimal numbers since they highlight the x10 multiplicative relationship between place values and illustrate the values of digits based on their place. However, it is very important to always clearly define the block that constitutes the whole from which the other blocks will be defined. When studying whole numbers, the small cube was usually associated with ones. However, if we choose the rod as the whole, then the small cube represents one tenth.
Example
The small cube represents 0.1 of a rod.

If we choose the flat as the unit, then the small cube represents one hundredth of the flat and the rod represents one tenth of the flat.
Example
The small cube represents 0.01 of a flat.

The pictures below show different numbers represented by different wholes on a place value mat. In the first picture, 2.39 is represented by 2 flats (ones), 3 rods (tenths), and 9 small cubes (hundredths). In the second picture, 7.3 is represented by 7 rods (ones) and 3 small cubes (tenths).
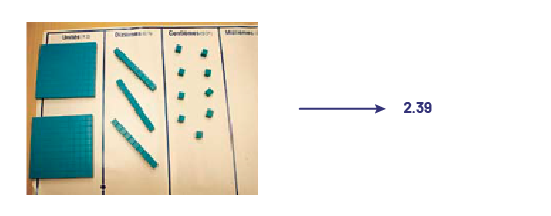
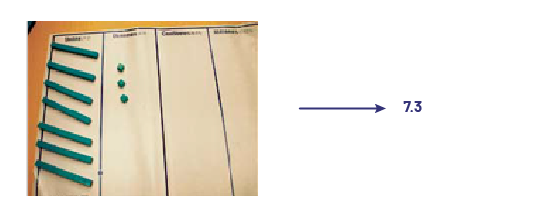
Money can also be used when exploring decimal numbers. However, it is important to use only $1, 10¢, and 1¢ coins since their value respects the x10 multiplicative relationship of the base ten place value system. It is important to note that a representation made with coins is more abstract than one using base ten blocks since the x10 relationship is based on the value of the coin and not its size. Students could identify the $1 coin as the whole (unit). In this case, the 10¢ coin represents one tenth (0.1) of a dollar and 10 dimes are equivalent to one dollar. The 1¢ coin represents one hundredth (0.01) of a dollar and 100 pennies are equivalent to one dollar. Students could also identify the 10¢ coin as the whole (unit). In this case, the 1¢ coin would represent one tenth (0.1) of a dime and 10 pennies are equivalent to one dime.
Visual (Semi-Concrete) Representations
Students can also develop a sense of decimal numbers and percents by representing them in visual ways. For example, a disk divided into 10 equal parts can represent tenths and a disk divided into 100 equal parts can represent hundredths.
Examples
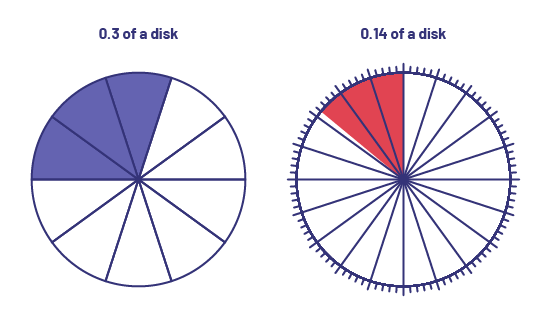
Strips and grids separated into 10 or 100 equal parts can also be used to represent decimal numbers.
Example
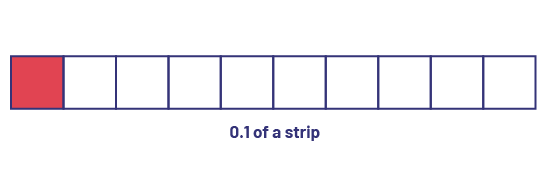
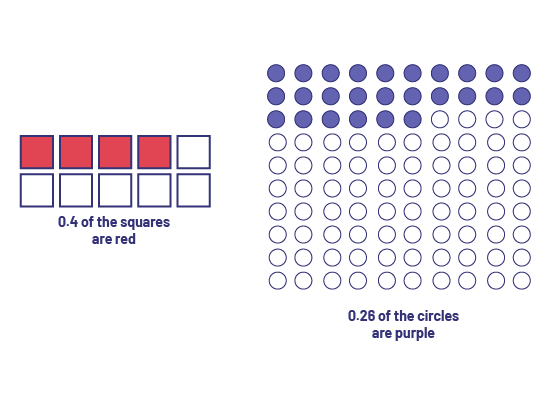
It is also important that students use set models to represent decimal numbers.
Examples
To develop a good sense of number, it is important that students explore different representations of the same quantity. For example, they can be invited to represent 0.50 in several ways on a 10 × 10 grid.
Examples
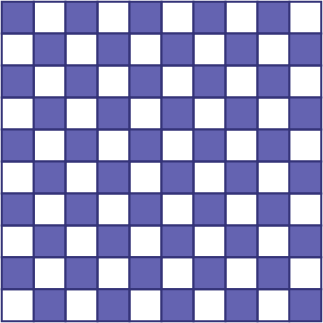
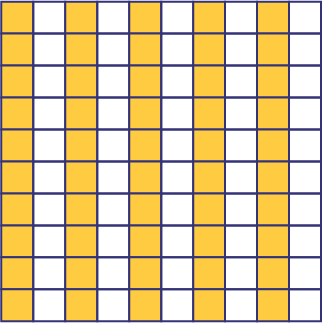
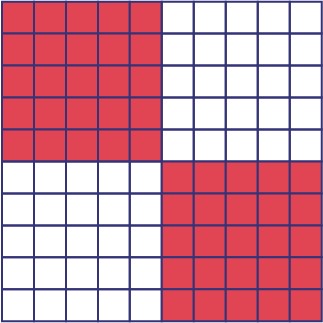
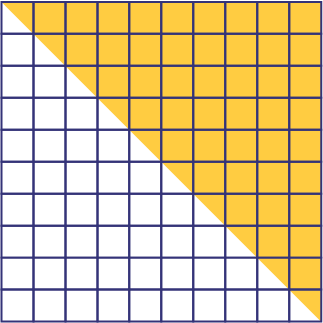
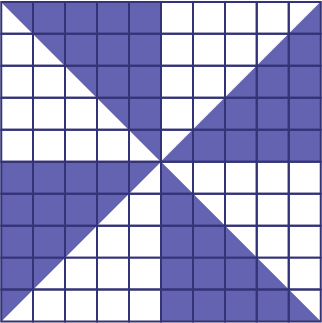
They can also be asked to use different visual representations to represent a given number.
Examples
Three representations of 0.3:
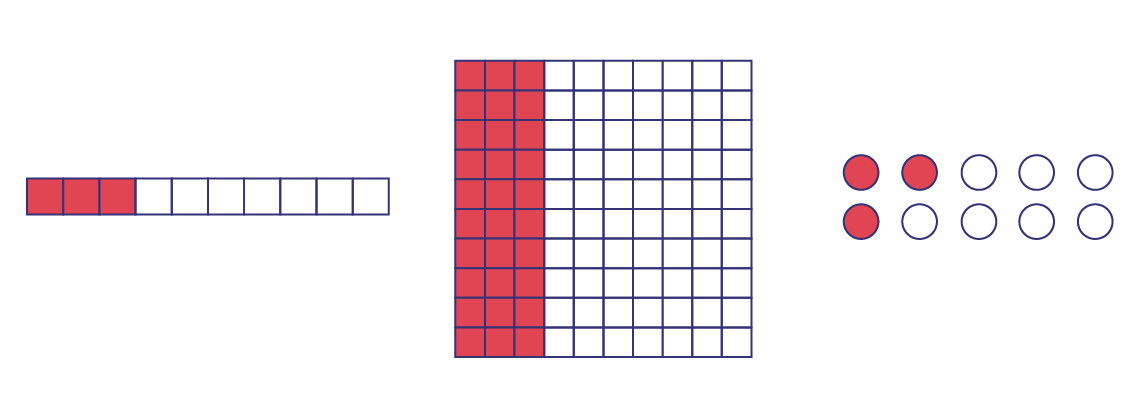
Students have learned to locate whole numbers on a number line. They can similarly use number lines to locate decimal numbers. To do this, students need to understand how to define and partition the intervals used on it.
Number line partitioned into hundredths with intervals of tenths:
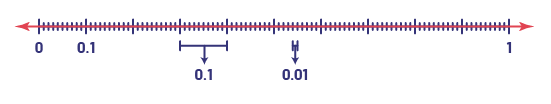
Number line from 5.0 to 5.7 with intervals of tenths:
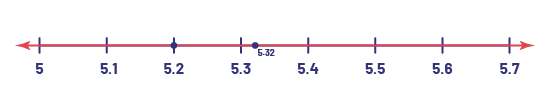
Symbolic Representations
Decimal numbers are a way of expressing numbers with a whole number and a fractional part.
A decimal separated the whole number part from the fractional part.
Example
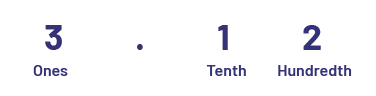
Writing decimal numbers requires an understanding of the concept of regrouping. For example, the number "35 hundredths", can be represented as 3 tenths and 5 hundredths after regrouping with 3 in the tenths position and 5 in the hundredths position, which is 0.35.
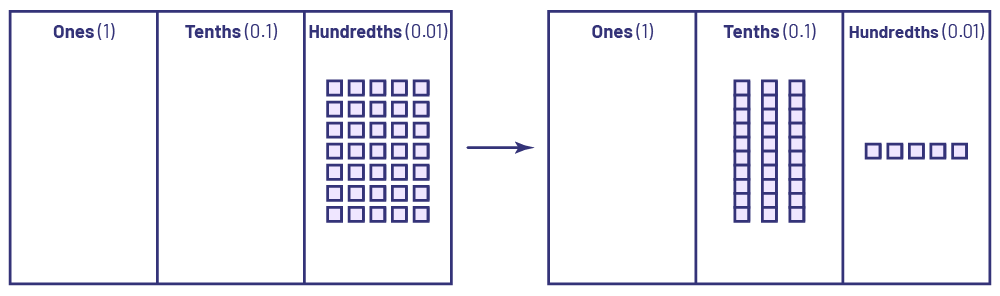
This relationship between writing a decimal number and regrouping can best be understood by exploring decimal numbers using concrete and visual (semi-concrete) representations that allow one to experience the regroupings.
It is important to distinguish between the digit in the hundredths position and the number of hundredths in the number. For example, in the number 0.11, the digit in the hundredths position is 1, but there are 11 hundredths in the number. In the number 1.01, the digit in the hundredths position is also a 1, but this number is 101 hundredths. Thus, if we want students to identify the digit in the tenths position in a given number (for example, 2.35), we should ask the question "What digit is in the tenths position in the number 2.35?" rather than "How many tenths are in the number 2.35?
Representations Using Words
How students learn to read decimal numbers can affect their understanding. If they are taught to read the numbers 0.7 and 0.75 by saying "zero point seven" and "zero point seven five," or "0 point seventy-five," the meaning of the number is left out. This way of reading a decimal number is merely a successive listing of the symbols that make up the number, just as it would be if one were to read the number 123 by saying "1, 2, 3." However, insisting on reading these numbers as "7 tenths" and "75 hundredths" emphasizes the meaning of the representation of the number. This way of reading decimal numbers gives students the opportunity to visualize the 7 parts of 10 and the 75 parts of 100 and has the added advantage of reminding them of the corresponding decimal fractions.
It is important that teachers model this way of reading decimal numbers at all times. The numbers 12.34 and 1013.7 are read as "twelve and thirty-four hundredths" and "one thousand thirteen and 7 tenths" respectively. Note that when reading a decimal number, we read the whole number part; then the word "and" (not "point"), which serves as a link between the two parts; and the fractional part is read according to the place value of the rightmost digit in the number.
Note: Some students may confuse the terms ten and tenth or hundred and hundredth when reading because of their phonetic similarity.
Source: translated from Guide d'enseignement efficace des mathématiques de la 4e à la 6e année, Numération et sens du nombre, Fascicule 3, Nombres décimaux et pourcentages, p. 57-69.
Skill: Comparing and Ordering Decimal Numbers to Hundredths
The order relationship is based on the comparison of numbers. One of the great strengths of decimal numbers is the speed with which it is possible, through the concept of place values, to compare and order quantities. For example, it is much easier to compare the numbers 0.64 and 0.75 when expressed in decimal notation rather than as the fractions \(\frac{{16}}{{25}}\) and \(\frac{{15}}{{20}}\).
In general, students have little difficulty comparing decimal numbers with the same number of decimal places (for example, 0.34 < 0.46). They have more difficulty comparing numbers with a different number of decimal places (for example, 1.3 and 1.27). Some tend to compare these numbers without the decimal point (for example, 13 < 127) and conclude that 1.3 < 1.27. Others reach the same incorrect conclusion by comparing only the numbers to the right of the decimal point (for example, 3 < 27).
The order relationship should be addressed by comparing decimal numbers in contextualized situations. For example: "Remi did a jump of 3.55 m and Samantha did one of 3.7 m. Which of the two had the longer jump?" Students can respond and justify their choice if they understand place value. The number line is a powerful visual model for comparing decimal numbers. To place 3.7 on a number line, students can represent tenths from 3.0 to 4.0. To place 3.55, they must partition the interval between 3.5 and 3.6 into tenths, with each space representing one hundredth. They can then conclude that 3.55 < 3.7, so Samantha has taken a longer jump than Remi.
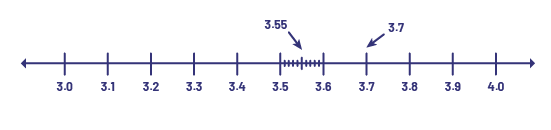
Students with good number sense can also compare 3.55 m and 3.7 m by first noticing that they each represent jumps greater than 3 m. Then, they can compare tenths to notice that the first number has 5 tenths (representing 5 decimetres), while the second has 7 tenths.
Samantha's jump is therefore longer than Remi's. Students can also, after comparing units, think of 3.7 as 3.70, or 3 metres and 70 centimetres. The number 3.55 represents 3 metres and 55 centimetres. The 3.7 m jump is therefore longer than the 3.55 m jump.
Traditionally, a procedure was taught whereby one was instructed to add a 0 to the end of 3.7 to result in two numbers with the same number of decimal places. The decimal parts, 55 and 70, were then compared to conclude that 3.70 was greater than 3.55. Although the teaching of this method might have been accompanied by an explanation, so much emphasis was placed on the method that the explanation and the concept were not paramount. Not surprisingly, students often answered such questions incorrectly. For example, in an international test of Grade 6 students, 87% reported that 6.987 is greater than 6.879, while only 52% concluded that 1.05 is greater than 1.015 (Brissiaud, 1998). The finding illustrates that it is important that students understand the concept of place value and do not need to rely on a procedure that may let them down when comparing decimal numbers.
Open problems, which offer more than one answer and are thought-provoking, allow students to deepen their understanding of ordering decimal numbers.
For example:
- Determine three decimal numbers between 0.55 and 0.62.
- Determine three numbers within one tenth of 2.8.
Source: translated from Guide d'enseignement efficace des mathématiques de la 4e à la 6e année, Numération et sens du nombre, Fascicule 3, Nombres décimaux et pourcentages, p. 54-55.
Skill: Reading, Representing, Comparing and Ordering Decimal Numbers in Various Contexts
Context includes all the information surrounding a given situation. To deeply understand the meaning of the quantity represented by a decimal number, students must analyze it in its context. Like a fraction, a decimal number includes a fractional part of the whole. The magnitude of the quantity represented by a decimal number is therefore entirely dependent on the magnitude of the whole.
A context may help to make the mental image of a quantity more clear. For example, if 2.34 is mentioned, students understand that this number represents 2 (the whole) and \(\frac{{34}}{{100}}\). But, if the amount is stated as 2.34 m, it is easier to form an image of the quantity in context.
However, in some situations, it is important to recognize that decimal numbers may not be referencing the same whole.
Example
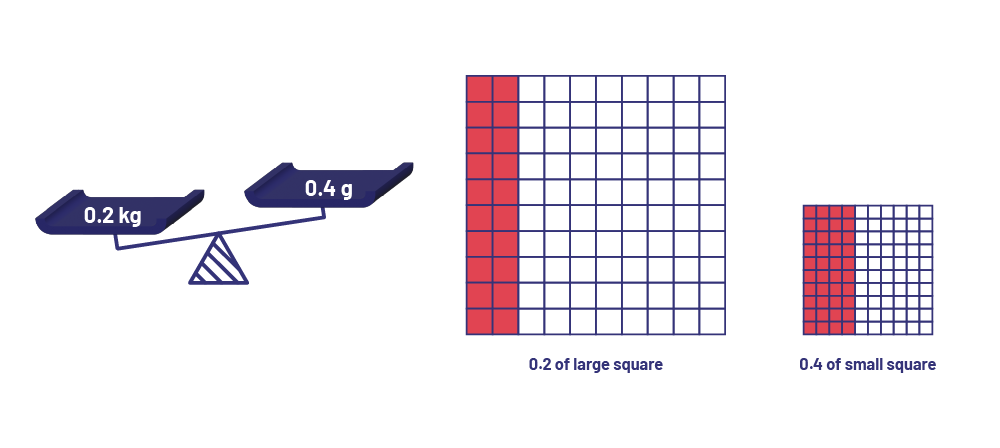
In these examples, even though the number 0.2 is less than the number 0.4 (0.2 < 0.4), 0.2 kg is a larger mass than 0.4 g, just as 0.2 of the large square is a larger area than 0.4 of the small square.
Furthermore, without context, it is sometimes impossible to make a critical judgment about the size of the quantity represented by a decimal number. For example, a child's purchase of a toy car for $8.34 may be considered expensive by their parents, while the child may find it a bargain for a collectible car. The more students are exposed to different mathematical situations and context, the more they grow their knowledge and their ability to make informed critical judgments.
Headlines often provide topics for discussion that can help students understand and interpret decimal numbers.
Example
- A decrease of $13.7 thousand in the municipal budget.
- New world record, an apple of 13.45 kg!
During a class discussion, teachers can ask questions that help students analyze the headlines given above.
Example
- Is it important to specify the 0.45 kg in the mass of the apple? Why or why not?
- At first glance, $13.7 thousand seems like a lot of money, but is it a significant amount of the budget?
- How can a number like 13.7 represent such a large amount of money?
Source: translated from Guide d'enseignement efficace des mathématiques de la 4e à la 6e année, Numération et sens du nombre, Fascicule 3, Nombres décimaux et pourcentages, p. 40-41.
Knowledge: Decimal Number
Decimal numbers are representations of numbers that have a decimal point (for example, 3.72; 0; 12.13564). A decimal number can be a terminating decimal number (for example, 0.45), a repeating non-terminating decimal number (for example, 0.333…) or a non-repeating non-terminating decimal number (for example, 3.14159…). Students in Grade 5 will work with terminating decimal numbers.
Examples
\(3.72\; = \;3\frac{{72}}{{100}}\; = \;\frac{{372}}{{100}}\)
\(5\; = \;5.0\; = \;\frac{5}{1}\)
 Note: A decimal number can be a terminating decimal number (for example, 0.45), a repeating non-terminating decimal number (for example, 0.333…) or a non-repeating non-terminating decimal number (for example, 3.14159…).
Note: A decimal number can be a terminating decimal number (for example, 0.45), a repeating non-terminating decimal number (for example, 0.333…) or a non-repeating non-terminating decimal number (for example, 3.14159…).
Source: translated from Guide d’enseignement efficace des mathématiques de la 4e à la 6e année, Numération et sens du nombre, Fascicule 2, Fractions, p. 42.
Knowledge: Hundredths
The decimal point plays a significant role when interpreting the value of a decimal number. It separates the whole number part from the fractional part.
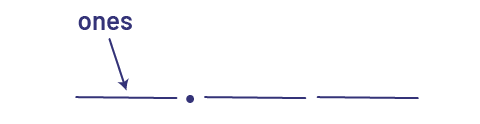
The position of the ones defines the whole according to which the tenths, hundredths, tens, hundreds, and thousands are formed. It can therefore be said that the ones, identified by the decimal point, is at the heart of the decimal number.
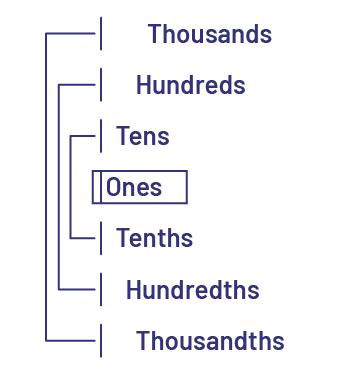
The importance of the ones is evidenced by the name prefixes given to the place value of the digits on either side. For example, tenths are one tenth as large as one, and hundredths are one hundredth as large as one.
Source: translated from Guide d’enseignement efficace des mathématiques de la 4e à la 6e année, Numération et sens du nombre, Fascicule 3, Nombres décimaux et pourcentages, p. 51.
