E1.2 Plot and read coordinates in the first quadrant of a Cartesian plane, and describe the translations that move a point from one coordinate to another.
Activity 1: Slide, Slide
Describe how to get from one point to another on the Cartesian plane.
Instructions
Present the first quadrant of the Cartesian plane on a whiteboard.
Draw a blue triangle whose vertices are (3,4) , (7, 2) and (8,6).
Draw the outline of a translated triangle in red and ask students to identify its new coordinates.
Explain that:
- the triangle drawn in blue is the initial shape and that the triangle drawn in red is the translated triangle and is also referred to as the image;
- this kind of movement is called a translation and that, in a translation, iall the coordinates of the triangle slide.
Have teams of two students perform other translations to the blue triangle that are left, up, or down, and ask students to identify the coordinates each time.
Note: Have students limit their moves so they stay within the boundaries defined by the vertical and horizontal axes. Also, different strategies can be used to assess understanding of the concept of translations. For example:
- draw the initial shape and give the movement; ask to represent the image;
- draw the image and give the movement; ask to represent the initial shape;
- draw the initial shape and the image; ask to describe the movement.
Source: translated from Guide d’enseignement efficace des mathématiques de la 1re à la 3e année, Géométrie et sens de l'espace, p. 68-69.
Activity 2: Tic-Tac-Toe, Let’s Have Fun!
Goal
This activity integrates concepts from the Spatial Sense and Algebra strands. The teacher asks students to pair up and gives each student a copy of the first quadrant of a Cartesian plane to play a game similar to tic-tac-toe. The teacher explains the rules of the game.
Rules of the game- Each student chooses tokens of the same colour.
- In turn, a student places one of their tokens on a point on the Cartesian plane, giving the coordinates of the point aloud.
- Their opponent checks if the token is correctly located according to the given coordinates. If it is not, it must be removed.
- The first person to place tokens on four successive points to form a straight horizontal, vertical, or diagonal line wins.
When the game is over, the teacher asks students to record the coordinates of the four points corresponding to the aligned tokens in a table of values and note the patterns. In the example below, students could note that for every 1 increase in the value of x, there is a 1 decrease in the value of y.
Example
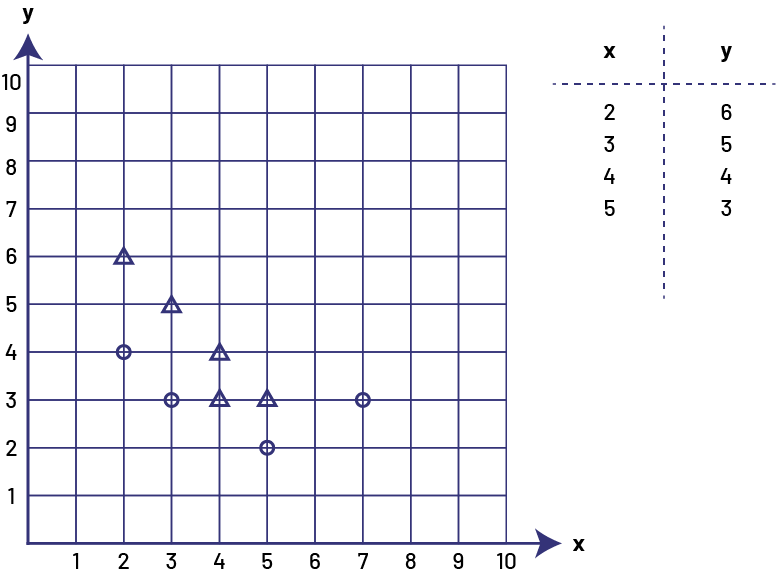image A grid with ten columns and ten rows. The columns are numbered on the horizontal axis, "x", and the rows on the vertical axis, "y". Triangles are placed at the intersections of "x" two and "y" six, "x" three and "y" five, "x" four and "y" four, "x" five and "y" three, "x" four and "y" three. Circles are placed at the intersections of "x" four and "y" two, "x" three and "y" three, "x" two and "y" five, and "y" three and "x" seven. To the right of the grid is a table with two columns, "x" and "y". Under the column "x" are the numbers two, three, four, and five; under the column "y". Are the numbers six, five, four, and three.
Source: translated from Guide d'enseignement efficace des mathématiques de la 4e à la 6e année, Géométrie et sens de l'espace, Fascicule 2, p. 42.
Activity 3: Looking for Kiki the Mouse!
Provide each student with a copy of the Looking for Kiki the Mouse! game (Appendix 4.3). Form teams of two students and have them place a barrier (for example, ring binder, book) on the desk between their copies of the game. Explain the rules of the game.
Rules of the Game
- Each person draws an X in one of the grid squares at the top of their sheet (My mouse) to show where their mouse, Kiki, is hiding.
- To determine who starts the game, the partners can play “rock, paper, scissors”.
- The starting partner names the coordinates of the square in which they think their opponent's mouse is located (for example, F 5). If this is not the correct square, their opponent will give feedback using cardinal points (for example, “Kiki is hidden northwest of that square”).
- The partner who named the coordinates then draws an X in the appropriate square on the grid at the bottom of their sheet (My opponent's mouse) so as to keep track of their guesses. They can also use the table to the right of the grid to record their guesses as well as the clues received from the opponent.
- Then the second person follows the same steps to try to locate the opponent's mouse.
- The game continues in this way until one of the pair finds the square in which their opponent's mouse is located.