E1.5 Describe and perform translations, reflections, and rotations up to 180° on a grid, and predict the results of these transformations.
Skill: Describing and Performing Translations
Students can describe and perform horizontal, vertical, or oblique translations indicated with an arrow. The arrow can be placed on the figure or outside the figure. The direction of the arrow corresponds to the direction of the translation and its length corresponds to the magnitude of the displacement.
To describe and perform the translation, students must determine the magnitude of the horizontal and vertical movements represented by the arrow. For example, the arrow below defines the translation (4 left and 2 up), that is, a movement of four units left and two units up.
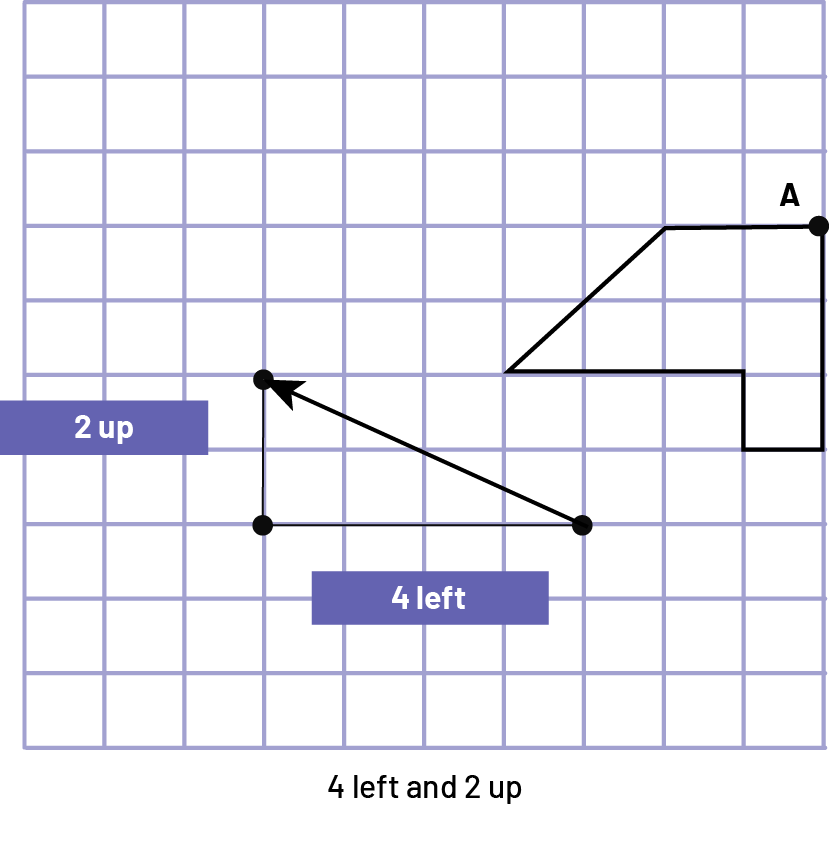image A grid of ten columns and ten rows. On the right side of the grid, there is a figure. Only the point "a" is identified. There is an oblique arrow on the diagonal of the figure, pointing to the left. The arrow forms a right triangle. To the left of the triangle is marked: two units up. Below the triangle is marked: four units to the left. Under the grid is written: (open parenthesis), four "g", two "h", (close parenthesis).
During a translation, all the points of the initial shape undergo the same movement, that is to say that all the points of the initial shape are moved in the same direction and are equidistant from the corresponding points of its image.
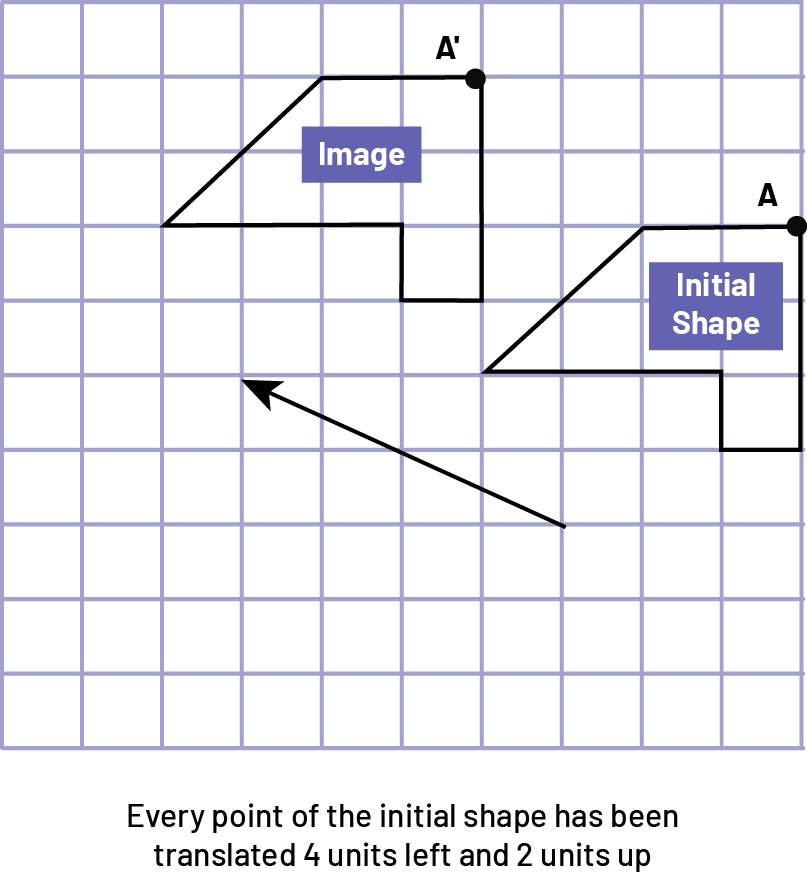image A grid of ten columns and ten rows. There are two figures placed obliquely on the grid. In the first one is written initial figure; in the second one is written image. The point "a" is identified on the initial figure, and the point "a" apostrophe is identified on the image. There is an oblique arrow at the diagonal of the figures, pointing to the left. Under the grid is marked: Each point of the initial figure has moved four units to the left and two units up.
Source: translated from Guide d'enseignement efficace des mathématiques de la 4e à la 6e année, Géométrie et sens de l'espace, Fascicule 2, p. 29-30.
Skill: Describing and Performing Reflections
To describe and perform a reflection, it is necessary to understand that all points in the original shape and corresponding points in its image are at equal distances from the line of reflection. The reflection results in a change in the original position and orientation of a shape, and the reflected image is congruent with the initial shape. In other words, the reflected image has the same size and shape, but it will be oriented in a different direction, and will be in a different position. It is important to note that the line of reflection can be drawn in any direction relative to the original shape - horizontally, vertically or diagonally, at any angle.
In this example, points A, B and C are exactly the same distance from the line of reflection as points A', B' and C'.
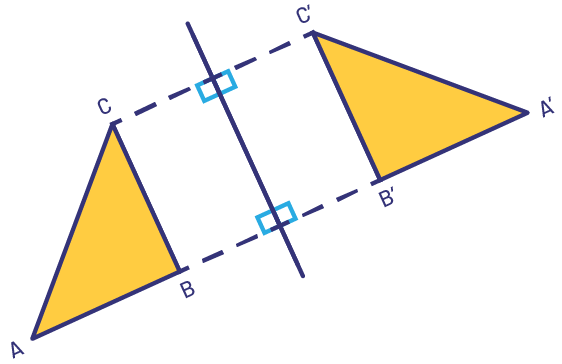image A quadrilateral formed by a rectangle and two congruent triangles of opposite sides. An axis of reflection passes through the center of the figure. On one side, the points "a", "b", and "c" are identified; on the other side, the points "a" apostrophe, "b" apostrophe, and "c" apostrophe are identified.
Source: A Guide to Effective Teaching of Mathematics in Grades 4 to 6, p. 35.
Students perform reflections of simple and complex shapes (for example, to create friezes and tessellations) on dot paper or grid paper, using a Mira, tracing paper, or dynamic geometry software or application.
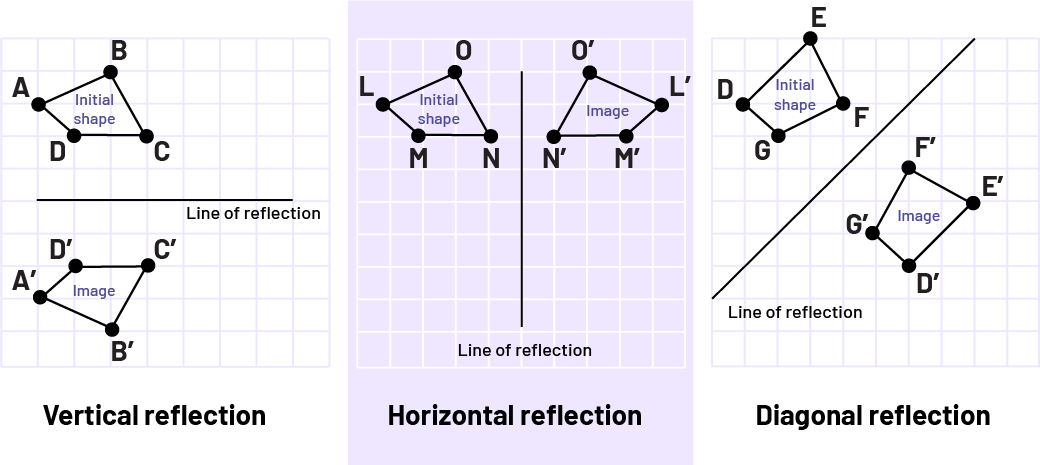image There are three grids: the first represents a vertical reflection, the second represents a horizontal reflection, and the third represents an oblique reflection. In the first grid, two congruent quadrilaterals are on either side of a horizontal line, named the axis of reflection. The first quadrilateral is the initial figure; the second quadrilateral is the image.In the second grid, two congruent quadrilaterals are on one side each of a vertical line, labeled the axis of reflection. The first quadrilateral is the initial figure; the second quadrilateral is the image. In the third grid, two congruent quadrilaterals are on one side each of an oblique line, labeled the axis of reflection. The first quadrilateral is the initial figure; the second quadrilateral is the image.
Source: translated from Guide d'enseignement efficace des mathématiques de la 4e à la 6e année, Géométrie et sens de l'espace, Fascicule 2, p. 31.
Skill: Describing and Performing Rotation of up to 180° on a Grid
To describe and perform a rotation, one must be able to understand and state:
- the location of the point of rotation around which a shape rotates (for example, the point of rotation can be a point on the outline of the shape, inside the shape or outside the shape);
- the measure of rotation (for example, a quarter turn or 90°, or a half turn or 180°);
- the direction of rotation (for example clockwise, counterclockwise).
Students need to understand what a quarter, half, and three-quarter turn clockwise or counterclockwise represents. It is important to introduce them to a variety of concrete activities that will help them develop this understanding.
Using the clock as a model is an effective strategy to help students develop a sense of how a full revolution can be represented using fractions. For examples, students could use a cardboard clock made with two hands attached using a brass fastener. Ask students to place both hands at 12. Then, to represent a rotation of one quarter-turn clockwise, ask students to move the minute hand to 3. The hour hand represents the initial position while the minute hand represents the position after the rotation.
Students should be supported to make the connection between the fraction of the rotation represented by the hands of the clock, the number of minutes between the two hands and as well as the number of degrees between them.
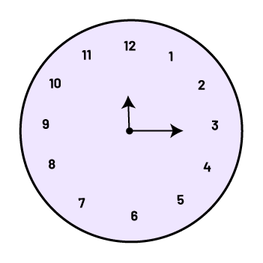
\(15 \ minutes = \frac{1}{4} \ rotation = 90^{\circ}\)
Note: On a real clock, this correspondence is not quite accurate, since the hour hand moves slightly when the minute hand rotates a quarter rotation.
Again, it is important to vary the initial direction of the hands. For example, the teacher can place the two hands of the clock at 2 and have the students rotate them clockwise one-half turn. The students can then use the fact that one-half turn of the large hand corresponds to 180° or 30 minutes to determine that the minute hand should be placed at 8.
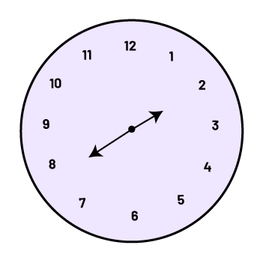
\(30 \ minutes = \frac{1}{2} \ rotation = 180^{\circ}\)
Once students have developed their understanding of fractions of a rotation using concrete materials, they are able to represent them on circles drawn on paper.
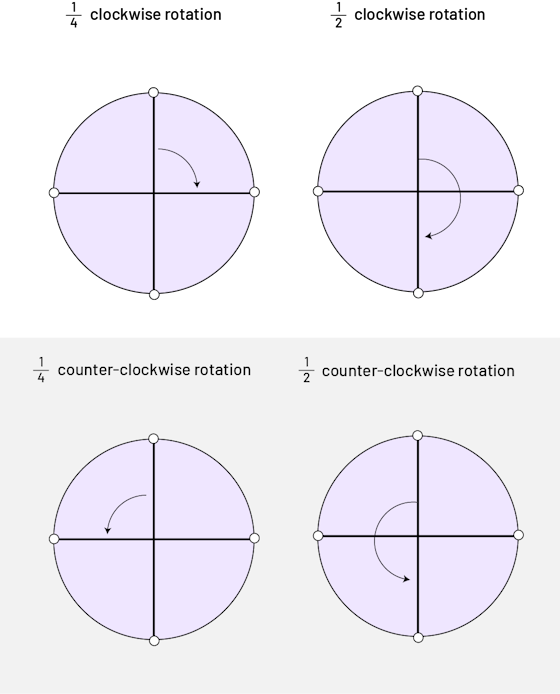image There are four circles, each divided into quarters. Above the first circle is labeled: one quarter turn clockwise. There is a rounded down arrow in the first quarter. Above the second circle is labeled: one half turn clockwise. There is a rounded arrow passing through the first and second quarter. Above the third circle is labeled: a quarter turn counterclockwise. There is a downward rounded arrow in the fourth quarter. Above the fourth circle is labeled: one half turn counterclockwise. There is a rounded arrow passing through the fourth and third quarter.
Students should perform rotations of shapes using one of the vertices of the shape as the point of rotation and a variety of tools (for example, tracing paper, polar coordinate graph paper, square grid paper, dynamic geometry software or application). Performing the rotation according to the specified fraction will support students to better understand the impact of this transformation. Students should also perform rotations with the point of rotation on the perimeter of the shape or inside of the shape, and also with the point of rotation outside the shape.
Example
A quarter turn clockwise rotation, with the center of rotation located:
- on a vertex of the shape;
- on the perimeter of the shape or inside the shape;
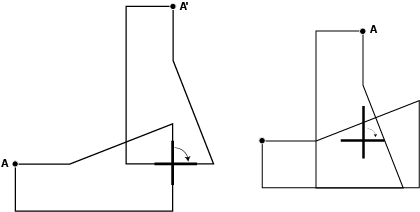image There are two examples of overlapping patterns. In the first example, the first figure is horizontal and is marked with a point "a". The second figure is vertical and is marked with an apostrophe "a" point. The point where the figures intersect is marked with a 90 degree rotation point, in which an arrow curves downward into the first quadrant. In the second example, the first figure is horizontal and is marked with a point "a". The second figure is vertical within the first figure and is marked with an apostrophe "a" point. The point where the figures intersect is marked with a 90 degree rotation point, in which an arrow curves downward into the first quadrant.
- outside the shape;
image There are two figures. The first figure is horizontal and is marked with a point "a". The second figure is vertical and is marked with an apostrophe "a" point. The point where the figures intersect is marked with a 90 degree rotation point, in which an arrow curves downward into the first quadrant.
Source: translated from Guide d'enseignement efficace des mathématiques de la 4e à la 6e année, Géométrie et sens de l'espace, Fascicule 2, p. 32-35.
Skill: Predicting the Results of Transformations
In the junior grades, students predict the outcome of a transformation and describe what will happen to the object when the transformation is completed. Through guided investigations, students will eventually be able to observe the initial orientation of an object and the outcome of a transformation, and describe the transformation that has been completed without having to see it.
Source: translated from Guide d'enseignement efficace des mathématiques de la 4e à la 6e année, Géométrie et sens de l'espace, Fascicule 2, p. 34.
Knowledge: Transformations
Transformations contribute to the development of a spatial sense and the ability to visualize the movement of objects in two- and three-dimensional space. The movement of geometric shapes can be described using various transformations, such as translation, reflection, and rotation.
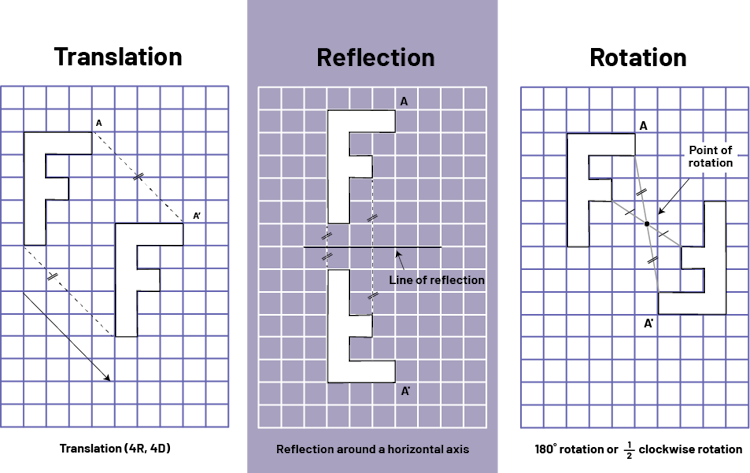image There are three grids. The first represents a transfer, the second represents a reflection, and the third represents a rotation. In the first grid, there are two letters "f" with the points "a" of the first figure "f" and the apostrophe "a" of the second figure "f" connected by a dotted line. There is a second dotted line of the same length at the base of the figure. Under the figures there is an arrow pointing to the right. Under the grid it is written: transfer ( open parenthesis), four "d", four "b", (close parenthesis). In the second grid there are two figures "f" connected by two dotted lines of different length. The figures are on one side each of a horizontal line, the axis of reflection. Under the grid is written: Reflection with respect to a horizontal axis. In the third grid there are two figures "f", one of which is inverted, linked by two dotted lines of different length that intersect at a center of rotation. Under the grid is written: 180 degree rotation or half a turn clockwise.
Source: translated from Guide d’enseignement efficace des mathématiques de la 4e à la 6e année, Géométrie et sens de l'espace, Fascicule 2, p. 37.
Knowledge: Translation
A translation is defined by its size and direction (represented symbolically by coordinates or by an arrow). It represents a linear, horizontal, vertical or oblique (diagonal) movement. The initial shape and its image are congruent. The distance is constant between each point of the initial shape and each corresponding point of its image. The orientation of the image is the same as the orientation of the initial shape.
Source: translated from Guide d'enseignement efficace des mathématiques de la 4e à la 6e année, Géométrie et sens de l'espace, Fascicule 2, p. 36.
Knowledge: Reflection
A reflection is a flip performed over a line of reflection. Each point on the initial shape and the corresponding points on the image are at the same distance from the line of reflection. The initial shape and its image are congruent, but the orientation of the image is different from the orientation of the initial shape.
Source: translated from Guide d’enseignement efficace des mathématiques, de la 4e à la 6e année, Géométrie et sens de l'espace, Fascicule 2, p. 36.
Knowledge: Rotation
A rotation is a transformation that moves each point of a shape around a fixed point, also called the point or centre of rotation. A rotation creates an image that is congruent with the original shape. However, the orientation of the image is different from the orientation of the initial shape.
The point of rotation can be anywhere on the plane, either outside the figure or inside.
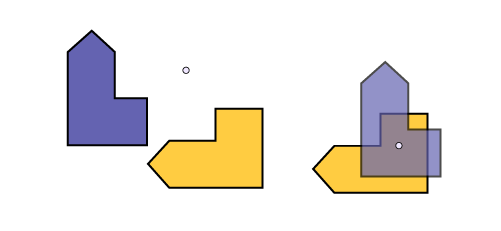
When the point of rotation is on a vertex of the initial shape, the initial shape and its image will share this point.
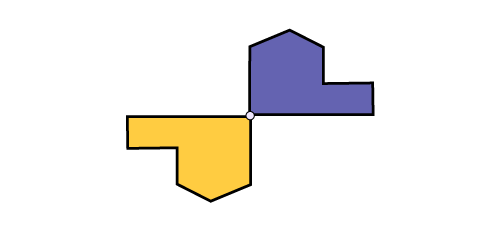
Source: A Guide to Effective Teaching of Mathematics in Grades 4 to 6, p. 36.