E1.2 Construct three-dimensional objects when given their top, front, and side views.
Activity 1: Objects and Their Shape
This activity integrates concepts from the Spatial Sense strand with science and technology concepts.
To promote the development of scientific inquiry and design skills, the teacher suggests to students that they analyze a common object with an emphasis on properties related to its shape. For example, the teacher may ask students to:
- picture any object that has a different shape than the traditional one and discuss its advantages and disadvantages (for example, a door that is not rectangular, a balloon that is not round, a roof that is not triangular);
- identify the various shapes that an object takes and highlight the advantages and disadvantages of each (for example, various shapes of light bulbs).
A variety of objects can be studied with particular attention to shape (for example, airplane, rocket, parachute, hot air balloon, flying disc, kite, hourglass). The teacher forms teams of two students. Each team must research (for example, library, Internet) and construct a prototype of an object using a non-traditional geometric shape. Students must then present their object to the class and point out its advantages and disadvantages, particularly those related to shape (for example, malleability, resonance, strength, capacity, aerodynamics, aesthetics). However, when constructing the prototype, it is important that students do not focus solely on the aesthetic aspect of the object.
Source: translated from Guide d’enseignement efficace des mathématiques, de la 4e à la 6e année, Géométrie et sens de l'espace, Fascicule 1, p. 44-45.
Activity 2: Mysterious Ruins
Distribute the following views of a monument to students.
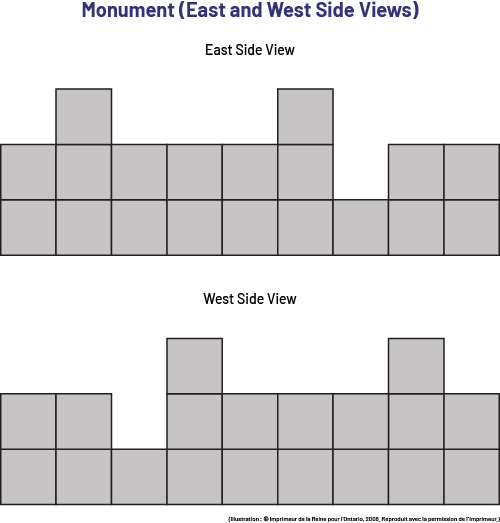Image the view of a monument, from two different perspectives represented in squares. Above the first figure is written: East side view. Above the second figure is written: West side view. Above is written: monument (opening parenthesis) east and west side views (closing parenthesis). 
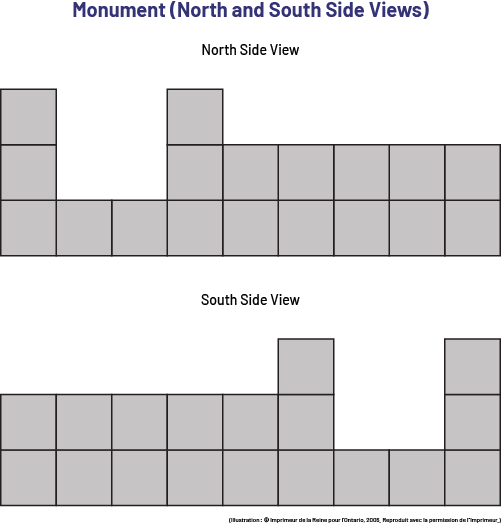Image the view of a monument, from two different perspectives represented in squares. Above the first figure is written: North side view. Above the second figure is written: South side view. Above is written: monument (opening parenthesis) North and South side views (closing parenthesis).
Image there is a photo of an ancient monument in ruins. To the left is written: On this archaeological site, a team of researchers are renovating the ruins of a very old monument. In order for the archaeologists to be able to reconstitute the monument, one of the researchers has traced the views of the four sides (opening parenthesis) east, west, north and south (closing parenthesis).
The archaeologists want to use interlocking cubes to make a model of the ruins according to the four views that the excavator drew. They realize that there are several possible arrangements of the cubes that respect the views.
How can you build a model of one of these layouts using interlocking cubes? How can you build one with as few cubes as possible?
Skills to be developed related to spatial visualization :
- Oculomotor coordination
- Perception of positions
- Perception of spatial relationships
Source: translated from Guide d’enseignement efficace des mathématiques, de la 7e à la 10e année, Mesure et géométrie, Fascicule 3, p. 15.
Activity 3: Three Views Are Better than Two
Form teams of two students. Have them build a model of some sort with a given number of interlocking cubes (for example, 15 cubes) and draw the front, side, and top views of that model. Then group the teams in pairs and identify them (for example, Teams A and B). Give the following explanations:
Team A first gives Team B two views of their model, the top view and the side or front view. Team B must build a model that respects these views while using all of the cubes. Team A then gives Team B the third view and asks them to see if they need to change the placement of any of the cubes with this new information. Team B then compares their final model with Team A's and the students discuss the similarities and differences. Team A then tries to replicate Team B's model by following the same process.
Note: Even with three views, it can be possible to build two models that are not perfectly identical.
Source: translated from Guide d’enseignement efficace des mathématiques, de la 4e à la 6e année, Géométrie et sens de l'espace, Fascicule 1, p. 92.