E1.2 Draw top, front, and side views, as well as perspective views, of objects and physical spaces, using appropriate scales.
Activity 1: Three Views Are Better than Two
Form teams of two. Ask students to build any model with a given number of interlocking cubes (for example, 15 cubes) and draw the front, side and top views of this model. Then group the teams in pairs and name them (for example, teams A and B). Give the following explanations.
Team A first gives team B two views of their model, the top view and the side or front view. Team B must build a model that respects these views using all the cubes. Team A then gives the third view to Team B and asks them to see if, with this new information, they should change the location of certain cubes. Afterwards, team B compares its final model with that of team A and the students discuss the similarities and differences. It is then the turn of team A to try to reproduce the model of team B by following the same approach.
Note: Even with three views, it is possible to build two models that are not perfectly identical.
Source: translated from Guide d’enseignement efficace des mathématiques de la 4e à la 6e année, Géométrie et sens de l'espace, Fascicule 1, p. 92.
Extensions
- Ask students to find the perimeter of the base of their model, the surface area and its volume. The unit used to measure lengths of the model should be centimetres.
- Students must now convert these three measurements to metres, the base metric unit. Students should be able to apply and understand the relationships that exist between the units in order to convert measurements. If they do not yet understand all the relationships, go back to the conversions of linear measures (units of length) and then generalize the units of area and volume. Also, students can repeat all calculations of perimeter, area and volume, and compare their answers to highlight the relationships.
Activity 2: What Do We See from Above?
Ask the students to draw all the top views of a structure made up of four connected interlocking cubes. Tell them that they must first try to visualize all the possible structures and draw the corresponding top views without using manipulatives. Form teams of two and invite students to compare their answers. Allow them to use interlocking cubes to verify their thinking.
Note: The following 11 top views are possible.

Source: translated from Guide d’enseignement efficace des mathématiques de la 4e à la 6e année, Géométrie et sens de l'espace, Fascicule 1, p. 93.
Activity 3: Draw Me a Picture
Provide students with an illustration of a model (see examples 1, 2 and 3 below). Ask them to draw the front, side and top views on isometric dot paper. Form teams of two and invite students to compare their answers. Allow them to use interlocking cubes to verify their thinking.
Note: The illustration shows the model from one angle only. Since it could be made up of more cubes than those seen in the illustration, the drawings of the views may vary.
Examples 1, 2 and 3:
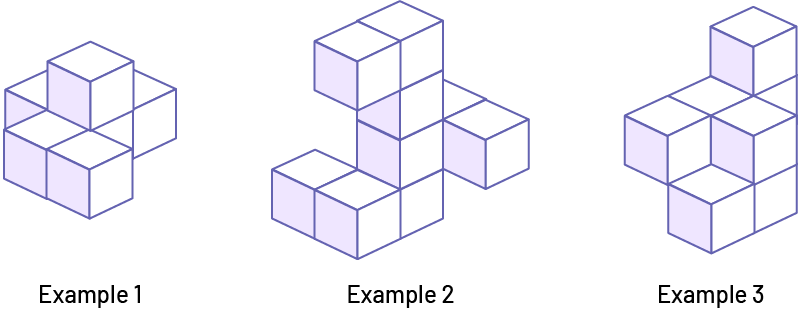
Source: translated from Guide d’enseignement efficace des mathématiques de la 4e à la 6e année, Géométrie et sens de l'espace, Fascicule 1, p. 93.
Ask students:
- How do you know that the front view is correct?
- What are the benchmarks in the illustration that allow you to draw the front view? the top view? the side view?
- How many squares should you have in total?
- Can the drawings be different? Explain your answer.
Activity 4: The Smallest Opening
- Form teams of two. Each team must:
- Examine the 12 flat pentacubes made of interlocking cubes and draw the shape of the smallest opening through which they could pass, no matter which way they inserted them. Solution:

- Examine the representations of the 17 non-flat pentacubes (Appendix 5.3) and draw the shape of the smallest opening through which they could pass the corresponding objects, regardless of which way they insert them. Solution:

Source: translated from Guide d’enseignement efficace des mathématiques de la 4e à la 6e année, Géométrie et sens de l'espace, Fascicule 2, p. 76.
Appendix 5.3 the 29 pentacubes
