C1.2 Create and translate patterns using movements, sounds, objects, shapes, letters, and numbers.
Skill: Representing Patterns in Different Ways
Repeating Patterns
Using multiple representations of the same pattern to communicate understanding is an essential part of developing algebraic thinking. Repeating patterns can be represented using concrete or visual (semi-concrete) materials, symbols, or verbal descriptions.
Examples of Representation Modes
|
Representation Modes |
Examples |
|
Concrete Material |
Patterns made with: the body, such as sounds, movements or positions, or objects, such as necklaces
Pattern A 
Pattern B 
|
|
Visual (Semi-Concrete) Material |
Illustrated Pattern Pattern C 
|
|
Oral Description |
Pattern A: "It's a pattern because the standing arms outstretched and squatting positions always repeat in the same order." Pattern B: "The necklace makes a pattern because the four colours always repeat in the same way." Pattern C: "It's a pattern because the pattern core, which is a fire truck followed by a firefighter, always repeats in that order." |
|
Symbols |
Pattern A: The structure of the pattern is AB. Pattern B: The structure of the pattern is ABCD. Pattern C: The structure of the pattern is AB. |
Students can demonstrate their understanding of the concepts of regularity and relationships and further develop their ability to recognize, describe, and extend a pattern by changing the representation of a pattern without changing the rule.
The change can be from one mode to another; for example, a non-numeric pattern such as the one shown below can be represented with the movements "tap, jump, jump" or with small plastic animals "cat, dog, dog".

Change can also occur within the same mode of representation.
Example


By representing a pattern with symbols, its structure can be clearly determined. To do this, letters are used in alphabetical order. Each new letter represents a different element in the pattern. In the patterns shown above, the structure is AABB.
Often, multiple representations of the same patterns can be used as a good mathematical justification, and each contributes to the understanding of the ideas presented. The ability to create, interpret, and represent ideas in multiple ways is a powerful tool.
Source: translated from Guide d’enseignement efficace des mathématiques de la maternelle à la 3e année, Modélisation et algèbre, Fascicule 1, Régularités et relations, p. 34-36.
Growing Patterns
Growing patterns, like repeating patterns, can be represented using concrete or visual (semi-concrete) materials, symbols, or verbal descriptions.
Examples of Representation Modes
|
Representation Modes |
Examples |
|
Concrete Material |
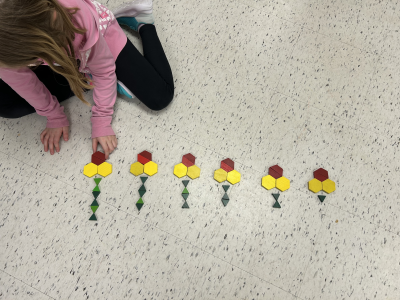 |
|
Visual (Semi-Concrete) Material |
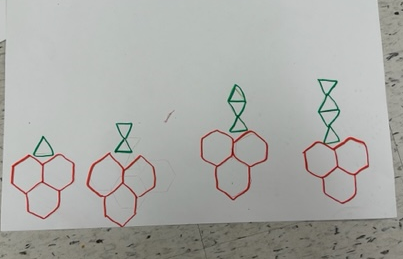 |
|
Oral Description |
"A trunk is always added to the trunk of the previous tree." |
|
Symbols |
In the drawing above, the pattern rule can be represented symbolically by the addition sign and the number (+ 1). |
Teachers should provide opportunities for students to explore and create different representations of the same pattern. For example, they may be asked to exchange their representation and reproduce the pattern using concrete or visual (semi-concrete) materials, and vice versa. It is important to focus on the relationship between the two representations so that the focus remains on reasoning and not on numerical calculation.
Number Patterns
The acquisition of concepts related to number patterns is a prerequisite to the study of more abstract algebraic concepts. The exploration of various representations of number patterns facilitates the entry into the world of algebra.
In the primary grades, certain representations facilitate the development of problem solving, reasoning, and communication skills. The representations of number patterns explored in the Primary Division are made using concrete or visual (semi-concrete) materials (hundreds chart, number pocket, number line, number grid), symbols, and oral descriptions.
Examples of Representation Modes
|
Representation Modes |
Examples |
|
Concrete Material |
  |
|
Visual (Semi-Concrete) Material |
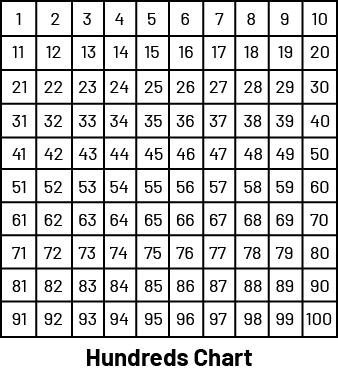 |
|
Representation Modes |
Examples |
|
Visual (Semi-Concrete) Material |
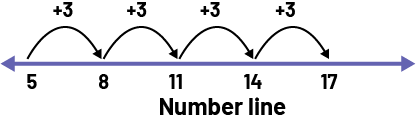
|
|
Oral Descriptions |
"The number pattern is: six vegetables in the first week, 12 vegetables in the second week, 18 vegetables in the third week, 24 vegetables in the fourth week and 30 vegetables in the fifth week. The pattern rule is that the vegetable garden has six more vegetables every week." |
|
Symbols |
In the previous example, the pattern rule in the number pattern is represented by the sign + and the number 6, that is + 6. |
Representations of number patterns are made using a variety of concrete and visual (semi-concrete) materials. The types of manipulatives listed below can be used as models to further the understanding of number patterns:
- number pocket charts;
- number grids (for example, hundreds chart);
- number lines;
- calculators.
Number Pocket Charts
There are many patterns in a number pocket chart, and many arrangements of the patterns. In a chart of 100 such as the one shown below, a pattern with the + 2 pattern rule will be arranged in a row (for example, 61, 63, 65…); if the pattern rule is + 10, the pattern will be arranged in a column (for example, 7, 17, 27…); if the pattern rule is + 11, it will be arranged in a diagonal line (for example, 1, 12, 23…). In a number pocket chart, number cards can easily be moved, turned around, or removed to create patterns.

Number Grid
Possible strategies for exploring number patterns with a 50s number grid include:
- Use bingo chips to identify a pattern on a 50s grid. Using removable tokens helps students make changes without erasing.
Example 1
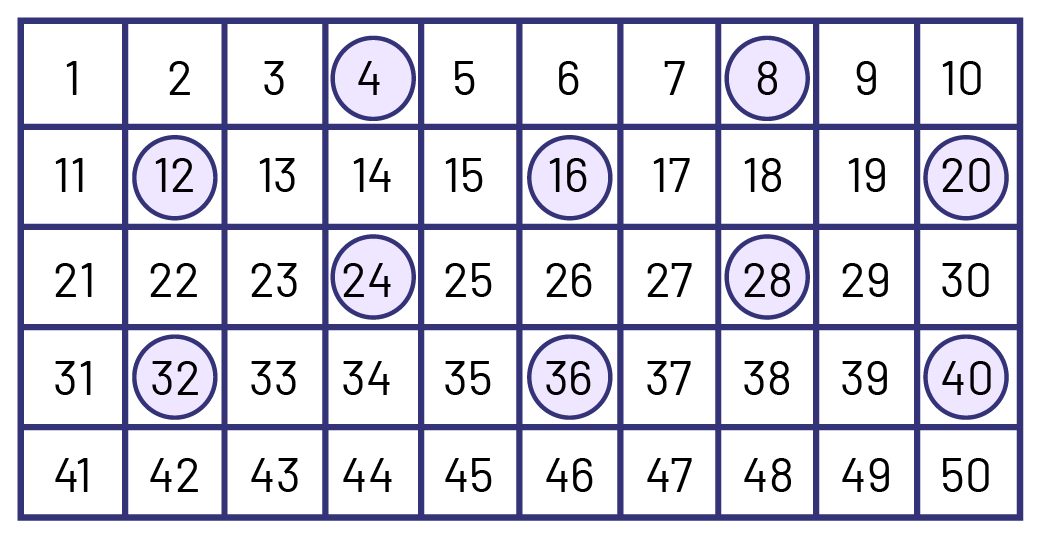
- Use a paper cut-out and move it around the grid to highlight a number pattern. Determine the pattern rule in this pattern and extend it.
Example 2
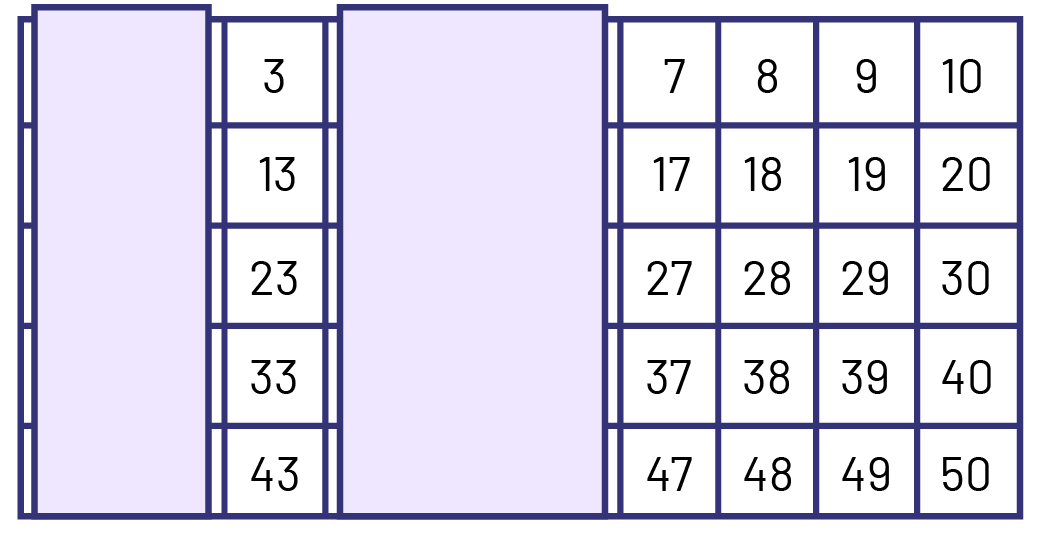
Pattern: 3, 13, 23, 33, 43
Pattern rule: +10
- Introduce sections of the grid and identify a number pattern. Determine the pattern rule in this pattern.
Example 3
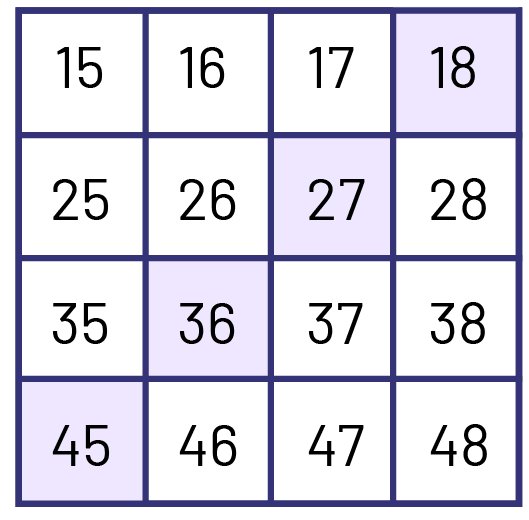
Pattern: 18, 27, 36, 45
Pattern rule: + 9
As students explore these number patterns with a grid of 50, ask them questions to develop their ability to represent them.
- What do you notice about the numbers in the pattern?
- What change is there from one number to another in each row or column? (They increase or decrease by 1 or 10.)
- If the cut-out is moved and the first number is 5, will the number 46 appear? Explain your answer.
Number Lines

Used as a model for discovering pattern rules in patterns, the number line helps to represent a variety of patterns. The representation of the number line can be concrete or visual (semi-concrete).
"Mathematical models are mental maps of relationships that can be used as a tool for problem solving; for example, when mathematicians think of a number, they may have a number line in mind. They visualize the numbers, relative to each other, on the line and picture the movements on the line (Fosnot and Dolk, 2001, p. 77)."
Some possible strategies for exploring number patterns with the number line include:
- Initially, using a laminated number line on which the pupils can make jumps across the numbers, at regular intervals, by jumping with their fingers or by using any object.
- Using a number line drawn on a large sheet of paper, students circle the terms in the pattern and indicate, with arrows, the pattern rule in the pattern.
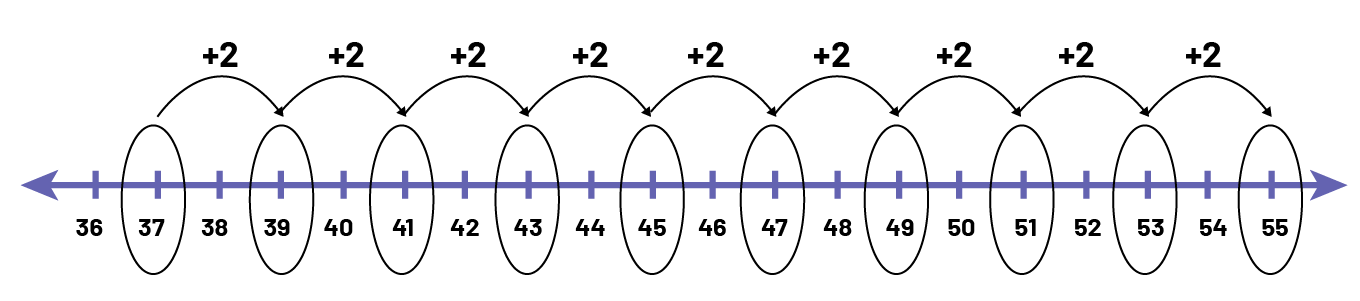
As students explore number patterns using a number line, ask questions such as:
- Would the number 48 be part of the pattern if we extended the line?
- What do you notice about the circled numbers on the number line?
- What is the pattern rule in the following? How is it indicated?
- If the pattern began with the number 1, what would be the same? different?
- If the pattern began with the number 2, what would be the same? different?
Source: translated from Guide d’enseignement efficace des mathématiques de la maternelle à la 3e année, Modélisation et algèbre, Fascicule 1, Régularités et relations, p. 50-59.
Skill: Creating Various Patterns
Before creating patterns, students must first recognize, compare, describe, change the representation for, and extend a wide variety of patterns.
Students can demonstrate their understanding of regularities in patterns by creating a pattern and explaining it.
Initially, the use of manipulatives is essential for representing patterns because, by creating them, students can easily change one element of the pattern and check the rule. When students draw it on paper, they focus on the element to be reproduced rather than looking at the whole pattern and thinking about what the rule is.
Initially, teachers should have students work in small groups to encourage the exchange of ideas. They can provide them with the attributes and structure of the pattern, as well as the materials required to create it.
Teachers may then ask students to create their own patterns and exchange them for a partner's pattern. Students can then construct a different representation of the received pattern, describe its structure, extend it, or produce a completely different one for comparison. The number of elements in the pattern should be limited, however, as some students use too many, making it difficult to identify the structure of the pattern.
Source: translated from Guide d’enseignement efficace des mathématiques de la maternelle à la 3e année, Modélisation et algèbre, Fascicule 1, Régularités et relations, p. 39-40.
Teachers who want to engage students in creating number patterns can follow the same approach suggested for non-numeric patterns.
Some examples of activities to create number patterns include:
- Ask students to create patterns with a rule about the intervals 1, 2, 5, or 10 and compare them.
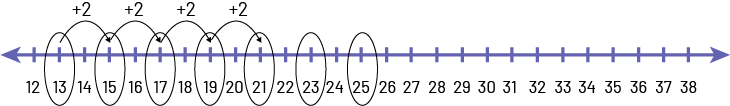
- Ask students to create a number pattern that starts with 13 and has an addition pattern rule of +2

Note: It is important for students to make a record of their process for reference during the mathematical exchange; for example, they can:
- circle the terms of each pattern;
- draw an arrow above each jump to indicate the interval;
- symbolically represent the pattern rule with the + sign, as in the following examples.
Possible Solution
Source: translated from Guide d’enseignement efficace des mathématiques de la maternelle à la 3e année, Modélisation et algèbre, Fascicule 1, Régularités et relations, p. 63-65.
