C2.2 Determine whether given pairs of addition and subtraction expressions are equivalent or not.
Activity 1: Let's Play Cards
Prepare cards with the = sign and the ≠ sign on them, as well as cards like the ones below, made with stickers or illustrations.

Group students into teams of two. Each student draws a card. The team members must then determine whether or not the two cards are equivalent. They indicate this with an = card or a ≠ card.

When students have done several similar activities, write down the number sentences that represent the equality or inequality.
Example
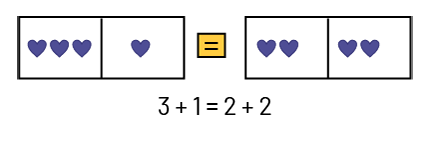
Extensions
Ask students to help you prepare the cards for the game. They must find all the combinations for 10 objects and show them on a card divided in half. Do the same for 9, 8, 7, 6, 5, 4, 3, 2, 1.
Compare the possibilities for each number in an organized list and find patterns. (There are 11 combinations with 10 objects, 10 combinations with 9 objects, 9 combinations with 8 objects, etc.)
Source: translated from L’@telier – Ressources pédagogiques en ligne (atelier.on.ca).
Activity 2: Let's Roll Some Dice!
Divide a placemat, sheet of paper, or shoebox lid into two equal parts by drawing a line.
Have two students roll a die.
Use counters or cubes to represent the roll of one die on one side of the placemat and the amount of the second die on the other side.
Example
4 and 3
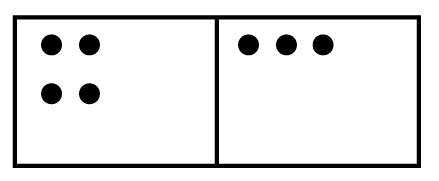
Ask students if the quantities are equivalent or not on both sides. If the quantities are different, ask students what it would take to make the two sides equivalent.
Ask them to think of several ways; for example, one student may say to remove a counter from the left side. Another student may say to add a counter to the right side, etc. Once students have had several opportunities to explore the concept of equivalence, represent the answers symbolically as well.
Example
4 - 1 = 3
4 = 3 + 1
4 + 1 = 3 + 2
Source: translated from L’@telier – Ressources pédagogiques en ligne (atelier.on.ca).
Activity 3: Representing Expressions
Set up three centres in three different areas of the classroom.
In one centre, provide students with ten frames and two-coloured counters (or two-coloured cubes).
In another, provide students with interlocking cubes (in two colours).
In another, provide students with tray scales and interlocking cubes.
Provide students with different expressions involving addition and subtraction, and ask them to check whether or not the expressions are equivalent. Students will explore different ways of representing whether or not pairs of expressions are equivalent as they rotate through the centres.
In the ten frame centre, select phrases with a sum of 10 or less so that students can see the quantity on both frames.
In the interlocking cubes centre, have students build towers with pairs of addition expressions using two colours of cubes to represent the two terms and then put them side by side to compare.
Facilitate a mathematical discussion about a few pairs of expressions to discuss students' findings. Have them carefully observe the expressions and predict whether they are equivalent, then represent them either with ten frames or with towers of cubes.
For example, if the expressions are 8 + 2 and 2 + 8, support the students see that the two expressions have the same value. Represent them to see that the expressions are equivalent. Ask them if this is true for all numbers (conjecture). Then ask them to explore.
Return to the large group to have students verbally generalize, for example, if two numbers are added together, the same quantity is obtained, even if the order of the terms is not the same.
Activity 4: Matching Expression Pairs to a Number Line
Prepare pairs of expression cards and corresponding cards that represent them as hops on two number lines.
Give half the class cards with pairs of expressions and the other half cards with jumps on two number lines.
Ask students to circulate and find the partner who has the card associated with theirs.
When everyone has found their partner, start the game again.
