C1.3 Determine pattern rules and use them to extend patterns, make and justify predictions, and identify missing elements in patterns represented with shapes and numbers.
Skill: Determining and Using Rules to Extend Patterns
Understanding Change
Students live in a changing world. Understanding that change is a part of life and that most things change over time (for example, each year their height and weight increases and their feet get longer) is the final component of developing algebraic thinking. Observed changes can be described qualitatively (for example, I am taller than last year; my hair is longer; the bucket filled with water quickly during the storm; it is colder than this morning) and quantitatively (for example, I grew 2 cm this year; the bucket of water filled with 50 ml in 30 minutes; the temperature dropped 6°C in 3 hours). Students need to learn to observe and understand changes in patterns.
Change and regularities are two concepts that cannot be separated in the study of patterns. Students realize that a
change in one term affects the next term. Subsequently, observing the changes and the relationships between these
changes allows them to predict other terms in the pattern and thus generalize.
As an example, by observing the
non-numeric repeating pattern below, students can extend it and find the regularity, as well as describe the pattern
rule by explaining the repetition of the sun, sun, heart pattern.
Non-Numeric Repeating Patterns
By examining the change from one shape to the next, students observe a pattern rule that helps them predict the shapes in the next terms.
Source : Guide d’enseignement efficace des mathématiques de la maternelle à la 3e année, p. 21-22.
The main goal is to develop students' algebraic reasoning by enabling them to justify the extension of a non-numeric or number pattern and to explain the relationships that exist between the terms of the pattern.
Source : Guide d’enseignement efficace des mathématiques de la maternelle à la 3e année, p. 27.
Relationships Between Terms
Using a variety of representations and materials, students explore the concept of regularity in non-numeric and number patterns, and communicate, in their own words or through personal representations, their observations and perceptions of the relationships among the terms in the pattern.
In the primary grades, students learn to recognize relationships that exist between terms in a pattern. By examining and exploring patterns, they identify how this information can be used to determine what needs to be added to a pattern to extend it. By discovering relationships, students realize that the next terms in the pattern are not chosen randomly. Finding patterns is, in itself, an important problem-solving strategy.
Students continually redefine their mental image of patterns. Their representation is often limited by the examples they are presented with or by their personal experiences. It is therefore important that teachers present a variety of representations and patterns during activities to facilitate the integration of the concept. The key is to develop students' algebraic reasoning by enabling them to justify the extension of a non-numeric or number pattern and by making explicit the relationships that exist between the terms of the pattern.
Source : Guide d’enseignement efficace des mathématiques de la maternelle à la 3e année, p. 27.
Non-number patterns
In order to extend a pattern, students must recognize the elements of the pattern and determine their order. By extending a pattern while justifying their choice, students communicate their understanding of what the pattern rule is; for example, a student may say, "I'm going to be the sailboat because right before me is the balloon, and the pattern is always sailboat, balloon, sailboat, balloon repeating."
Using their bodies or manipulatives, students can explore the extension of a pattern and make changes more easily. They can also extend a pattern that others have constructed.
Source : Guide d’enseignement efficace des mathématiques de la maternelle à la 3e année, p. 38.
Number Patterns
Very early on, students become aware of regularities in their environment, in nature, in the objects around them. This is why it is possible to introduce them to number patterns as early as Grade 1. At the same time, students develop a sense of number, can count in intervals and backwards, and eventually acquire the concept of addition as a grouping of objects. All of these concepts have an important connection to learning number patterns.
Exploring the base ten numbering system, synonymous with the decimal system, allows students to discover that there is a repetition of digits from 0 to 9 in counting beyond 9 (10, 11, 12, 13, 14, 15…). Seeing and justifying this regularity in the decimal system improves their understanding of number sense and groupings (units, tens, hundreds, etc.). For example, by counting in increments of 2, starting at 16, students observe a predictable pattern in the numbers (16, 18, 20, 22, 24, 26, 28, 30, 32…). This is a first step toward exploring multiples of 2. This understanding also leads them to develop the ability to count from any number using any leap.
Similarly, when students count by 5s, they quickly recognize a pattern, that is, that the units digit alternates between the digit 0 and the digit 5 (5, 10, 15, 20). They can generalize this discovery informally by saying that any number that is a multiple of 5 will end in 5 or 0.
It may be helpful for teachers to offer students the opportunity to identify patterns in a number grid to help them develop their conceptual understanding of number and the base ten numbering system.
Source : Guide d’enseignement efficace des mathématiques de la maternelle à la 3e année, p. 49-50.
Skill: Making Predictions and Justifying Them
The use of concrete and semi-concrete materials and a variety of representations and rules allows students to make and justify near and far predictions.
Making a close prediction is to indicate or represent what the next terms in a given pattern will look like. The prediction can be verified simply by extending the pattern.
Making a far prediction is to indicate or represent what a pattern will look like far beyond a given section. Calculations are often required to make a correct prediction or to check its plausibility.
Source : The Ontario Curriculum. Mathematics, Grades 1-8 Ontario Ministry of Education, 2020.
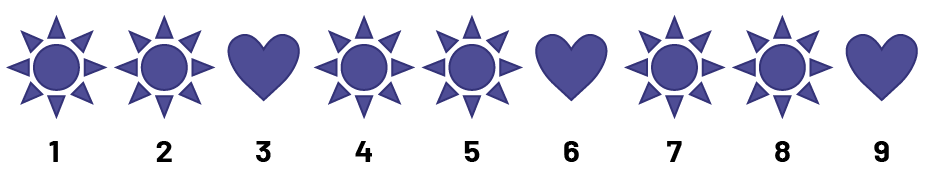
Students can best describe a pattern when they understand the relationship between each term in the pattern and the position each term occupies in the pattern. They can do this by numbering each term in the pattern in turn.
In this way, students can refer to specific terms in the pattern (for example, in the pattern above, the suns are in the 3rd, 6th , and 9th positions from the left). By analyzing the relationship between the position and the term, it is easy to predict the next terms in the pattern without having to extend it. This analysis allows students to generalize (for example, a sun will be in the 12th position, since it is in the 3rd position of each pattern. The position of the sun is always a multiple of 3).
In the problem situation "How many suns are needed to complete 10 patterns in this pattern?", elementary students can informally discuss, model and create multiple representations, describe them, and conclude by identifying the number of suns needed and justifying their approach. Exploring this type of problem allows students to develop their algebraic thinking and serves as a foundation for using a rule and variables in future grades.
Source : Guide d’enseignement efficace des mathématiques de la maternelle à la 3e année, p. 38-39.
In growing patterns, there is also a relationship between the position of each shape and the number of elements in each shape. This relationship is a very important mathematical concept, which leads to a more formal generalization, the pattern rule formulation.
In carefully analyzing a pattern, for example, students observe that the shape in the 1st position has two
geometric shapes, the shape in the 2nd position has three, the shape in the 3rd position has
four, etc. Their observation is that there is always one more geometric shape than the position of the shape. This
observation, the matching rule, allows them to find any term in the pattern without having to extend
it.
Informal discussions dealing with the relationships between shapes and the number of elements in them can
occur by determining the rule and extending the pattern.
Source : Guide d’enseignement efficace des mathématiques de la maternelle à la 3e année, p. 45-46.
Skill: Finding Missing Terms in Patterns
In non-numeric and number patterns, students need to determine what is in a predetermined position (before, after, or within the pattern). Therefore, they need to determine the pattern rule and then identify the missing shape or number.
Example
Find the 2nd term of the following growing pattern.
Source: En avant les maths! grade 2, CM, Algebra, p. 4.
The study of regularities in a number pattern can be continued using a number grid or number line with missing numbers. Students must first find the rule in order to discover the missing numbers, and then explain the addition or subtraction pattern rule. The calculator can be helpful in solving these types of problems.
Examples
Missing Numbers in a Partial Number Grid
A grid table which certain spaces are numbered and shaded. The model shows the numbers 11 and 12 and two followed by two shaded squares. The following row shows number 21 and 22 and two shaded squares. The third row has the first two squares shaded and number 33 and 34. In the last row, the first two numbers are 41 and 42 and the last two squares are shaded.
Missing Numbers on an Open Number Line
Source : Guide d’enseignement efficace des mathématiques de la maternelle à la 3e année, p. 67.
Knowledge: Pattern Rules
Pattern rule: Rule which allows to extend a pattern by respecting the difference between the terms (also called constant jump).
Rule of correspondence : Rule that allows to extend a pattern by establishing the relation between the position and its term.
Source : The Ontario Curriculum. Mathematics, Grades 1-8 Ontario Ministry of Education, 2020.