C1.2 Create and translate patterns using various representations, including shapes and numbers.
Activity 1: Calculated Patterns (Create a Pattern Using the Calculator)
Develop algebraic reasoning by exploring patterns using a calculator.
Give an instruction such as, "Press the [+], [5], and [=] keys. You will see the number 5 displayed."
Note: Ensure that students' calculators have the Constant Factor function.
Help them to discover patterns by asking questions such as:
- What will happen if you press the [=] key three more times?
- What will be the number if you press the [=] key 5 times?
- What happens next when you count in intervals of 5?
- How do you count in increments of 10 using a calculator?
- Does the number 78 show up when you count by 10s?
- How many times must you press the [=] key to reach 100?
- Counting by 2s, will the number 34 be in the pattern? How can you tell?
Source : Guide d’enseignement efficace des mathématiques de la maternelle à la 3e année, p. 66.
Activity 2: Invasion of Planet Ice
Summary
In this activity, students create a number pattern from a non-numeric growing pattern.
Materials
- sufficient quantity of pattern blocks for the whole class
- math journal (one per student)
Directions
Explain to students that an imaginary planet called Ice is being invaded by beings from outer space. Every day, new invaders arrive.
Project on the screen an invader created with the following pattern blocks: a yellow hexagon, three green triangles, and an orange square as shown.
Ask students to count the pattern blocks used. On the interactive whiteboard, write "Day 1" next to the invader.
Tell students that on the second day, two more invaders arrive on the Planet Ice. Ask them: How many invaders are there now?
Make the invaders and place them under the first day's invader, then write "Day 2" next to these new invaders.
Ask students the following question: If two new invaders arrive on the planet each day, how many will there be after seven days?
Ask students to write the number pattern that corresponds to the non-number pattern in their math journals. Circulate around the classroom and ask them to explain their reasoning.
Continue the activity by inviting students to create their own pattern of invaders using at least two pattern blocks to represent each one and to write the corresponding number pattern.
To extend the task, invite students to determine the total number of pattern blocks used each day and to indicate whether this pattern of numbers forms a pattern.
Source : Guide d’enseignement efficace des mathématiques de la maternelle à la 3e année, p. 130.
Activity 3: Guess My Pattern
Summary
In this activity, students are asked to create a non-numeric repeating pattern by applying specific criteria.
Materials
- attribute blocks (one set per student or in pairs)
Directions
Allow students to work individually or in teams. Provide each student or team with a set of attribute blocks. Present the problem and criteria to the students and ensure that they understand the task.
Problem
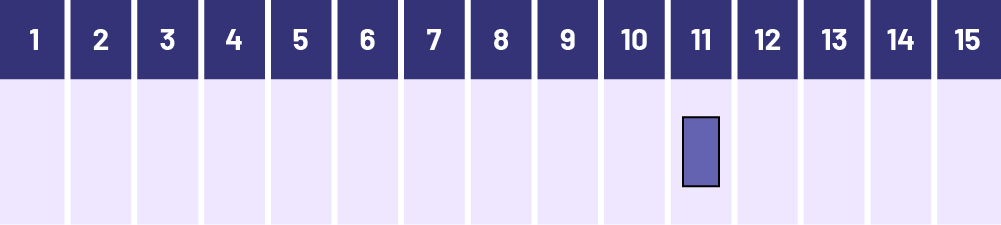
Create a non-numeric repeating pattern that meets the following criteria.
Criteria
The pattern must be created using attribute blocks.
Each term in the pattern must have three attributes: size, shape, and colour.
The term in the 11th row of the patternmust be a large blue rectangle.
Source : Guide d’enseignement efficace des mathématiques de la maternelle à la 3e année, p. 147.
Once the work is done, ask the students to circulate around the classroom to observe the different patterns created.
Then compare the different patterns with students using the following points:
- the attributes;
- the created pattern;
- the number of elements in each pattern;
- the position of each repeating term;
- the rule.
Source : Guide d’enseignement efficace des mathématiques de la maternelle à la 3e année, p. 147.