C1.2 Create and translate repeating, growing, and shrinking patterns involving whole numbers and decimal numbers using various representations, including algebraic expressions and equations for linear growing patterns.
Activity 1: Strategies (Linear Growing Patterns)
Cut out the equations, tables of values, and graphs from the table below. Divide students into teams and distribute the cut-outs to each team. Teams should combine the different linear pattern representations in such a way as to group the graph, table of values, and equation by comparing the initial values, b, and the constant rate, m (for example, 1A, 2B, and 3C could represent the same situation).
Debrief as a class, focusing on the students' grouping strategy/strategies.
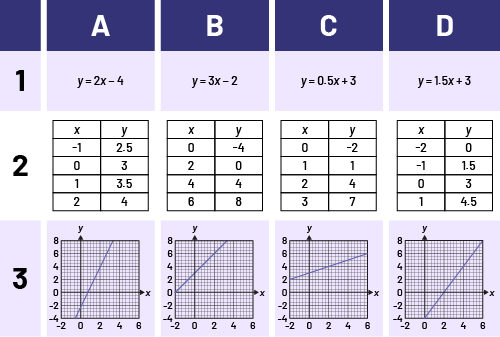
Strategy 1: Solution determined by comparing the initial values in the 3 forms of representations.
I look in the equation for the initial value (the b in the formula y = mx + b). In the table of values, I look for the value of y when x = 0. In the graph, I look for the value of y when the line crosses the y-axis..
First situation: The line crosses the y-axis at (y = -4).
Solution A1, B2, D3
Second situation The line crosses the y-axis at (y = -2).
Solution B1, C2, A3
Third and fourth situations, C1 and D1 : I have a problem, because there are 2 possibilities when I find the value y when x = 0, either C1 (b = 3) and D1 (b = 3). I can't go any further using this strategy.
Strategy 2: Solution determined by comparing the constant rates in the 3 representations.
I will look at constant rates in equations, jumps in value tables, and slope of lines in graphs.
First situation : A1 has a constant rate of 2 in the equation, which implies a sloping increasing line in the graph D3. This implies, for each value in x, jumps of 2 for the y values in the table of values B2.
Solution: A1, B2, D3
Second situation: B1 has a constant rate of 3 in the equation, which implies a steeply sloping increasing line in the graph A3. This implies, for each value in x, jumps of 3 for the y values in the C2 table of values.
Solution: A3, B1, C2
Third situation: C1 has a constant rate of 0.5 in the equation, which implies a line that climbs slowly in the C3 graph. This implies, for each value in x, jumps of 0.5 for the y values in the table of values A2.
Solution: C1, A2, C3
Fourth situation: D1 has a constant rate of 1.5 in the equation, which implies a linear line that climbs quite rapidly in graph B3. This implies, for each value in x, jumps of 1.5 at values of y in the table of values D2.
Solution: D1, D2, B3
Source : En avant, les maths!, 7e année, CM, Algèbre, p. 5-7.
Activity 2: Creating a Linear Growing Pattern
Materials
- interlocking cubes;
- grid paper;
- blocks or objects of any kind.
Prepare 5 stations with manipulatives. At each station, students will have to create a linear growing pattern with the material. Once completed, they will have to create a table of values, make the graph and establish the algebraic equation defining it. Allow about 15 minutes per station. Take pictures and use them to report back to the class. Discuss strategies, vocabulary and different representations (materials, table of values, graphs and equations).
Note: For each pattern, students should be able to identify the initial value (Term Number 0) and the constant rate. If necessary, guide students.