D2.2 Determine and compare the theoretical and experimental probabilities of an event happening.
Skill: Comparing Probabilities
Proportional Reasoning
There is an important link between probabilistic thinking and proportional reasoning. Proportional reasoning helps students recognize the equivalence relationship between two probability situations, thus contributing to an understanding of the concept of theoretical probability. Teachers should provide students with a variety of activities that encourage them to make this connection.
Example
The teacher presents the students with two bags and their contents. Bag A contains 3 green cubes and 2 purple cubes. Bag B contains 6 green cubes and 4 purple cubes. The teacher asks them the following question, "Which bag do you think has a greater probability of randomly picking a green cube?"
A student responds, "I think it's in bag B, because there are more green cubes, so there's more chance of picking one at random." This is typical of a learner who has not yet acquired proportional reasoning in a probability context. He or she has not understood that the two situations are equivalent, since the number of green cubes and the number of purple cubes have been doubled.
Another student says, "The probability of randomly taking a green cube from bag A is \(\frac{3}{5}\), while with bag B the probability is \(\frac{6}{10}\); since \(\frac{3}{5} = \frac{6}{10} \), then the probability is the same for each bag."
This answer demonstrates the application of proportional reasoning in a context of equivalent fractions.
Finally, another student rearranges the cubes and concludes:
"In bag A, I see 3 green cubes out of a total of 5 cubes, while in bag B, I see 3 groups of green cubes out of a total of 5 groups. I judge that it is just as likely to randomly pick a green cube from bag A as from bag B." Rearranging the cubes is a concrete way to represent the proportionality relationship.
Source: translated from Guide d’enseignement efficace des mathématiques, de la 4e à la 6e année, Traitement des données et probabilité, p. 144-145.
Knowledge: Theoretical Probability
The analysis of data collected during randomized experiments allows us to better understand the concept of theoretical probability.
The theoretical probability quantifies the randomness and uncertainty of an event or outcome. This quantity can be represented by a fraction between 0 and 1. In the game of heads or tails, for example, the theoretical probability of getting the outcome "tails" is equal to \(\frac{1}{2}\). This fraction indicates that the game has two equally likely outcomes and that one of these outcomes is "tails". In other words, in this game, there is a 50/50 chance of getting "tails".
Teachers should be aware that if all possible outcomes of an experiment are equally likely, the theoretical probability of any outcome A is formally defined as follows.
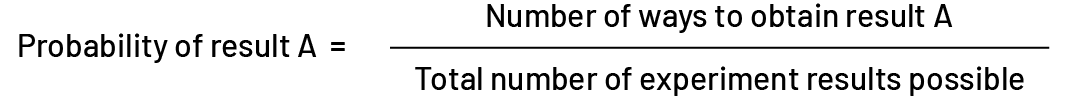
Thus, if one seeks to determine the probability of obtaining an even number by rolling a six-sided die, it suffices to recognize that there are six possible results (1, 2, 3, 4, 5 and 6) and that three of these results correspond to an even number (2, 4 and 6). We can therefore say that the theoretical probability of obtaining an even number is equal to \(\frac{3}{6}\), that is to say that there are three possibilities out of six that the result corresponds to an even number. The notation P(A) is commonly used to describe the probability of any outcome A. Thus, in the previous situation, we could write P(even number) = \(\frac{3}{6}\). Note, however, that in the Junior Division it is best to help students develop an understanding of the concept of theoretical probability without placing too much emphasis on definition or formal notation. The strategies presented in what follows support this position.
In some situations, we realize that a desired outcome can never occur (for example, getting a number greater than six by rolling a die). In this case, we say that the probability of this outcome is 0. In other situations, it is certain that the desired outcome will occur (for example, getting a number less than 7 by rolling a die). We say that the probability of this result is equal to 1.
Theoretical probability helps in making informed decisions in any random situation. Since in such situations it is impossible to predict the outcome without the risk of being wrong, we can reduce this risk by determining the theoretical probability of each outcome and choosing the outcome with the highest probability. This is a way of "mathematizing chance".
Source: translated from Guide d’enseignement efficace des mathématiques, de la 4e à la 6e année, Traitement des données et probabilité, p. 137-138.
Example
Teachers present students with the spinner below and ask them to determine the probability of the arrow stopping in the yellow area.

This situation may cause cognitive dissonance for some students. Intuitively, they know that it is more likely that the arrow will stop in the yellow sector than in the red or blue sector. However, they tell themselves that there are only three possible outcomes (yellow, blue and red) and, therefore, the probability that the arrow will stop in the yellow area should be equal to \(\frac{1}{3}\). Of course, the flaw in this reasoning is that the three outcomes are not equally likely, since the area of the yellow sector is greater than the area of each of the other two sectors. Teachers can then suggest that students divide the yellow sector as follows.
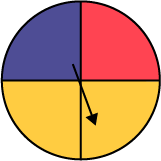
We can now consider that there are four possible outcomes (yellow, yellow, blue and red) and that they are equally likely, since the area of each sector is the same. The probability that the arrow stops in the blue sector is \(\frac{1}{4}\). The same is true for the probability that it stops in the red sector. However, the probability that the arrow stops in the yellow sector is equal to \(\frac{2}{4}\). It is therefore twice as likely that the arrow will stop in the yellow sector as in the blue or red sector.
Other examples related to manipulatives do not provide as much opportunity to emphasize the idea of equiprobability of outcomes. When using dice or coins, it is assumed that the outcomes are equally likely, in other words, that the dice are not loaded or that the coin can naturally fall as often on one side as on the other. In the case of the bag of marbles, it is assumed that it is not possible to distinguish one marble from the other by touch, as it would be if, for example, the red one were larger than all the others. Therefore, when using these materials, teachers should encourage students to always establish the premise that the outcomes are equally likely.
Source: translated from Guide d’enseignement efficace des mathématiques, de la 4e à la 6e année, Traitement des données et probabilité, p. 140-141.
Theoretical probability is an abstract mathematical concept that is not always easy to grasp. However, understanding it is essential to the development of probabilistic thinking. To ensure that this understanding is not simply a matter of learning a formula, it must be the result of a lengthy process in which teachers expose students to a variety of probability games, situations, or experiments that allow them to confront theory and practice. This approach often creates counters misconceptions for students, who may be led by intuition or faulty analysis in the face of a particular situation. Even among adults, it is very common to misunderstand a seemingly simple random situation. Therefore, in the junior grades, it is very important to keep situations relatively simple and to work with concrete materials. The following describes how teachers can help students develop this conceptual understanding.
Development of the Concept of Theoretical Probability
In order to develop an understanding of the concept of theoretical probability, students need to use both their intuitive and analytical thinking, so it is important that teachers present them with situations that involve both theory and practice in order to encourage a back-and-forth between these two modes of thinking.
From an early age, students develop an informal understanding of the concept of probability. Using a die, for example, in the context of various games, they recognize that there are six possible outcomes. Over time, they develop an intuitive understanding that, although these outcomes are random, they are also equally likely. In the junior grades, students gradually develop an understanding of the concept of theoretical probability. In Grade 4, for example, they can state that there is a one-in-six chance of getting the number four by rolling a die. In Grade 5, they learn to express this probability using a fraction, in other words, \(\frac{1}{6}\), and in Grade 6, they are able to use a decimal number or percentage to represent the probability of an outcome.
Manipulatives
To help students develop an understanding of the concept of theoretical probability, teachers should use a variety of manipulatives that make it easy to visualize different possible outcomes. The table below shows some examples.
| Material | Theoretical Probability |
|---|---|
|
Bag of Marbles or Tokens  |
If you draw a marble at random from a bag like the one shown here, there are 5 possibilities for the colour of that marble. Since there is only one marble of each colour, the probability of getting, for example, a green marble is equal to \(\frac{1}{5}\). |
|
Die  |
When we toss a 6-sided die, the results 1, 2, 3, 4, 5, 6 are equally likely. Therefore, the probability of obtaining the number 6 is \(\frac{1}{6}\). Other dice models can also be used.  |
|
Spinner 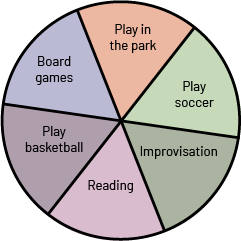 |
On the spinner shown here, there are 6 sectors of equal area. Therefore, the probability that the arrow will stop in the Play Soccer sector is equal to \(\frac{1}{6}\). |
|
Coin  |
When a coin is tossed, the heads and tails results are equally likely. Therefore, the probability of getting the heads side is \(\frac{1}{2}\). |
|
Two-Coloured Tokens  |
When a two-coloured token is tossed, the results of the red and blue sides are equally likely. Therefore, the probability of getting the red side of the token is equal to \(\frac{1}{2}\). |
Equally Likely Results
All of these materials help students develop an understanding of the concept of theoretical probability that is based on both intuition and reason, since in each case the possible outcomes are readily observable and limited in number. The spinner model, however, helps to emphasize, more than other models, the importance of ensuring that all possible outcomes are truly equally likely.
Source: translated from Guide d’enseignement efficace des mathématiques, de la 4e à la 6e année, Traitement des données et probabilité, p. 138-140.
Other Situations
As students develop an understanding of the concept of theoretical probability in simple situations, teachers can present them with situations that require more thought. For example, they can present situations that involve two successive choices, situations that involve a repeated experiment, or situations that involve simulation. Teachers can also use these situations to point out some common errors or misconceptions in probability.
In each case, however, it is important to take small steps, keep the situations relatively simple, and ensure that students understand the probability concepts they are learning. Otherwise, students may construct misconceptions that they will have difficulty deconstructing.
Source: translated from Guide d’enseignement efficace des mathématiques, de la 4e à la 6e année, Traitement des données et probabilité, p. 145.
Knowledge: Experimental Probability
Probability Experiment
Probability experiments are essential to the development of a sound understanding of the concept of theoretical probability. Once students have established the theoretical probability of some outcomes based on a scenario, teachers can suggest that they test that probability experimentally. This activity also helps students to better understand that uncertainty is inherent in any situation involving chance. For example, even if students establish that the theoretical probability of some outcome is equal to \(\frac{1}{4}\), there is no guarantee that they will get that outcome one-quarter of the time during an experiment.
Source: translated from Guide d’enseignement efficace des mathématiques, de la 4e à la 6e année, Traitement des données et probabilité, p. 142.
Probability experiments are used to support or challenge intuitive reasoning and are central to the development of probabilistic thinking.
Experimental Probability
Experimental probability is used in situations where it is impossible to determine the theoretical probability of a particular event or outcome. Examples include weather forecasting, estimating life expectancy, or determining the probability of hitting a free throw in basketball. In the curriculum, students are first introduced to the concept of experimental probability in Grade 5.
This concept should be taught using real-life situations. For example, teachers can present students with a variety of situations that involve the activity of randomly picking an object from a bag whose exact contents are unknown. They can also present students with situations where the possible outcomes are not equally likely.
Source: translated from Guide d’enseignement efficace des mathématiques, de la 4e à la 6e année, Traitement des données et probabilité, p. 150.
Example 1
The teacher groups the students into pairs. The teacher explains that they are to conduct an experiment that involves tossing a coin 20 times and recording the results. Before proceeding, students must predict how many times they will get each of the "heads" and "tails" results. Knowing that the probability of each outcome is equal to "heads" and "tails", most students may predict that they will get "heads" 10 times and "tails" 10 times.
When the students have completed the experiment, the teacher asks each team to write their results in a table.
Tossing A Coin, 20 Times
| Team | Results | T | H | |||
|---|---|---|---|---|---|---|
| 1 | H-T-H-H-T | T-T-H-T-T | T-H-H-T-H | T-H-H-H-H | 9 | 11 |
| 2 | T-T-H-T-T | T-T-T-H-T | H-T-T-T-T | H-T-H-H | 13 | 7 |
| 3 | T-T-T-T-T | H-H-H-T-T | T-H-H-T | T-H-H-T-H | 11 | 9 |
| 4 | H-H-T-H-T | T-T-T-H-T | T-H-T-T-H | H-T-H-T-H | 11 | 9 |
| 5 | H-H-T-T-T | T-H-H-T | T-H-H-T-H | T-H-T-T-H | 10 | 10 |
| 6 | H-T-H-H-T | H-T-H-H | T-T-T-H-H | H-T-T-H-T | 9 | 11 |
| 7 | T-T-T-T-H | H-T-T-H-H | T-T-T-H-T | H-H-H-T-T | 12 | 8 |
| 8 | T-T-T-H-H | T-T-T-H-H | H-H-T-H-H | H-T-T-H-T | 10 | 10 |
| 9 | H-T-T-T-T | T-T-T-T-T | T-H-T-H-T | T-T-H-T-T | 16 | 4 |
| 10 | T-H-T-T-T | H-H-T-T-T | H-T-H-T-H | T-H-T-H-T | 12 | 8 |
| Total | 113 | 87 | ||||
To help students better understand the concept of theoretical probability, the teacher encourages them to analyze the results. Students can note that only trials 5 and 8 produced 10 tails and 10 heads, while trials 1, 3, 4 and 6 were very close to this distribution. Students can also note that the results of trial 9 (16 tails and 4 heads) are very different from what the theoretical probability would suggest. However, this result helps to emphasize the idea that all outcomes are possible, even those that seem unlikely. Students can also note that, out of a total of 200 tosses, tails were obtained 113 times and heads 87 times.
Note: if the theoretical probability of any outcome of an experiment is known, the Law of Large Numbers states that the more times the experiment is done, the closer the ratio of the frequency of the outcome to the number of trials will be to the theoretical probability. This law is not being studied in the junior grades; however, teachers can take advantage of probability experiments to introduce the general idea to students.
Source: translated from Guide d’enseignement efficace des mathématiques, de la 4e à la 6e année, Traitement des données et probabilité, p. 142-143.
Example 2
Teachers have a box of identical thumbtacks and show students that if you throw a thumbtack in the air, it can fall out by pointing up or down.

Even though there are only two possible outcomes, there is no reason to believe that they are equally likely, so it is not possible to determine the theoretical probability of each. The teacher then reminds the students that on various occasions they have:
- determined the theoretical probability of any outcome;
- performed a probability experiment to check its plausibility;
- found that with a fairly large number of trials the ratio of the frequency of the desired event to the number of trials was comparable to the theoretical probability.
The teacher explains to students that experimental probability makes use of this finding, that is, if one performs a probability experiment enough times and records the frequency of each outcome, that frequency can be used to determine an approximation of the theoretical probability.
The teacher groups the students into teams of two. The teacher gives each team a thumbtack. The teacher asks the students to make 20 tosses, record the results, and determine, using a fraction, what the probability of each result might be. For example, one team gets the following results: 12 times pointing up and 8 times pointing down. The students might then say that the probability of the thumbtack falling pointing up is about 12/20 and the probability of it falling pointing down is about 8/20.
The teacher then leads a mathematical discussion to highlight the variability in the responses of the different teams, and suggests that the students add up the frequencies that each team obtained in order to get a more reliable approximation of each of the probabilities.
Source: translated from Guide d’enseignement efficace des mathématiques, de la 4e à la 6e année, Traitement des données et probabilité, p. 150-151.
