B2.2 Recall and demonstrate addition facts for numbers up to 10, and related subtraction facts.
Skill: Recalling Addition Facts for Numbers up to 10 and Related Subtraction Facts
There are many strategies that can help students develop their arithmetic skills. Not all students use the full range of strategies; a few students use one, for example, the doubles strategy. Students abandon less effective strategies as they discover and create more effective ones.
In the Primary Division, students first use objects or their fingers to represent problems and then, building on these experiences, begin to use more advanced addition and subtraction strategies.
Source: translated from Guide d’enseignement efficace des mathématiques de la maternelle à la 6e année, Fascicule 5, p. 11-12.
Representation of Basic Number Facts
Representing basic number facts can help students understand basic operations and lessen their abstract nature. Many representations can be developed using the materials listed:
- moveable objects, such as counters or tiles;
- visual materials, such as pictures or arrays;
- five frames;
- ten frames;
- part-part-whole mats;
- two-coloured counters;
- a number line;
- hundred charts.
Different representations support students in making connections between drawings or pictures, symbols and words. Below are different representations for the basic fact 6 + 3. Each of the four representations is appropriate. Students will find it less necessary to use visual representations as they develop some automaticity in solving basic number facts.
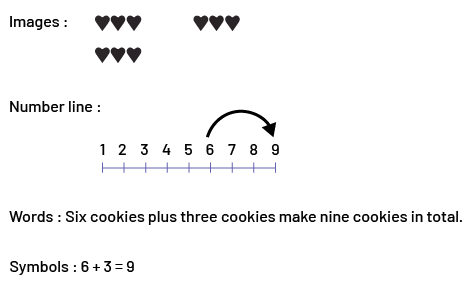
Source: translated from Guide d’enseignement efficace des mathématiques de la maternelle à la 6e année, Fascicule 5, p. 15.
Strategies for Learning Basic Number Facts
Teachers can help students develop effective strategies for knowing basic number facts by using their reasoning and encouraging them to look for patterns and relationships among numbers. The basic number fact strategies described below build on knowledge that students already have to determine unknown facts. For example, knowing 2 + 2 helps students figure out what 2 + 3 is (since 3 is 1 more than 2, the answer must also be 1 more), and is evidence of important reasoning skills. Strategies should be developed in a problem-solving context. In this regard, the teacher should:
- select problems that lend themselves to the use of the targeted strategies;
- provide opportunities for students to apply the strategy themselves;
- have students practice the strategy in a meaningful context.
Students need many experiences to become familiar with grouping by tens and to establish relationships between the number 10 and other numbers before they can develop strategies for basic number facts. Recognizing the number 10 as an anchor and defining the relationships between this benchmark and the numbers 0 to 10 is a core concept for all students. A solid understanding of how numbers are related to 10 (for example, 8 is 2 less than 10) will support students using the patterns and relationships between numbers when presented with strategies focused on basic number facts. For example, knowing the ways in which numbers are related to each other will make it easier for them to learn strategies based on decomposing numbers.
A ten frame is an important model that allows students to represent numbers from 0 to 10 concretely and supports them in seeing the relationships between 10 and other numbers. When some of the boxes in the frame are filled in, it is possible to see the empty boxes and recognize the overall number represented and how it relates to 10. Students can work with a ten frame and counters to illustrate numbers from 0 to 10. Using different coloured counters, students can identify relationships and see how different numbers relate to the number 10 and different ways to break down the number 10. For example, if there are 8 blue counters on one ten frame and 2 red counters on another ten frame, students can grasp that 8 blue counters plus 2 red counters equals 10 counters. Students can visually see the decomposition of the number 10 by changing the number of counters of each colour in a filled ten frame.
The strategies below are not presented in any particular order. Some students may find some strategies more useful than others, or may ignore some strategies in favour of their own. Others may find it easier to memorize facts than to rely on a strategy. Whatever the case, the teacher's primary goal is to get students to fully understand addition and subtraction.
1 More and 2 More
For the basic number facts 5 + 1 = 6, 5 + 2 = 7, 7 + 1 = 8, 7 + 2 = 9, one of the terms in each addition is 1 or 2. The strategy is based on the assumption that students easily remember the number that follows the larger number, or can easily count on from it.

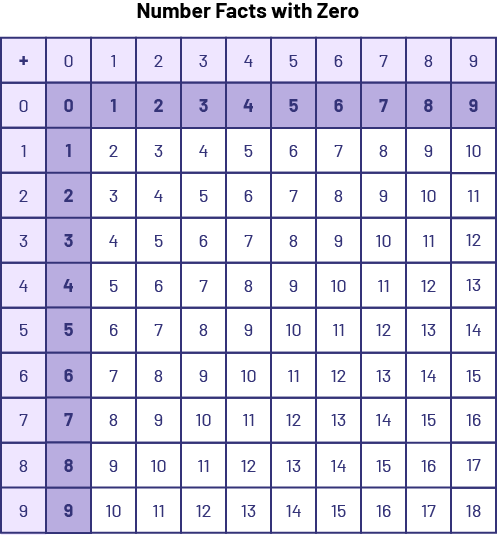
Math Facts With Zero (1 + 0 = 1, 1 - 0 = 1)
For these basic addition and subtraction facts, zero is one of the terms. Students often overgeneralize by thinking that the answer to addition is necessarily larger and the answer to subtraction is necessarily smaller. Understanding of the concept of the identity property in addition and subtraction can be reinforced through games using flashcards and numbered cubes.
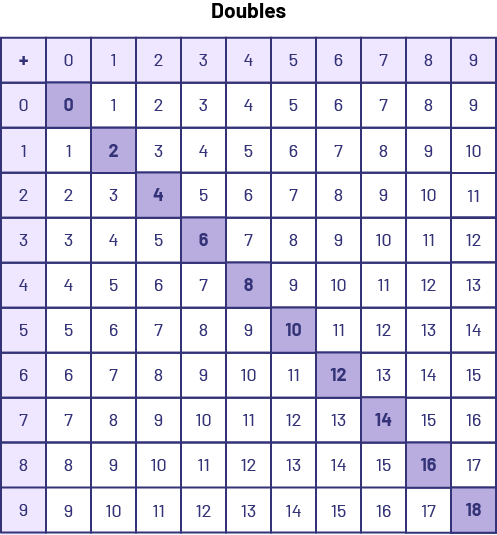
Doubles
For these basic number facts, the first term and the second term (or both terms) are the same. It is beneficial for students to recognize and learn about doubles. There are only ten number facts related to doubles. Simple memorization tricks can be used to learn several doubles:
Double of 3: an insect has 3 legs on one side and 3 legs on the other (6).
Double of 4: a spider has 4 legs on one side and 4 legs on the other (8).
Double of 5: the 5 fingers of one hand and the 5 fingers of the other (10).
Double of 6: an egg carton with 6 eggs on one side and 6 on the other (12).
Double of 7: on the calendar, there are 7 days in a week and 14 days in two weeks.
Double of 8: There are 8 crayons in one row of a crayon box and 16 crayons in two rows.
Doubles plus 1 (5 + 4 can be seen as 4 + 4, and 1 more)
For these basic number facts, one term of the addition is 1 more than the other term. Students can learn to recognize that in these additions, the answer is the same as the double of the lesser number plus 1 (5 + 4 = 4 + 4 + 1)). Students need to be proficient with doubles before they can use this strategy effectively.
Commutative Property (1 + 2 = 2 + 1)
Students who recognize the commutative property of addition can cut the amount of number facts they need to learn in half. Visual representation of facts such as 3 + 2 and 2 + 3 helps students grasp this relationship.
Subtraction as the Inverse Operation of Addition for Basic Math Facts with a Sum up to 10
Students who know their addition well and understand that subtraction is the inverse operation of addition can use this knowledge to master the related subtractions (if 5 + 2 = 7, it follows that 7 - 5 = 2). For example, consider the following problem: "Julian has 6 marbles in his bag. George gives him some more. Julian now has 10 marbles. How many marbles did George give to Julian? Faced with this problem, the student thinks: "What number added to 6 gives 10? Thus, the addition facts with which he is familiar help him to find the unknown term in the number sentence.
1 Less and 2 Less
These subtraction facts have one term of 1 or 2 less than the other term. Students can usually count backwards for the easiest number facts, such as any number from which 0, 1, or 2 is subtracted.
Source: translated from Guide d’enseignement efficace des mathématiques de la maternelle à la 6e année, Fascicule 5, p. 14-20.
