B1.6 Use drawings to represent and solve fair-share problems that involve 2 and 4 sharers, respectively, and have remainders of 1 or 2.
Activity 1: Equal Sharing of Objects Between Two People
Show students the following illustration.
Form groups of two. Ask each group to divide the number of marbles equally using manipulatives or collections of objects.
Circulate among the students asking them questions such as:
- What do we notice?
- How can the marbles be divided so that each person receives the same amount? What strategies can be used?
- When the number of marbles is divided equally, are there any marbles left? If so, what can be done with the remaining marble? Explain your reasoning.
Activity 2: Equal Sharing of Objects Among Four People
Jamila, Fadila, Chafi and Nazim's parents are preparing snacks for the week. They bought a box of 18 granola bars. They plan to put one granola bar per day in each child's lunch bag. How can they share the bars fairly?
Strategy 1
The student uses rectangles to show the fair division of 18 granola bars among the 4 children in the family.
First, the student gives 4 whole granola bars to each child. Two bars remain to be shared equally. The student divides each rectangle into two equal parts (squares) and gives one half to each child. So, for a fair split, each child gets 4 bars and one half.
Strategy 2
The student can distribute the 18 bars while ensuring an equitable division among the 4 children. The children will each have 4 bars. There are two bars left to share. The student can then decide to divide the last two bars in half to create an extra half bar for each child. Thus, each child will have 4 and a half bars.
Strategy 3
The student can use 18 tokens to represent the bars. They can distribute the tokens equally among the 4 children. Each child in the family then receives 4 tokens. There are two left. Since we can cut one granola bar in half, the two remaining bars can be divided equally among the 4 children. Each child will then have 4 and a half bars.
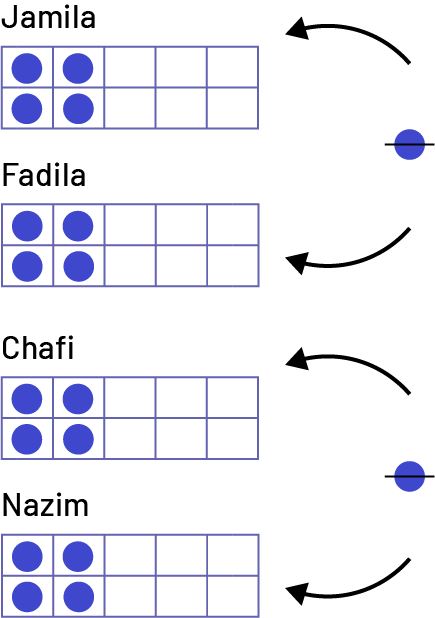
Ask questions such as:
- When comparing different student strategies, what do you notice?
- Is it possible to divide the 18 bars equally among the 4 children? Are there any bars left? How do you know?
- If there are any bars left, how can they be divided fairly? Explain your approach.
- Using words, how can you describe the shares resulting from the division?