B2.3 Use mental math strategies, including estimation, to add and subtract whole numbers that add up to no more than 50, and explain the strategies used.
Activity 1: "Human Calculator" Game
Invite a student to play the role of the "human calculator". Students in this role stand at the front of the class in front of a number chart reproduced on a large sheet of paper (a chart projected on the interactive whiteboard can also be used). Other students use a regular calculator.
Have students enter a number, such as 35, into the calculator.
Ask the "human calculator" to point to 35 on the number chart. The goal is to see who will go faster, the "human calculator" or the students using a calculator.
Have the "human calculator" and the other students add 15 to the original number and give the answer as soon as it appears on the calculator screen. The "human calculator" moves their finger vertically one row down, from 35 to 45, and horizontally five columns to the right to 50.
More often than not, the "human calculator" wins out. Students can take turns playing the role of the human
calculator.
This activity can be done first with the class and later in small groups.
Ask students increasingly difficult questions, such as 35 + 10, 11, 12, 20, 30, and so on.
Make cards. Write the starting number on one side of the card and the number to be added on the other side. The
"human calculator" puts her finger on the starting number and the other students enter that number into
their calculators.
Turn the card over to show students the number to add. Do not write the entire question on the same side of the card,
as students using a calculator may be faster.
This activity gets students used to adding by tens and not by ones.
Activity 2: The Mystery Number
Materials
- Appendix 2D.1 (one copy per student)
- tokens (one per student or per team)
Give a copy of Appendix 2D.1 and a token to each student or pair.
Have students place their token on a starting number, such as 54.
Draw an arrow or a series of arrows on a blank grid.
Invite students to move their tokens, one square at a time, in the order of the arrows to determine the mystery number chosen by the teacher.
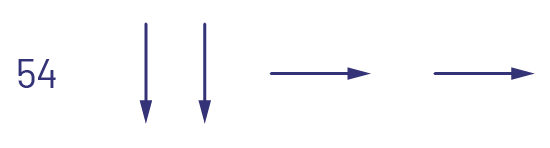
Note: The first arrow indicates that you need to move down one square, increasing the number by 10, to reach 64. The second arrow indicates that you should move down another square to 74. The third arrow indicates that you should move one square to the right to 75. The last arrow indicates that you must move another square to the right to get to 76, which is the mystery number in this case. The first trial may require a demonstration on a number mat or number chart.
Have students choose a mystery number, write it on a piece of paper, and provide clues in the form of arrows for their partner to find the number.
Ask students to write the number sentence that corresponds to the path taken to discover the mystery number (for example, (54 + 10 + 10 + 1 + 1 = 76)).
Activity 3: The Useful Line
Draw a line on the board with nothing but the first term of an operation.
Tell students that an open number line can be used to visually represent what is going on in our heads during a mental calculation.
Simple example
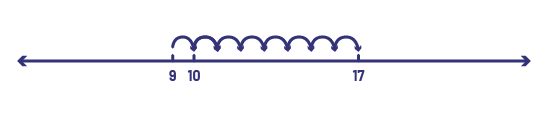
Ask students to perform the operation (9 + 8).
Allow students time to think and then collect their suggestions; for example, a student might say: I know that (9 + 1 = 10). I then added 7 by counting 11, 12, 13, 14, 15, 16, 17, so the answer is 17.
More complex example
Suggest to students an operation such as (44 - 18).
Allow students time to think and then collect their suggestions; for example, a student might express their idea as follows: Starting at 44, I went back 10 to get to 34. Then I went back 4 to 30, then 4 more to 26.
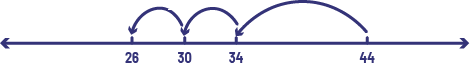
Source: Guide d'enseignement efficace des mathématiques de la 1re à la 3e année, p. 144-145.