B1.9 Describe relationships and show equivalences among fractions and decimal tenths, in various contexts.
Activity 1: The Clothesline
Write fractions, mixed numbers, whole numbers, and decimal numbers on flash cards. Place two cards on a clothesline (or number line). Have a student randomly select a flash card and place it on the clothesline (or number line), paying attention to the order and space between the numbers. When the student places their card, they must justify their choice of location and answer any questions that may be asked. Continue the activity by inviting other students to take turns doing the same thing.
Source: Guide d'enseignement efficace des mathématiques de la 4e à la 6e année, p. 159.
Activity 2: The Chocolate Bar
Share the following situation with students:
You have a chocolate bar and you have to share tenths of that chocolate bar equally among your friends. You can't cut the tenths. How can you distribute the tenths? How many friends can you share with?
Invite students to come up with various solutions using concrete or semi-concrete representations and to write, in fractions and decimal numbers, the portion of the chocolate bar shared.
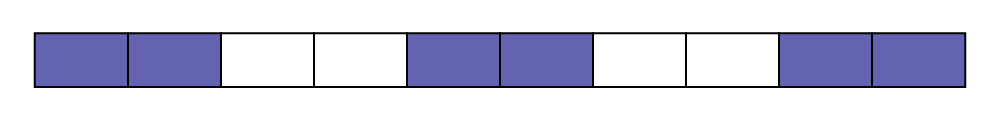
Example
\(\frac{2}{{10}}\) or \(\frac{1}{5}\) or 0.2 of the chocolate bar is shared among 5 friends.
\(\frac{1}{{10}}\) or 0.1 of a chocolate bar is shared among 10 friends.
\(\frac{5}{{10}}\) or 0.5 of the chocolate bar is shared between 2 friends.
Activity 3: Decimal Number Bingo
Prepare cards with numbers from one-tenth to nine-tenths written on them in letters, decimal notation, and fractional notation. Place the cards in a bag.
Ask students to fill in a \(3\; \times \;3\) grid with numbers from one tenth to nine tenths of their choice, written in either letter, decimal, or fractional notation.
Example
Remove a card from the bag and read it to the students. Explain that if one of the three representations of this number is on their grid, they must cover it with a token. If there is more than one representation of that number on their grid, they must cover only one of them. Explain that the game continues until a student has covered all the squares on their grid, at which point they win the game.
Source: Guide d'enseignement efficace des mathématiques de la 4e à la 6e année, p. 144-145.