B1.4 Compare and order fractions from halves to twelfths, including improper fractions and mixed numbers, in various contexts.
Activity 1: Comparing Fractions
Write on the board a set of fractions between 0 and 2 (for example, \(\frac{5}{6},\;\frac{5}{8},\;\frac{4}{4},\; \frac{5}{3},\;\frac{9}{{10}},\;1\frac{2}{3},\;\frac{8}{5},\;\frac{ 3}{5}\)).
Invite students to sort these numbers as follows: those that are close to 0, those that are close to \(\frac{1}{2}\), those that are close to 1, and those that are close to 2. Then, focusing on fractions that are close to \(\frac{1}{2}\), ask them to name those that are greater than \(\frac{1}{2}\) and those that are less than \(\frac{1}{2}\).
Then draw a number line, locating the numbers \(0,\;\frac{1}{2},\;1,\;1\frac{1}{2}\;\rm{and} \ 2\), and invite students to come and locate the numbers written on the board on this number line, explaining their decision.
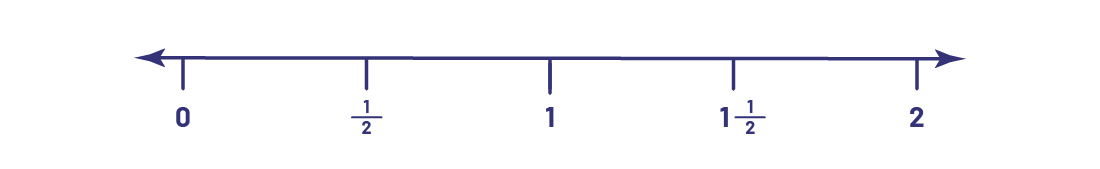
Repeat the exercise using other classification criteria (for example, between 0 and 1, between 1 and 2) or using other numbers.
Source: Guide d’enseignement efficace des mathématiques de la 4e à la 6e année, p. 163.
Activity 2: Which Key to Choose?
This activity allows students to examine the use of fractions to define the measurements of tools (wrenches, bolts, nuts).
Materials
- bolts and nuts of various sizes previously arranged;
- set of wrenches identified in imperial measurement (examples of the most common measurements: \(\frac{1}{4}\) inch, \(\frac{3}{8}\) inch, \(\frac{1} {2}\) inch, \(\frac{5}{8}\) inch, \(\frac{3}{4}\) inch).
Directions
Teachers invite students to sit in a circle around the materials on the floor. The teacher explains to the students that the metric system is the system of measurement generally used in Canada, but that in some areas such as construction, the imperial system is still commonly used.
The teacher then shows the students a wrench with a fraction on it and explains that the measurement of the opening of the wrench is a fraction of an inch. For example, the wrench with the fraction \(\frac{3}{4}\) on it has an opening of \(\frac{3}{4}\) inch. If necessary, they may point to an inch on a ruler or tape measure and explain that an inch is a measurement representing approximately 2.5 cm. Next, they explain that the wrench is the tool used to tighten a nut and that it is important to ensure that the measurement of the wrench opening matches the width of the nut.
The teacher presents students with fractions corresponding to the wrenches and nuts brought in. Fractions can be written on the board or on a large piece of paper.
Next, the teacher chooses a nut (partially threaded onto a bolt), passes it among the students, and asks them to estimate its width in inches to determine which wrench should be used to screw or tighten it.
The teacher invites the student to try to tighten the nut in question using the wrench of their choice. If the wrench does not fit the nut precisely, the student should indicate whether the chosen wrench is too big or too small. For example, the student (pictured below) explains that he chose the \(\frac{5}{8}\) inch key, but it is a bit too big.
If the first attempt is unsuccessful, students try again to determine which wrench should be used. Teachers invite other students to try until the correct wrench for the nut is found, then repeat the process with other nuts.
Throughout the activity, teachers encourage students to compare fractions (for example, the opening of the \(\frac{3}{4}\) inch wrench is larger than that of the \(\frac{3}{8}\) inch wrench) and to justify their choice of wrench (for example, "During the last test, the \(\frac{1}{2}\) inch wrench was too big; so I'm going to try the \(\frac{3}{8}\) inch wrench, since \(\frac{3}{8}\) of an inch is a little less than \(\frac{1} {2}\) of an inch”).
To complete the activity, teachers ask students to place the fractions in ascending order (for example, \(\frac{1}{4}\), \(\frac{3}{8}\), \(\frac{1}{2}\), \(\frac{5}{8}\), \(\frac{3}{4}\)) and verify their answer by placing the corresponding wrenches in order of size.
Note: To make the task more authentic, instead of loose nuts and bolts, a bicycle (or other object that includes several nuts) can be presented and students can be asked, for example, to identify which wrench to use to loosen the nut to mount the seat or to remove the wheel.
Source: Guide d'enseignement efficace des mathématiques de la 4e à la 6e année, p. 105-106.
Activity 3: In Order
Materials
- Appendix 5.4 (Fractions)
Group students into groups of 4. Give each group a copy of Appendix 5.4 and point out that the shaded area represents a fraction of the strip. Ask them to cut between the strips so that they have a set of strips, each with the corresponding fraction.
Note:Invite students to combine strips to create mixed numbers or improper fractions. Students may also create their own strips.
Example
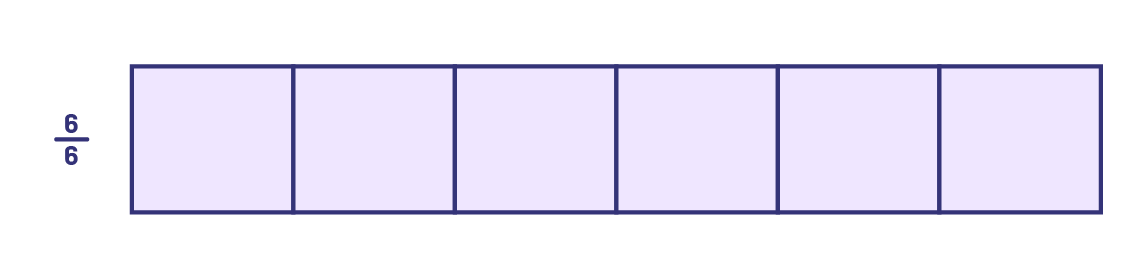
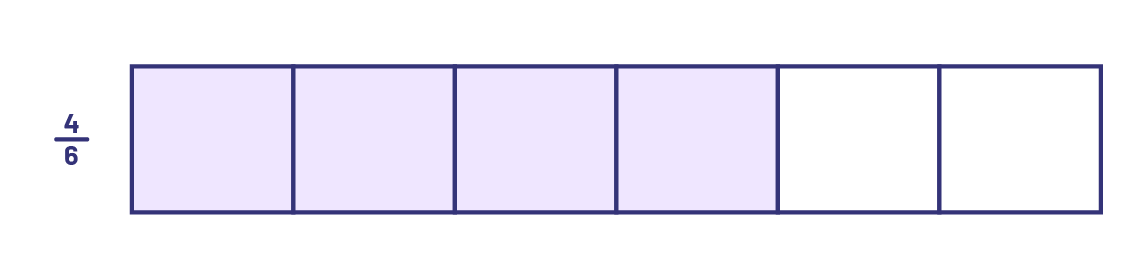
The student creates the mixed number \(1\frac{4}{6}\) or the improper fraction \(\frac{{10}}{6}\).
Then specify that their task is to place the fractions in ascending order (smallest part to largest part).
Circulate and question students to check for understanding. Ask questions such as:
- Why did you place this fraction in this location?
- How do the numbers in the numerator and denominator help you place fractions in correct order?
- Some fractions represent the same quantity. What does this mean? How did you know where to place them? How can you justify that they are equivalent?
When the task is complete, have a mathematical discussion to emphasize the strategies used to determine the ordering of the fractions. Next, have students observe unit fractions (for example, \(\frac{1}{2},\;\frac{1}{3},\;\frac{1}{4},\;\frac{1}{6},\;\frac{1}{8},\;\frac{1}{9},\;\frac{1}{{12}}\)), more particularly their numerator and their denominator, to emphasize that when fractions have the same numerator, one having a larger denominator represents a smaller part. Then, ask them to compare all the fractions with the same denominator (for example, \(\frac{1}{8},\;\frac{2}{8},\;\frac{3}{8} \ldots \)) to emphasize that when fractions have the same denominator, one with a larger numerator represents a larger part. Finally, invite them to analyze equivalent fractions in order to recognize the relationship of proportionality.
Source: Guide d'enseignement efficace des mathématiques de la 4e à la 6e année, p. 160.