B2.1 Use the properties of operations, and the relationships between operations, to solve problems involving whole numbers and decimal numbers, including those requiring more than one operation, and check calculations.
Activity 1: Triplets
Strategy: Commutative Property (Addition or Multiplication)
Materials
- a set of Triplets Addition Cards (Appendix FR15 and Appendix FR16) or prepare addition cards according to grade level
- a set of Triplets Cards for Multiplication (Appendix FR17 and Appendix FR18) or prepare multiplication cards according to grade level
This activity is done with the whole class. Each student receives one card from the Triplets game. Once the cards have been dealt, the students look for the other members of their trio. They need to know the answer to the question, or consider the possible questions to which their card has the answer. For example, the triplets could be \(3\; + \;5,\;5\; + \;3\;{\rm{and}}\;{\rm{8}}\); or \(3\; \times \;6,\;6\; \times \;3\;{\rm{and}}\;18\). When a triplet is complete, the three students who have the cards sit together. Once all the students are seated, each trio presents the cards to the class.
Source: translated from Guide d'enseignement efficace des mathématiques de la maternelle à la 6e année, Fascicule 5, p. 78.
Activity 2: True or False?
To explore the properties of operations, facilitate a mini-lesson by grouping students together and writing these number sentences one at a time. For each, ask students to determine whether the sentence is true or false.
For example, for the distributive property, write these sentences one at a time.
\(47\; \times \;21\; = \;\left( {40\; \times \;20} \right)\; + \;\left( {40\; \times \;1} \right)\; + \;\left( {7\; \times \;20} \right)\; + \;\left( {7\; \times \;1} \right)\)
\(92\; \times \;34\; = \;\left( {90\; \times \;30} \right)\; + \;\left( {90\; \times \;4} \right)\; + \;\left( {2\; \times \;30} \right)\; + \;\left( {2\; \times \;4} \right)\)
\(\left({40\; \times \;50} \right)\; + \;\left( {40\; \times \;0} \right)\; = \;2000\)
Activity 3: What Do You See?
Show students a picture of objects (for example, strawberries) placed in an array.
Example
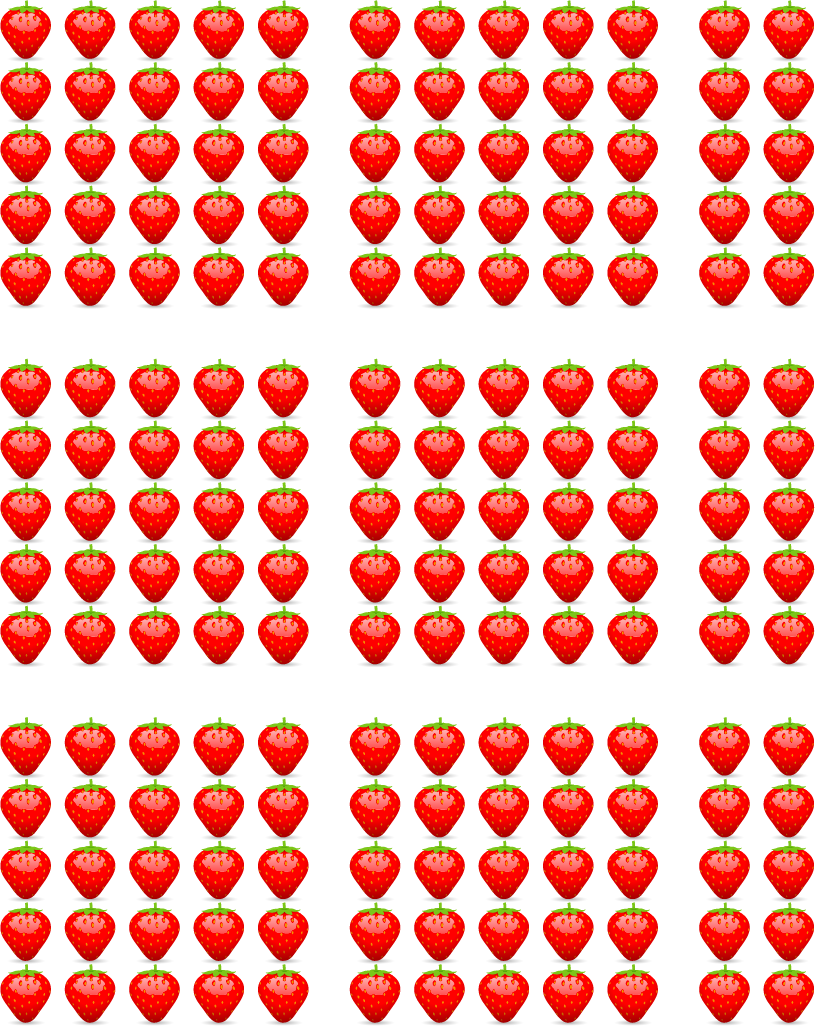
Ask students to work in pairs to determine the total number of strawberries using operations.
For example: \(15\; \times \;12\; = \;180\)
Pair up the pairs so that students can compare their operations.
Pool the results to identify properties and relationships.
Examples
Commutative Property
\(12\; \times \;15\; = \;180\)
\(15\; \times \;12\; = \;180\)
Distributive Property
\(12\; \times \;15\; = \;\left( {10\; \times \;15} \right)\; + \;\left( {2\; \times \;15} \right)\)
\(12\; \times \;15\; = \;\left( {10\; \times \;10} \right)\; + \;\left( {10\; \times \;5} \right)\; + \;\left( {2\; \times \;10} \right)\; + \;\left( {2\; \times \;5} \right)\)
Inverse Relationship of Division and Multiplication
\(12\; \times \;15\; = \;180\)
\(180\; \div \;15\; = \;12\)
\(15\; \times \;12\; = \;180\)
\(180\; \div \;12\; = \;15\)
Activity 4: A Beautiful Zero
Strategy: Identity principle and the Zero Property (in multiplication)
Materials
- Appendix FR20 (Spinning for Zero spinners [+ 0, - 0, + 1, × 0, × 1]), or a spinner according to the level of study
- Appendix FR21 (Spinning for Zero Game Sheets) (1 per student)
- counters
Students are given a blank game sheet and write the numbers from 0 to 8 in the boxes, repeating them as they wish, until all the boxes are filled in. They then work in groups of two, three or four. The student who opens the game spins the two spinners and performs the operation indicated. If the box indicating the answer is still empty, the student places a counter on the number. It is then the next student's turn. If there is already a counter on the number, the next student plays. The game continues until a student has a counter in every box in one row of their game sheet.
Source: translated from Guide d'enseignement efficace des mathématiques de la maternelle à la 6e année, Fascicule 5, p. 80.
Activity 5: Related Operations
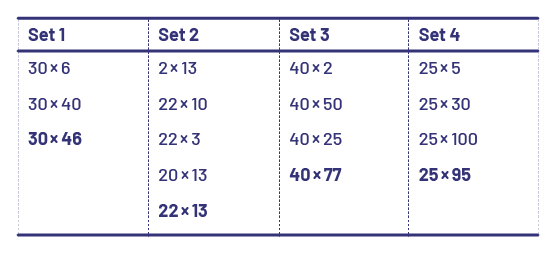
Write each of the following sets of related operations on the board or on chart paper, one at a time, and have students perform the operations.
Once a set is completed, draw out the various mental math strategies by asking questions such as:
- How did you perform the last operation?
- To perform the last operation, how did the previous calculations help you?
- How would you describe the order in which you performed the operations?
If necessary, before doing the same with the next set, present other examples of similar related operations.
If students have difficulty seeing and applying the distributive property of multiplication to solve the last operation in each set, support them to do so by representing these operations using an area model.
Example
Findings for each set
Set 1
This set demonstrates a simple application of the distributive property of multiplication over addition. The idea is to recognize that to obtain the product of \(30 \times 46\), it is possible to perform x30 on the decomposed parts and then sum the results:
\(30\; \times \;46\; = \;30\; \times \;\left( {40\; + \;6} \right)\)
\(30\; \times \;46\; = \;\left( {30\; \times \;40} \right) + \;\left( {30\; \times \;6} \right) \)
Set 2
This set demonstrates that when using the distributive property, decomposition can be performed on either term:
\(\begin{array}{l}22\; \times \;13\; = \;\left( {20\; \times \;13} \right) + \;\left( {2\; \times \;13} \right)\;{\rm{or}}\\22\; \times \;13\; = \;\left( {22\; \times \;10} \right) + \;\left( {22\; \times \;3} \right)\end{array}\)
Set 3
This set demonstrates how decomposing a number to apply the distributive property can be done in more than two parts. For example:
\(40\; \times \;77\; = \;40\; \times \;\left( {50\; + \;25\; + \;2} \right)\)
\(40\; \times \;77\; = \;\left( {40\; \times \;50} \right)\; + \;\left( {40\; \times \;25} \right)\; + \;\left( {40 \times \;2} \right)\)
Set 4
This set demonstrates how we can decompose a term using subtraction and then apply the distributive property of multiplication. For example:
\(25\; \times \;95\; = \;25\; \times \;\left( {100\; - \;5} \right)\)
\(25\; \times \;95\; = \;\left( {25\; \times \;100} \right) - \;\left( {25\; \times \;5} \right) \)
Source: adapted and translated from Guide d'enseignement efficace des mathématiques de la 4e à la 6e année, Numération et sens du nombre, Fascicule 1, Nombres naturels, p. 221-222.
