B1.5 round decimal numbers, both terminating and repeating, to the nearest tenth, hundredth, or whole number, as applicable, in various contexts
Skill: Rounding Decimal Numbers, Both Terminating and Repeating, to the Nearest Tenth, Hundredth, or Whole Number
In most real-life situations, it is not necessary to work with a precise number, since an approximation is just as valid and often more convenient. Junior students need to be able to round using their number sense, which requires analysis and reflection.
A number can be rounded to a pre-determined place value. For example, when purchasing an item for $11.34, the provincial sales tax of 8% is calculated to be $0.907 2. However, we will pay $0.91 because the amount is always rounded to the nearest hundredth of a dollar since thousandths and ten-thousandths of a dollar are not part of our coins.
Students should be presented with a variety of problems that encourage them to think about the effect of rounding on quantity and to choose how to round. Should they round to the nearest one, the nearest tenth, or the nearest hundredth? The choice depends on the context, the meaning of the number and the reasons for rounding. For example, a restaurant owner who has tables that are 2.27 m long may, when purchasing tablecloths, round the length to 2.3 m or even 2.5 m to be sure that the tablecloths purchased will be long enough. However, when talking to her employees, she can refer to it as the 2 m table. In short, it is only after an analysis of the context that one can determine how to do the rounding or at what position the rounding should be done.
Reading numbers on a calculator is an essential skill, especially since division with a calculator often results in a quotient with a very long decimal part. In this case, to better understand the quantity displayed, it may be helpful to round a number (for example, round 0.248,953 2 to 0.25) or replace it with a landmark fraction (for example, 0.248,953 2 is about \frac{1}{4}\).
Unfortunately, rounding is too often taught using methods that are meaningless because they deal with numbers and not quantity. For example, to round a decimal number to the nearest tenth, students are taught to identify the digit in the position to be rounded and then consider the digit that follows it. If it is greater than or equal to 5, the identified digit is increased by one and the digits that follow are eliminated.
Example:
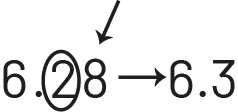
Research findings indicate that these traditional rounding methods do not develop the concept of approximation in students.
Note: Since rounding a decimal number requires students to use their decimal number sense, it is important that they have had the opportunity to develop it by representing decimal numbers, placing them on a number line and comparing them.
Example:
To round \(0,\overline 6 \) to the nearest unit, students recognize that the number \(0,\overline 6 \) represents a quantity equivalent to "a little less than 1". They then know that the number is between 0 and 1. They then use their number sense to determine whether \(0,\overline 6 \) is closer to 0 or 1. They can, for example, using a reference number representing the middle of the interval (0.5), "seeing" that \(0,\overline 6 \) is close to 1 or even visualizing the location of \(0, \overline 6 \) on a number line.
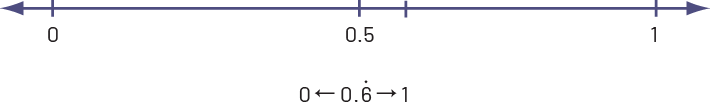
Whichever element of number sense is chosen, students are then able to round \(0,\overline 6 \) to 1. If they decide to round to the nearest tenth instead, they should use their understanding of the infinite, periodic quantity represented by this number and recognize that \(0,\overline 6 \) represents a quantity that lies between 0.6 and 0.7.
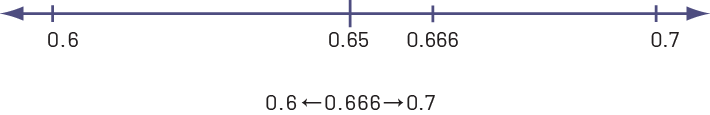
By visualizing the number 0.6 on a straight line, students can conclude that 0.7 is closer to 0.6 than to 0.7 and therefore round up to 0.7.
Source: A Guide
to Effective Instruction in Mathematics, Grades 4 to 6, p 42-44.
Knowledge: Decimals
A number expressed in decimal notation is composed of two parts, namely the integer part and the decimal part.
Other numbers are also written in decimal notation. For example, the number is written as 3.141 592 65… and the number is written as 0.333 3…or 0.333 overline 3. However, since these numbers are not composed of a finite decimal part, they are not decimal numbers. Instead, they are grouped together with decimal numbers under the term decimal numbers, since the decimal point (,) is the symbol chosen to separate the integer part from the decimal part. In a decimal number, the decimal part can be finite, infinite and periodic or infinite and non-periodic.
Note: In English, the dot (.) is used to separate the integer part from the decimal part.
Terminating decimal
The decimal part contains a finite number of digits.
Note: Numbers with a terminating decimal part can be represented by decimal fractions.
Example
\(0,5\;\left( {\frac{5}{{10}}} \right)\)
\(1,458\;(\frac{1458}{1000})\)
Non-terminating and repeating decimals
The decimal part contains an infinite number of digits of which a part (the period) repeats indefinitely.
The period is indicated by a horizontal line placed above the repeated digit or group of digits.
Note: Numbers with non-terminating and repeating decimal parts can all be represented by fractions.
Example
\[0,353\;535...\;{\rm{ou}}\;0,\overline {35} \left( {\frac{{35}}{{99}}} \right)\]
\[0,666...\;{\rm{ou}}\;0,\overline 6 \left( {\frac{2}{3}} \right)\]
Source: A Guide to Effective Instruction in Mathematics, Grades 4 to 6, p. 29.