B2.10 Divide whole numbers by proper fractions, using appropriate tools and strategies.
Skill: Dividing Whole Numbers by Proper Fractions, Using Appropriate Tools and Strategies
The exploration of division, like that of other operations, should focus on concrete and visual (semi-concrete) representations and not on algorithms. In order to understand division, one needs to know the type of situation and the nature of the numbers involved. A division is the sharing type when we need to find the size of the groups; it is the grouping type when we need to find the number of groups.
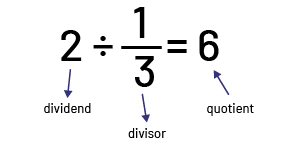
Thus, dividing a whole number by a fraction (for example, \(2\; \div \;\frac{1}{3}\)) is well represented using grouping. In this case, the fraction is the divisor.
For example, if we have 2 licorice chews and we want to give \(\frac{1}{3}\) of the licorice to each child, we need to separate or measure out one quantity (2 licorice chews) into equal quantities, or groups. In this case, we can make 6 groups of (\(\frac{1}{3}\), so 6 children will receive \(\frac{1}{3}\) of the licorice. In this case, it is important to recognize that the quotient expresses a number of groups, not a quantity of objects (licorice chews).

Source: translated from Guide d’enseignement efficace des mathématiques de la 4e à la 6e année, Numération et sens du nombre, Fascicule 2, Fractions, p 79-82.
In the case of a division of a whole number by a fraction, division usually takes on the quotative meaning. Thus, the analogy of repeated subtraction is in order since it involves separating parts.
For example, in dividing 2 by \(\frac{1}{4}\)(\(2\; \div \;\frac{1}{4}\)), doing \(2\; - \;\frac{1}{4}\; - \;\frac{1}{4}\; - \;\frac{1}{4}\; - \;\frac{1}{4} \; - \;\frac{1}{4}\; - \;\frac{1}{4}\; - \;\frac{1}{4}\; - \;\frac{1}{ 4}\), we can create 8 groups of \(\frac{1}{4}\). However, the group created is rather abstract since it is a group that is a fraction of a whole. The questions "How many \(\frac{1}{4}\) can be created with 2 wholes?" and "How many times does \(\frac{1}{4}\) go in 2?" can help visualize the operation.
At the beginning of learning, it is possible to explore situations with unit fractions (for example, \(4\; \div \;\frac{1}{3}\; = \;12\); \( 2\; \div \;\frac{1}{5}\; = \;10\); \(3\; \div \;\frac{1}{4}\; = \;12\)) before discussing operations with a non-unit fraction. In both situations, it is recommended that the quotient be a whole number (for example, \(6\; \div \;\frac{3}{5}\; = \;10\); \(6\; \div \;\frac{2}{3}\; = \;9\)).
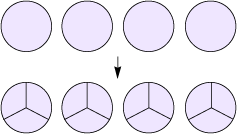
Example
\(4\; \div \;\frac{1}{3}\; = \;12\)
Area Model
How many pieces of \(\frac{1}{3}\) pie can be offered if there are 4 pies and each piece is \(\frac{1}{3}\) of one pie?
Linear Model
Mathieu wraps gifts in small boxes. He needs \(\frac{1}{3}\) of a metre of ribbon to make one decorative bow. If there are 4 metres of ribbon, how many bows can he make?
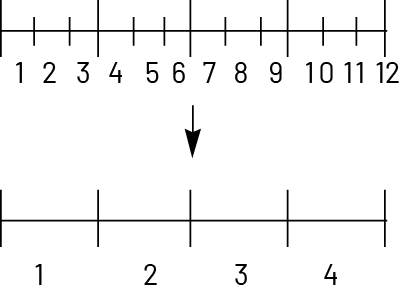
Source: translated from Guide d’enseignement efficace des mathématiques de la 4e à la 6e année, Numération et sens du nombre, Fascicule 2, Fractions, p. 101.
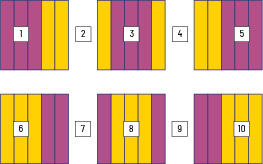
Example
\(6\; \div \;\frac{3}{5}\; = \;10\)
Area Model
In order to create a tessellation, each team needs the equivalent of \(\frac{3}{5}\) of a sheet of paper. How many teams can complete the task if we have 6 sheets?
Linear Model
A teacher has a 6 m rope and wants to cut it into sections of \(\frac{3}{5}\;\) of a metre each. How many sections will she be able to create?

Source: translated from Guide d’enseignement efficace des mathématiques de la 4e à la 6e année, Numération et sens du nombre, Fascicule 2, Fractions, p. 102.
