B1.4 Use fractions, decimal numbers, and percents, including percents of more than 100% or less than 1%, interchangeably and flexibly to solve a variety of problems.
Skill: Describing Relationships and Representing Equivalences Among Fractions, Decimal Numbers to Thousandths, and Percents, Using Appropriate Tools and Diagrams, in a Variety of Contexts
In order to develop number sense, it is important that students be able to visualize the quantities represented by the numbers. In the case of decimal numbers, reading them correctly allows students to form better mental representations and to draw on their knowledge of fractions (for example, 0.75 is read as "75 hundredths", not "0 point 75" ). Students should be encouraged to use multiple models to help create various mental representations to describe the relationships between fractions and decimals.
Example
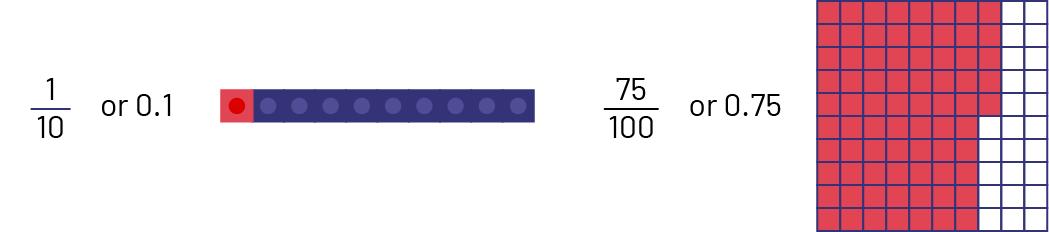
When representing decimal numbers using models, there is an adjustment to be made since these same models were previously used differently (for example, the rod represented ten ones). Students must understand that the unit (for example, the value assigned to a cube) has changed. In the first of the two previous examples, the rod is the whole; in the second, the large square represents the whole.
Students must also form a mental representation of decimal numbers greater than one. When reading such a decimal number, they must mentally represent the quantity it represents by interpreting the 2 parts that make it up: the integer part and the decimal part. For example, they must recognize that the number 8.24 represents 8 integers and a part of another identical integer. They can then visualize a quantity between 8 and 9.
Example

Over time, students are able to mentally picture the quantity in context. For example, in a situation involving an item that costs $197.98, a student who recognizes that $197.98 is a little more than $197.00 can then visualize or conceive that, in this context, the amount of $197.98 can be represented approximately by 10 $20 bills.
Students need to be able to create a mental representation of a percent, as they do with decimal numbers. The very nature of a percent makes it easier for them to visualize a quantity, as it is always a ratio to 100. It should also be understood that a percent is another way of representing a quantity.
Example
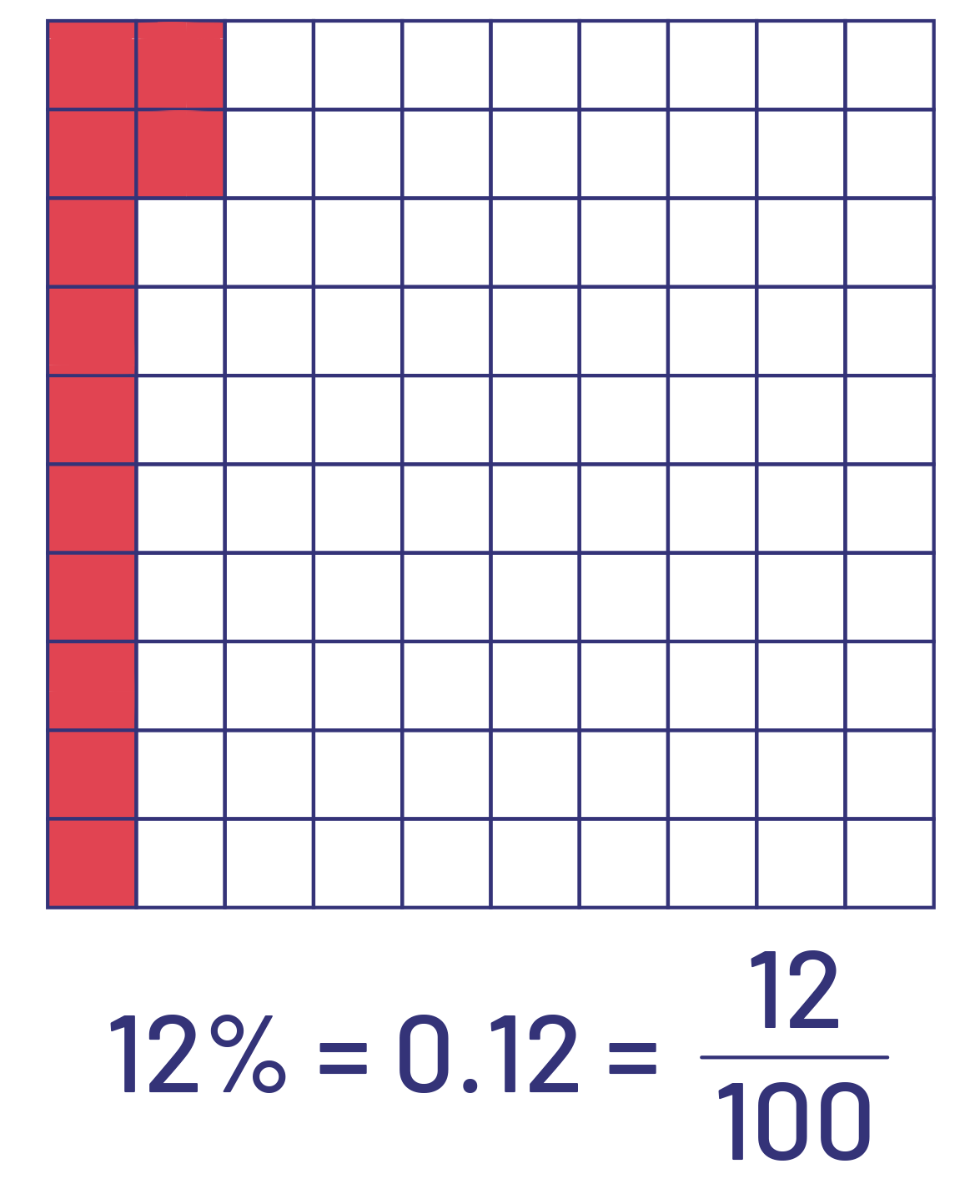
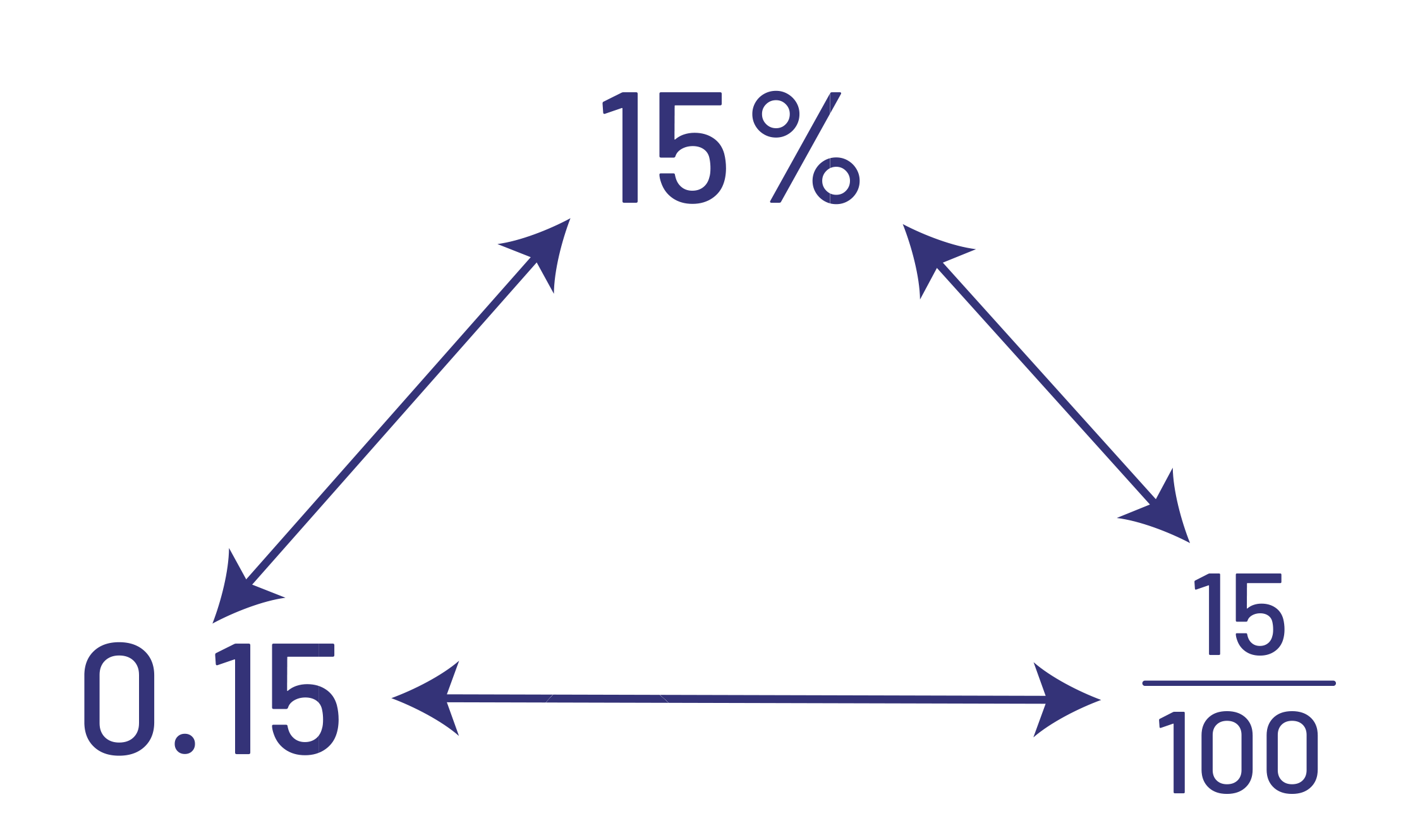
It is important to recognize the equality between various representations of numbers or numerical expressions. In the context of decimal numbers, students should recognize the equality between a decimal number, the corresponding decimal fraction, and the percent.
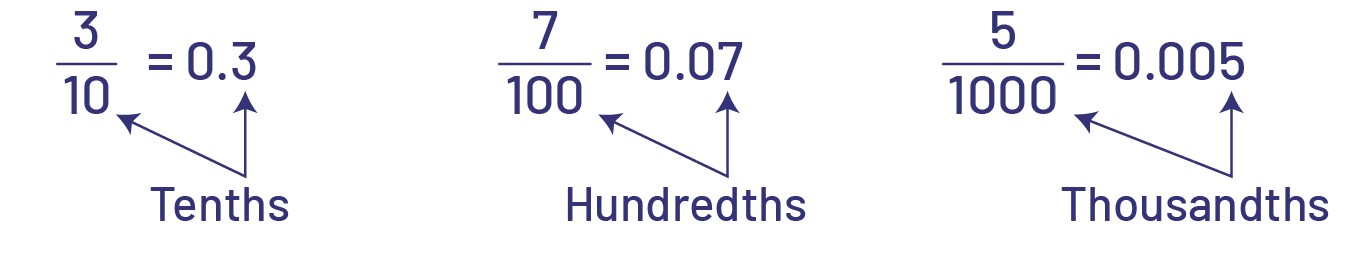
Students need to understand that since decimal notation is just another way of representing a decimal fraction (fractions with a denominator that is a power of 10), it is then possible to establish a relationship of equality between the two notations (for example, 0.3 = \(\frac{3}{{10}}\). By recognizing this equality, they are able to associate a place value with each of the digits that make up a decimal number, successively tenths, hundredths, thousandths and so on.
Example
Students who do not understand this association are sometimes inclined to represent a fraction such as \(\frac{2}{5}\) by 0.2 or 0.25. The following description describes observable behaviours of students who have developed a conceptual understanding of numbers and the relationship between their different representations.
Conceptual Understanding of Numbers
Number: 0.3
Observable behaviours:
- The student reads it as three tenths.
- The student can write the number in fractional notation as \(\frac{3}{{10}}\).
- The student can represent the number using concrete materials or visuals.
Example
Number: \(\frac{{16}}{{100}}\)
Observable behaviours:
- The student reads it as sixteen hundredths.
- The student can write the number in decimal notation as 0.16.
- The student can represent the number using concrete materials or visuals.
Example
Number: \(\frac{2}{7}\)
Observable behaviours:
- The student reads it as two sevenths.
- The student knows that the fraction \(\frac{2}{7}\) is not represented by 0.2 since they know that \(0.2\; = \;\frac{2} {{10}}\).
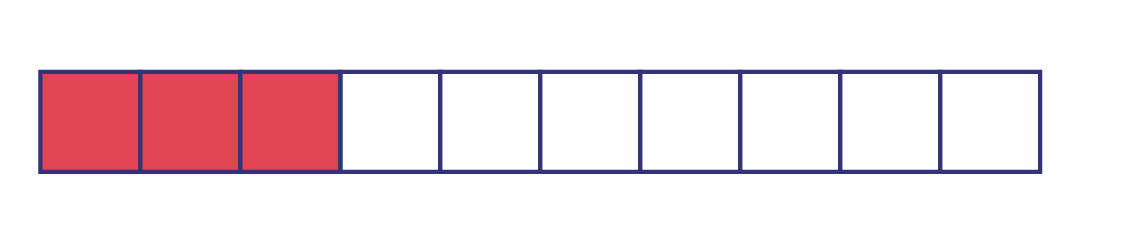
To establish the equality relationship between a fraction whose denominator is not a power of ten (for example, \(\frac{1}{4}\) and the corresponding decimal number, it is necessary to use the concept of equivalent fractions. For example, students can use strips of equal length as shown below to see that \(\frac{1}{4}\) is between \(\frac{2}{10}\) and \(\frac{3}{10}\).

They can then partition the tenths into ten equal parts, thus creating one hundred equal parts, or hundredths of the whole, and recognize that \(\frac{{25}}{{100}}\) is an equivalent fraction to \( \frac{1}{4}\).
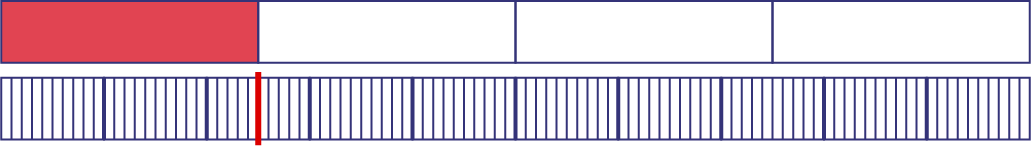
Since \(\frac{{25}}{{100}}\; = \;0.25\), they can conclude that the fraction \(\frac{1}{4}\) can also be represented in decimal notation by 0.25 (\(\frac{1}{4}\; = \;\frac{{25}}{{100}}\; = \;0.25\)).
This type of example allows students to recognize that all fractions that can be expressed as an equivalent decimal fraction can be represented by a decimal number.
| Fraction | Equivalent Decimal Fraction | Decimal Number |
|---|---|---|
| \(\frac{1}{2}\) | \(\frac{5}{{10}}\) | 0.5 |
| \(\frac{3}{4}\) | \(\frac{{75}}{{100}}\) | 0.75 |
| \(\frac{2}{5}\) | \(\frac{4}{10}\) | 0.4 |
| \(\frac{7}{20}\) | \(\frac{{35}}{{100}}\) | 0.35 |
Note: Some fractions (for example, \(\frac{2}{3}\), \(\frac{3}{7}\), \(\frac{5}{{11}}\)) cannot be represented by an equivalent decimal fraction. They can, however, be expressed by repeating decimals (for example, \(\frac{2}{3}\; = \;0.\dot{6}\); \(\frac{3 }{7}\; = \;0.\dot{4}\dot{2}\dot{8}\;\dot{5}\dot{7}\dot{1}\); \(\frac{5}{{11}}\; = \;0.\dot{4}\dot{5}\)) in dividing the numerator by the denominator.
A decimal number represents a fraction whose denominator is a power of 10 (for example, \(0.3\; = \;\frac{3}{{10}}\); \(0.47 \;=\;\frac{{47}}{{100}}\)). As the concept of percent is so closely linked to the concept of fractions, there is only one more step to take to connect the percent, the decimal number and the decimal fraction.
Example
To help students develop this skill, they should be regularly invited to express their answers using another notation. For example, teachers can encourage the student who answered that \(\frac{3}{4}\) of the young people in the class have black hair to also express this answer in decimal notation (0.75) and as a percentage (75%).
Source: translated from Guide d’enseignement efficace des mathématiques de la 4e à la 6e année, Numération et sens du nombre, Fascicule 3, Nombres décimaux et pourcentages, p. 46-51.
Some decimal numbers when converted to a percent result in whole number percents, and others result in decimal percents (for example, 0.15 = 15%, 0.642 = 64.2%, 3.425 = 342.5%).
Percents can be whole number percents (for example, 32%, 168%) or decimal percents (0.5%, 43.6%, 108.75%). Percents can be understood as decimal hundredths.
There are three types of problems that involve percents – determining the percent a quantity represents relative to a whole; finding the percent of a number; and finding a number given the percent.
Source: Ontario Curriculum, Mathematics Curriculum, Grades 1-8, 2020, Ontario Ministry of Education.
Students often mistakenly believe that a percent cannot exceed 100 (100%). However, there are situations in everyday life that lead to percents greater than 100%. To fully understand, these situations can be explored using concrete or visual representations where quantities are related to the whole. Fraction or decimal notation can also be used.
Example
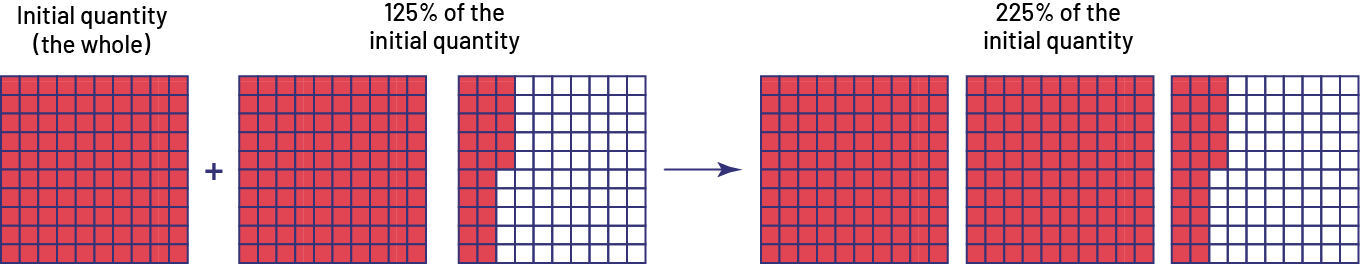
Following a 25% increase, it can be stated that the new quantity is 125% of the original quantity.
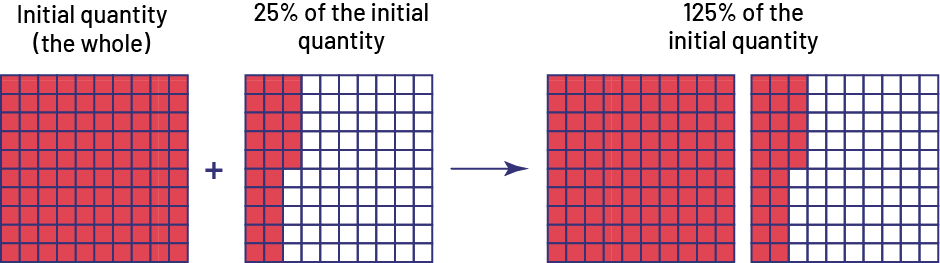
Thus, the new quantity represents \(\frac{{125}}{{100}}\) (one hundred and twenty-five hundredths) of the original quantity or 125% of the original quantity. However, an increase of 125% means that 125% of everything is added to the initial amount. The new quantity then represents \(\frac{{225}}{{100}}\) (two hundred and twenty-five hundredths) of the initial quantity or 225% of the initial quantity.
Knowledge: Percent
Percent is a special kind of a fraction. It is often used in everyday life. A numerical expression such as 30% (which reads "thirty percent") is actually another notation for the number thirty hundredths, or 0.30. To facilitate understanding of the concept of percent, students should first be introduced to the connection between the percent and the fraction with a denominator of 100 using concrete or visual materials.
Example

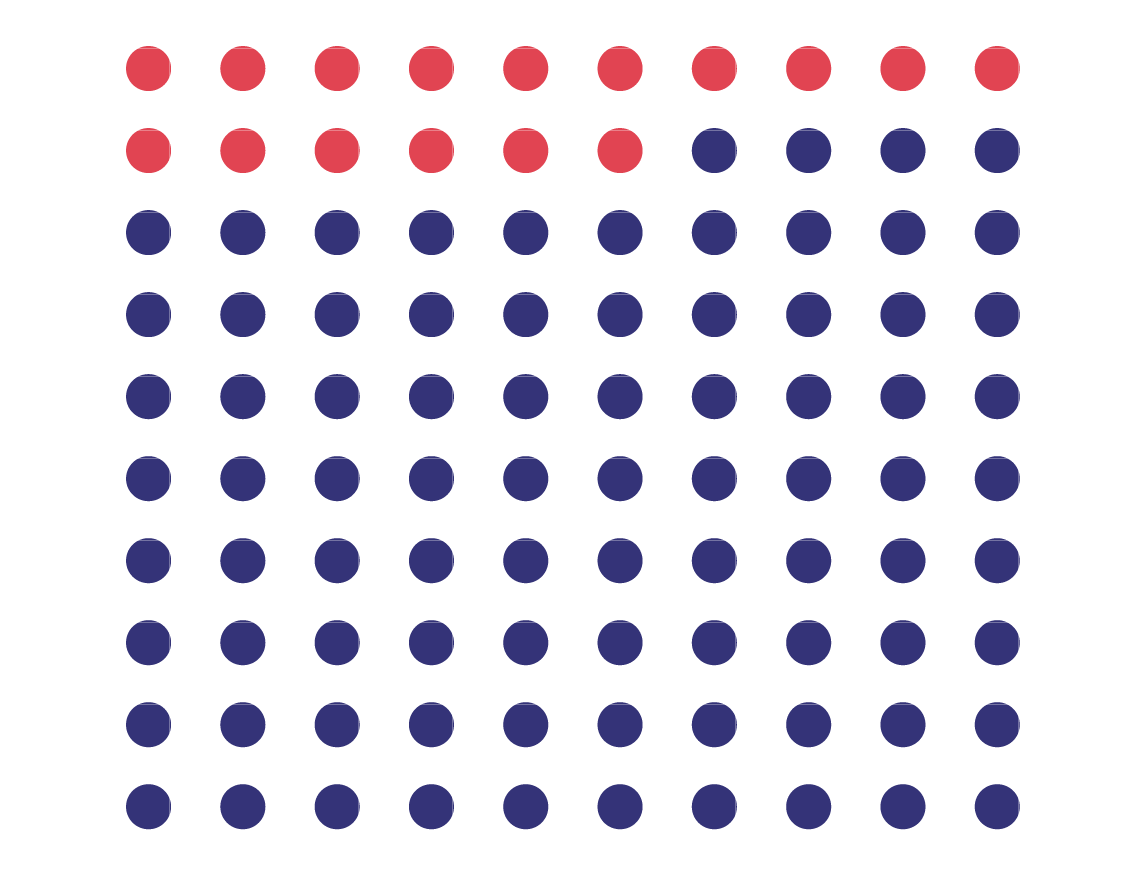
Students should also realize that a percent represents a ratio to 100 (for example, 30% represents the ratio 30:100). It is important to note that a result expressed as a percent does not mean that the quantity in question is necessarily composed of 100 parts, as explained in the following table.
Relationship Between Percent and the Quantity 100
| Representation | Percent | Instructional Notes |
|---|---|---|
  |
75% of the circles are green. | Even if 75% of the circles are green, it does not mean that there are 100 circles in the whole. However, if there were 100 circles, there would be 75 green circles. Also, the fraction of circles that are green is equivalent to \(\frac{75}{100}\) (for example, \(\frac{3}{4}\; = \;\frac{{75}}{{100}}\) and if the amount were doubled the corresponding fraction would be \(\frac{{150}}{{200}}\; = \;\frac{{75}}{{100}}\)). |
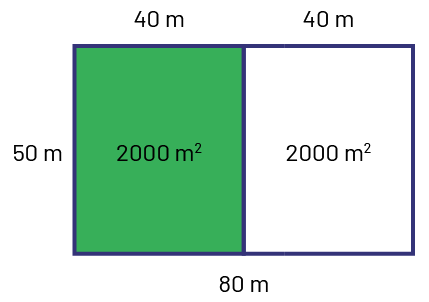 |
50% of the land is covered with grass. | Even if 50% of the field is covered with grass, it does not mean that the field has an area of 100 m2. The entire field is 4000 m2. If half of it is covered with grass, there would be 2000m2 of grass. Thus, \(\frac{2000}{4000} = \frac{1}{2} = \frac{50}{100} = 50\). |
Source: translated from Guide d’enseignement efficace des mathématiques de la 4e à la 6e année, Numération et sens du nombre, Fascicule 3, Nombres décimaux et pourcentages, p. 35.
