B2.4 Add and subtract integers, using appropriate strategies, in various contexts.
Skill: Representing, describing and solve whole number addition and subtraction
In grade7 , students add and subtract integers using a variety of representations (two-coloured tokens, number line) and computational strategies that will allow them to generalize computational rules. The use of two-coloured tokens and the number line is essential to illustrate addition and subtraction of integers. By using these models, students create visual representations of the operations to be performed and can better understand the rationale for the different rules that govern addition and subtraction of integers. Rather than teaching rules, it is better for the student to be able to generalize them as a result of certain observations. The rules for integer computation are the end point, not the beginning point, of teaching and learning about integers.
Source: Les mathématiques... un peu, beaucoup, à la folie, Guide pédagogique, Numération et sens du nombre/Mesure, Grade 7, Module 3, Series 1, Introduction, p. 15.
As with whole numbers, the order in which integers are written in an addition statement does not matter because the commutative property holds true.
However, as with whole numbers, the order in which integers are written in a subtraction statement does matter because the commutative property does not hold true.
When writing an equation, integers are often placed inside brackets and the equation written as \(\ { 3} \; - {(- 2)} \; = \; { 5} \).
- If an integer sign is not included, the number is considered positive.
- These conventions help reduce confusion between the number and the operation.
Source: Ontario Curriculum, Mathematics Curriculum, Grades 1-8, 2020, Ontario Ministry of Education.
Two-Coloured Tokens Model
One colour represents positive numbers and another represents negative numbers.
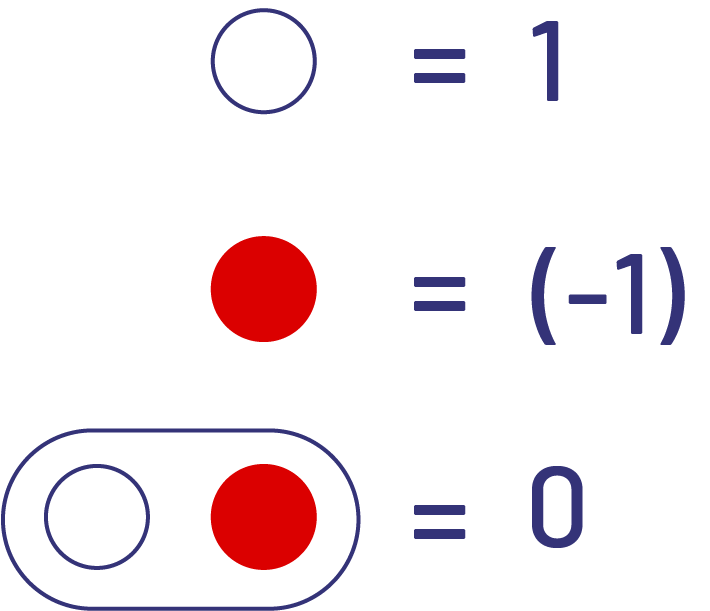
In this model, two tokens of opposite colours cancel each other out or give a zero pair.
Examples
\({}^ - 5\; + \;{}^ + 3\;\)
I represent each term with coloured tokens, that is 5 red and 3 white tokens.
I combine a red token with a white token to get a zero pair. In all, there are 3 zero pairs. This set of tokens represents the integer \({}^ - 2\). So, \({}^ - 5\; + \;{}^ + 3\; = \;{}^ - 2\) ).
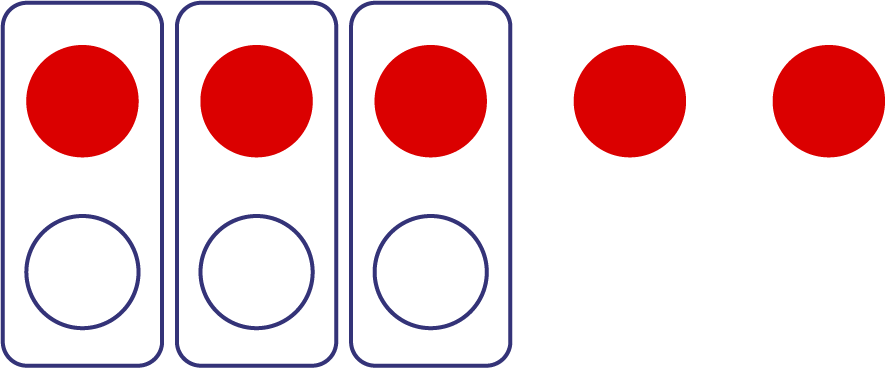
\({}^ - 5\; + \;{}^ - 3\;\)
I represent each term with coloured tokens, that is 5 red and 3 red tokens.
In total, there are 8 red tokens. This set of tokens represents the integer -8. Then, \({}^ - 5; + \;{}^ - 3; = \;{}^ - 8\).
\(5\; - \;{}^ + - 3\;\)
I represent each term with coloured tokens. I have 5 white tokens and 3 red tokens.
I combine a red token with a white token to get a zero pair. In all, there are 3 zero pairs. This set of tokens represents the integer 2. So, \(5\; + \;{}^ - 3\; = \;{}^ + 2\;{\rm{or}}\;{\rm{2}}\).
\(5\; - \;{}^ - 3\;\)
I represent 5 with 5 white tokens. I have to remove -3. If I remove -3, it is like adding its opposite. So I add 3 white tokens.
In total, there are 8 white tokens. This set of tokens represents the integer 8. Then, \(5; - \;{}^ - 3; = \;8\).
Number Line Model
In this model, integers are represented by vectors. The direction of the vector determines whether the value represents a positive or negative quantity. The length (always a positive value) of the vector represents the quantity, and its direction, the sign.
Example
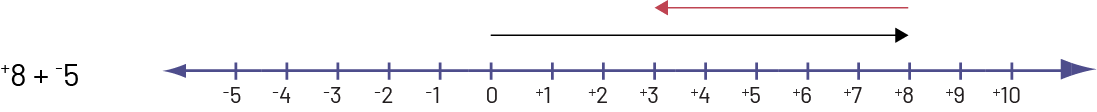
The black vector that is positive (points to the right) represents \({}^ + 8\).
The red vector that is negative (points to the left) represents \({}^ - 5\).
When two positive integers are added together, the result is positive. This can be visualized on a number line as:
- two vectors moving in a positive direction (right or up);
- a vector moving in a positive direction from a positive starting position.
Source: The Ontario Curriculum. Mathematics, Grades 1-8, Ontario Ministry of Education, 2020.
Example
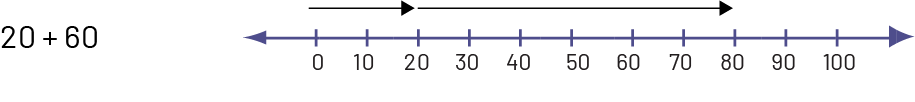
From zero, the student jumps 20 and then adds a jump of 60 so the final position is the sum that equals 80. So, \(60; + \;20; = \;80 \).
When two negative integers are added together, the result is negative. This can be visualized on a number line as:
- two vectors moving in a negative direction (left or down);
- a vector moving in a negative direction from a negative starting position.
Source: Ontario Curriculum, Mathematics Curriculum, Grades 1-8, 2020, Ontario Ministry of Education.
Example
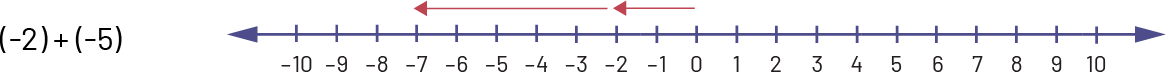
Starting from 0, the student draws a red vector 2 units to the left. From the \({}^ - 2\), they move another 5 units to the left. The student stops at \({}^ - 7\). So, \(\left( {^ - 2} \right)\; + \;\left( {^ - 5} \right)\; = \;{}^ - 7\).
When a positive and a negative integer are added together, the result is negative if the absolute value of the negative integer is greater than the absolute value of the positive integer. This can be visualized on a number line as:
- one vector moving in a positive direction and the other vector with a greater magnitude moving in a negative direction (the sign of the resultant vector is negative);
- a vector moving in a negative direction from a positive starting position with the head of the vector to the left (or below) zero;
- a vector moving in a positive direction from a negative starting position with the head of the vector to the left (or below) zero.
Source: Ontario Curriculum, Mathematics Curriculum, Grades 1-8, 2020, Ontario Ministry of Education.
Example
Starting from 0, the student draws a red vector 6 units to the left. From -6, they move 4 units to the right drawing a black vector. The student stops at \({}^ - 2\). So, \(\left( {^ - 6} \right)\; + \;\left( 4 \right)\; = \;{}^ - 2\).
When a positive and a negative integer are added together, the result is positive if the absolute value of the positive integer is greater than the absolute value of the negative integer. This can be visualized on a number line as:
- one vector moving in a negative direction and the other vector with a greater magnitude moving in a positive direction (the sign of the resultant vector is positive);
- a vector moving in a positive direction from a negative starting position with the head of the vector to the right (or above) zero;
- a vector moving in a negative direction from a positive starting position and the head of the vector to the right (or above) zero.
Source: The Ontario Curriculum. Mathematics, Grades 1-8, Ontario Ministry of Education, 2020.
Example
Starting from 0, the student draws a red vector 3 units to the left. From the \({}^ - 3\) they move 7 units to the right drawing a black vector. The student stops at 4. Then \(\left( {^ - 3} \right)\; + \;\left( 7 \right)\; = \;4\).
The subtraction on the number line can also be seen as the difference between the 2 numbers.
Example
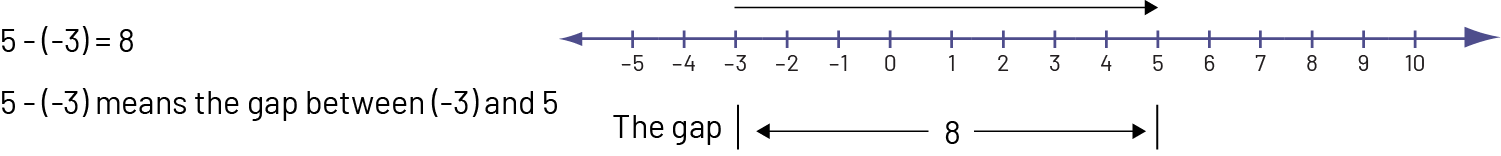
Familiar real-world contexts for negative and positive integers (e.g., temperature, elevators going up and down, parking garages, sea level, golf scores, plus/minus in hockey, gaining and losing money, walking forward and backwards) provide a starting point for understanding adding and subtracting with integers.
Source: Ontario Curriculum, Mathematics Curriculum, Grades 1-8 , 2020, Ontario Ministry of Education.
Example 1
According to the weather report, it is currently \({}^ - 4\;^\circ {\rm{C}}\) in Iqaluit. The temperature will rise by 9 degrees during the day. What is the expected maximum temperature?
Here is an example of a possible solution:
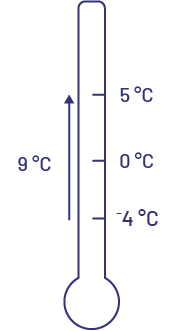
I know that 5°C is 9 degrees warmer than \((- 4)\;^\circ {\rm{C}}\).
Therefore, the maximum temperature expected in Iqaluit is 5°C.
Source: Les mathématiques... un peu, beaucoup, à la folie, Guide pédagogique, Numération et sens du nombre/Mesure, 7e année, Module 3, Série 1, Activité 3 Addition de nombres entiers, p. 82.
Example 2
In the spring, Patrick borrows $75 from his parents to buy a bicycle. During the summer, he saves in order to repay his parents. In September, he gives $25 to his parents. In October, he gives them $30. In what financial situation is Patrick following his loan?
Here are some examples of possible solutions:
Debt: $75 or ({}^ - 75)
Money saved: $55 \(\left( {25\; + \;30\; = \;55} \right)\)or 55
Patrick paid back $55 of the $75 he owed his parents.
\(\begin{align}{}^ - 75\; + \;{}^ + 25\; + \;{}^ + 30\; &= \;{}^ - 75\; + \;{} ^ + 55\\ &= \;\left( {^ - 20\; + \;{}^ - 55} \right)\; + \;{}^ + 55\\ &= \;{} ^ - 20\; + \;0\\ &= \;{}^ - 20\end{align}\)
Patrick still owes his parents $20.
Source: Les mathématiques... un peu, beaucoup, à la folie, Guide pédagogique, Numération et sens du nombre/Mesure, 7e année, Module 3, Série 1, Activité 3 Addition de nombres entiers, p. 78.
Knowledge: Integer
The integers belong to the set \(\Bbb{I}\).
\(\Bbb{I} = \left\{ { \ldots ,\;{}^ - } \right.3,\;{}^ - 2,\;{}^ - 1,\;0,\; 1,\;2,\;3\left.\ldots\right\}\)
Each integer is made up of a number and a sign (\({}^ + \;{\rm{ou}}\;{}^ - \)).
Every integer has an opposite (for example, \({}^ - 3\;{\rm{and}}\;{}^ + 3\)). The opposite of a positive number is a negative number of the same quantity (absolute value), and vice versa.
The signs \({}^ + \;{\rm{and}}\;{}^ - \) are associated with the number. These are not operation signs. Thus, the number \({}^ - 4\) reads: the opposite of 4 or negative 4.
There is no space between the negative sign "\({}^ - \)" and the number 4, since this is not the subtraction operation.
Zero is neither a positive nor a negative number, because it is the only number that is its own opposite.
On the number line, the numbers are in ascending order from left to right and descending order from right to left.
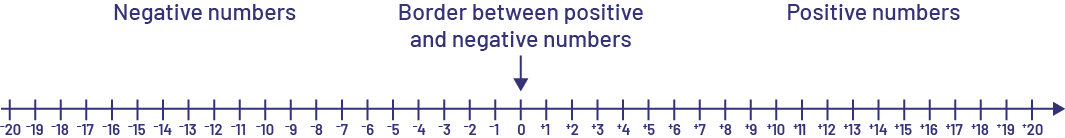
Source: Les mathématiques... un peu, beaucoup, à la folie, Guide pédagogique, Numération et sens du nombre/Mesure, 7e année, Module 3, Série 1, Activité 1 Des nombres entiers sur la droite numérique, p. 35-37.