At a Glance...

B2.6 Multiply and divide fractions by fractions, as well as by whole numbers and mixed numbers, in various contexts.

Activity 1: School Olympics

In this learning situation, students explore the multiplication of a fraction by a whole number in the context of planning school Olympics.

Total duration : approximately 75 minutes

Materials

• Appendix 6.1 (Olympic Circuit)
• Appendix 6.2 (Planning Questions) (1 copy per team)
• Appendix 6.3 (Objectivation) (1 copy per team)
• large sheets of paper (2 per team)
• felt-tip pens

Before Learning (Warm-Up)

Duration: approximately 15 minutes

First, review the addition of fractions by presenting the following situation to the students:

We want to organize an Olympics for the students at the school. One of the events, the endurance race, requires you to practice running over the next few weeks. Since you need to build up your endurance gradually, you will not run the whole distance in the first week.

During the first week, you will only cover \(\frac{3}{{12}}\) the distance. Then, from one week to the next, you will cover \(\frac{2}{{12}}\) more distance. What part of the distance will you run during the second week of training? In which week will you be running the full distance?

Invite students to reflect on the situation. Write equations on the board (for example, \(\frac{3}{{12}}\; + \;\frac{2}{{12}}\; = \;?\)) and discuss strategies that add fractions with like denominators. As a group, determine after how many weeks they will run the full distance. Take this opportunity to review the concepts of proper fraction, improper fraction and mixed number.

Point out that when we add fractions, we add the number of equivalent parts as defined by the denominator (for example, halves, thirds, twelfths). In this situation, it is twelfths of the distance: 3 twelfths + 2 twelfths = 5 twelfths.

Then present the following situation:

I have already mentioned the possibility of having an Olympics this year at school. Obviously, in order for this to happen, we need to plan for it. What do you think we need to consider in order to organize this day?

Record some of the students' suggestions (for example, date, location, number of teams, participants, events, operation, supervisors and equipment).

Note: The purpose of this step is to get students interested in actively participating in the learning situation. In the next step, students will be introduced to a circuit diagram.

Continue by saying:

You have identified several important elements and there are many more. We are only at the planning stage for the Olympics. It is not certain that it will happen. However, if the planning is solid, the project could be completed in some form. We need your help in organizing the Olympics. Today we will consider some of the elements for which decisions have been made. During the Olympics, the events will be distributed in different stations. The teams, previously formed, will circulate from one station to another. Each station will have one to three events in which each student can participate.

Project Appendix 6.1 and ask the following questions:

• What could be the events in the Olympics?
• What events could be held at the same station?

Record some of the students' suggestions (for example, relay race and sprint could be held at Station 1; high jump, long jump and pole vault could be held at Station 7).

Finally, explain to students the task they will have to accomplish:

First, I am submitting some questions (Appendix 6.2) for you to answer in order to advance the planning of the Olympics. Be careful, if you simply perform calculations without thinking about the meaning of the numbers, you may get the answers wrong. Think about what the numbers represent. Next, I will provide you with questions (Appendix 6.3) that will help you objectify the task at hand.

When solving problems, you should consider the following requirements:

• You should only work with fractions, that is you cannot transform any fraction into a decimal number.
• The quotient of the division cannot contain a remainder, so you will have to express it as a fraction.
• The answer must be expressed as a mixed number (for example, \(2\frac{1}{4}\) km) or as a whole number (for example, 2 km) and not as an improper fraction (for example, \(\ frac{9}{4}\) km) or as a decimal number (for example, 2.25 km).

Make sure that the students understand the task at hand by asking questions such as:

• Who can explain the task in their own words?
• Who can summarize the three requirements?
• What is a mixed number?
• What is an improper fraction?

Active Learning (Exploration)

Duration: approximately 40 minutes

Group students in pairs and distribute a copy of Appendix 6.2. Have them complete the task using strategies of their choice.

Allow sufficient time for students to solve and discuss the problems. Remind them to consider the reasonableness of their solution before confirming it.

Note: In order to solve problems A, B, and C, students may recognize that the fraction is multiplied a number of times since the context suggests repeated use of the fraction. To determine the product, students will use their sense of the fraction as well as various representations (for example, illustrations, addition sequences, groupings).

Here are the answers to the problems:

• Activity time : \(8; \times \;\frac{3}{4}\) of a period = 6 periods
• The relay race (1^st, 2^nd, 3^rd grade): \(12\; \times \;\frac{2}{5}\;{\rm{de \; km = }}\;\frac {{24}}{5}\;{\rm{de \; km = }}\;4\frac{4}{5}\;{\rm{de \; km}}\)
• The relay race (4th, 5th, 6th grade) \(10\; \times \;\frac{4}{3}\;{\rm{de \; km = }}\;\frac{ {40}}{3}\;{\rm{de \; km = }}\;13\frac{1}{3}\;{\rm{de \; km}}\)

Circulate and observe the strategies used. Intervene as needed to help certain teams along the way, without explaining how to perform the calculations.

When students have finished solving the three problems, give them a copy of Appendix 6.3 and have them answer the questions.

Note: The purpose of the questions is to encourage objectification and to generate ideas that will be shared in the mathematical exchange. It is not necessary for students to write down everything in detail; they should be able to discuss it. The mathematical exchange will lead them to consolidate their mathematical ideas and to verbalize them.

Assign each team one of the planning questions. Distribute large sheets of paper and markers for each team to record their approach to solving the problem. Tell students that they must also be able to present and explain their approach during the math exchange. Allow students sufficient time to prepare.

Display the different teams' approaches in the classroom, grouping them by question. Allow students to circulate and observe their peers' approaches to see the variety of strategies used.

Consolidation of Learning

Duration: approximately 20 minutes

Invite one or two teams, in the order of the questions, to present their approach to solving the assigned problem. Encourage other students to comment on the presentation and to add to the discussion by suggesting alternative strategies for solving the problem. Facilitate the discussion by asking questions such as:

• Who can explain this team's approach?
• Have other teams used this or a similar strategy?
• How is this strategy similar to another team's?
• Who can explain the multiplication in this problem?
• We often hear that when we multiply, the resulting product is larger than the starting terms. Looking at our problems, is this true? Who can explain this to me?
• A multiplication can be represented by an array. How could it be used to represent the multiplications in the learning situation?

The multiplication of problem A can be represented by the following array.

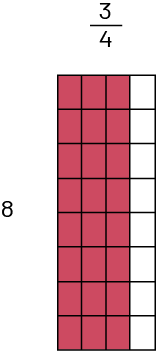
A multiplication can be represented by a set of groups. For example, \(5; \times \;6) can be interpreted as 5 groups of 6 objects. How can this interpretation be applied to the problems in the learning situation?

In problem B, it is 12 sections of \(\frac{2}{5}\) of the path. Then \(12\; \times \;\frac{2}{5}\) can be interpreted as 12 groups of \(\frac{2}{5}\) of the path.

Lead students to notice that each problem involves multiplying a fraction by a whole number, which can be done with repeated addition. Connect the semi-concrete representations to the actions to show the emergence of an algorithm. For example, to perform \(8; \times \;\frac{3}{4}\):

• multiply the numerator (3) by the whole number (8), that is \(8\; \times \;3\;{\rm{quarters}}\;{\rm{ = }}\;{\rm{24 }}\;{\rm{quarters}}\) which corresponds to the improper fraction \(\frac{{24}}{4}\);
• convert the improper fraction (\(\frac{{24}}{4}\) by dividing the numerator (24) by the denominator (4) to determine the solution (6).

Encourage the use of clear and precise arguments and the use of correct vocabulary and causal terms. Examples of reasoning include:

• If in a problem, we notice that a fraction is present a certain number of times, then we can say that the fraction is multiplied by that number.
• When multiplying a fraction by a whole number, the denominator of the product will be the same as that of the fraction. Indeed, we have so many times a certain number of pieces; for example, if we do \(3\; \times \;\frac{3}{4}\), the answer will be read in fourths, because 3 times 3 pieces equals 9 pieces and in this situation, the pieces correspond to fourths, so \(\frac{9}{4}\).
• By calculating \(8\; \times \;\frac{3}{4}\) of a period, we have determined that it will take \(\frac{{24}}{4}\) of period. Since \(\frac{4}{4}\) represents 1 period, then it will take 6 periods (\(24\; \div \;4\)).

End the exchange by presenting a problem such as:

• If problem A stated that there are 15 stations and the time allocated to each is \(\frac{3}{5}\) of a period, how many periods would be required? (9 periods.) How were you able to determine the solution?

Differentiated Instruction

The activity can be modified to meet the needs of the students.

Follow-Up at Home

At home, students solve one or two problems involving the multiplication of a fraction by a whole number using two different representations, for example, an area model (circle, rectangle), a linear model (number line, line segment), an array, or a calculation. They then present their representations to a family member.

Examples

• Serena wants to create a wedding cake that requires the equivalent of 7 cakes. If the recipe calls for \(\frac{3}{4}\) cups of sugar for a cake, how many cups of sugar will Serena need to make the wedding cake?

(\(7\; \times \;\frac{3}{4}\;{\rm{of \; cup}}\;{\rm{ = }}\;\frac{{21}}{4 }\;{\rm{of \; cup \; or \; 5}}\frac{1}{4}\;{\rm{cups}}\))

• Jean likes to walk by exaggerating the size of his steps. By walking in this way, each step allows him to cover a distance of \(\frac{2}{3}\) of a metre. How many metres does he cover in 10 steps?

(\(10\; \times \;\frac{2}{3}\;{\rm{of \; meter}}\;{\rm{ = }}\;\frac{{20}}{3 }\;{\rm{de \; meter \; or }}\;6\frac{{2}}{3}\;{\rm{m}}\))

Source: Guide d'enseignement efficace des mathématiques de la 4e à la 6e année, p. 171-180.

Activity 2: Planning the Olympics - Extension (Multiplying a Whole Number by a Fraction)

Materials

• Appendix 6.4

Recall the previous learning situation. Tell students that they will need to answer more questions related to other elements of Olympics planning.

Group students in pairs and distribute a copy of Appendix 6.4. Have them complete the task using strategies of their choice.

Here are the answers to the problems:

• The big 6th graders race: \(\frac{3}{4}\) of 12 laps = 9 laps
• The great Primary Division race: \(\frac{2}{5}\) of 12 laps = \(\frac{{24}}{5}\) of laps = \(4\frac{{4}} {5}\) rounds
• The teachers’ race: \(\frac{4}{3}\) of 12 laps = \(\frac{{48}}{3}\) of laps = 16 laps

Then, engage in a mathematical exchange. Point out that these problems involve the concept of a fraction of a set. Facilitate the exchange by asking questions such as:

• Who can explain this team's approach?
• Have other teams used this or a similar strategy?
• How is this strategy similar to another team's?
• This group mentions that they divided and then multiplied. Where did this division and multiplication come from? (To determine the fraction of a set, we separate the set by the denominator (division) and identify the number of groups by the numerator (multiplication). The contents of this part then represent the fraction of the set)

Source: Guide d'enseignement efficace des mathématiques de la 4e à la 6e année, p. 180-181.

Activity 3: Let's Share! (Operations on Fractions)

Group students in pairs. Explain that often a quantity that is only a part of the whole, that is, a fraction of the whole, must be shared equally.

Provide each team with two statements and invite students to determine what share (expressed as a fraction of the whole) each person will receive, depending on the situation. Make fractional models (for example, set of fraction circles, set of fraction strips) available to students.

Note: Some students will illustrate the situation, others will use equations, others will represent fractions in their simplest form using equivalent fractions. Students often have difficulty naming the part in terms of the whole; for example, regarding the statement \(\frac{3}{4}\) of a pizza for 2 people, they recognize that each person receives 1 piece and a half piece (\( 3\; \div \;2\)), but they must learn to relate the quantity to the whole in order to recognize that each receives \(\frac{3}{8}\) (fraction) of a pizza (whole).

Select teams based on their strategies and invite them to present their solutions. Compare the various strategies used. Point out that the statements represent the division of a fraction by a whole number, similar to the meaning of equal-sharing (partitive).

Source: Guide d'enseignement efficace des mathématiques de la 4e à la 6e année, p. 182-183.

Activity 4: The Kitchen Staff

This activity allows students to realize that changing the yield of a recipe requires multiplying or dividing quantities that are sometimes expressed as fractions.

The teacher first presents the following situation to the class:

In order to raise funds for their end of year outing, the grade⁵ students at À tire-d'aile School decided to have a bake sale. Florence and her friends chose to make healthy carrot, apple and raisin muffins. When they read the recipe, they realized that one recipe only made 8 muffins. They wanted to make 2 dozen.

Teachers provide students with a copy of the recipe and take the opportunity to draw their attention to the fact that quantities are expressed using imperial measurements.

The teacher groups the students in pairs and asks them to adjust the quantities so that Florence's team can make 2 dozen muffins. The teacher then facilitates a discussion to highlight the difficulties encountered, the strategies used, their differences and similarities. It is important to point out that the temperature and cooking time should never be changed in baking since the size of the muffins has not been changed.

Teachers then suggest that the recipe be reduced and allow time for students to attempt to rearrange the ingredient list to make the desired number of muffins. As with the previous preparation, this exercise is followed by a brief discussion to highlight the strategies used.

Since this is a real recipe, students can be encouraged to make these healthy muffins in class or at home, modifying the amount of ingredients to meet a specific need.

Source: Guide d'enseignement efficace des mathématiques de la 4e à la 6e année, p. 103-104.