E2.5 Use the row and column structure of an array to measure the areas of rectangles and to show that the area of any rectangle can be found by multiplying its side lengths.
Skill: Using the Array to Measure the Area of a Rectangle and Demonstrating That It Can Be Calculated by Multiplying Its Base by Its Height
Relationships Between Base and Height
Exploring these relationships allows students to develop a better understanding of the usual formulas used to determine the area of certain two-dimensional shapes or the volume of certain three-dimensional objects, and to apply them knowledgeably in various problem-solving situations.
Relationships Between Base (Length) and Area
Teachers should introduce students to measurement activities that allow them to relate the dimensions (base and height) of a rectangle to its area. Students should construct the concepts of “height” and “base” through activities that support them in recognizing that any side of a rectangle can be the base and that for every base there is a corresponding “height”.
Rectangle
In Grade 4, students will explore the relationship between the dimensions of a rectangle (including squares) and its area. In later grades, they will use this relationship to establish the area of a parallelogram and the area of a triangle.Example
The teacher provides students with a set of rectangles and a centimetre grid on an overhead transparency. By superimposing the overhead transparency on each rectangle, students determine its base, height and area and record the results in a table.
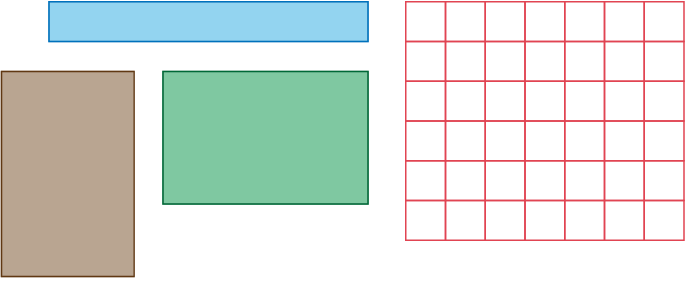
| Rectangle | Base | Height | Area |
|---|---|---|---|
| blue | 6 cm | 1 cm | 6 cm2 |
| green | 4 cm | 3 cm | 12 cm2 |
| brown | 2.5 cm | 4 cm | 10 cm2 |
During the math conversation, the teacher can select different strategies students used to determine area, such as:
- I placed the overhead transparency on the rectangle and counted the squares that cover its surface.
- The area of each rectangle is like an array. I counted the squares in a row and multiplied by the number of rows. For example, in the green rectangle there are 3 rows of 4 squares each.
- I counted the squares in a column and multiplied by the number of columns. For example, in the brown rectangle there are 2.5 columns of 4 squares each.
The teacher then encourages students to formulate a generalization about the relationship between the dimensions of a rectangle and its area, first in words (the area of the rectangle is equal to the product of the measurement of its base and its height), then using mathematical symbols (A = b × h).
Source: translated from Guide d'enseignement efficace des mathématiques de la 4e à la 6e année, Mesure, p. 70-72.
Note: this activity can also be done on a whiteboard and using virtual digital tools.
Knowledge: Area
Area is a measure of the space inside a two-dimensional shape.
The vastness of a piece of land and the area of a country are real-life examples of area.
Fundamental Concepts
Iteration
The student who understands this concept realizes that it is possible to determine the area of a surface by repeatedly layering a single standard or unit of standard or non-standard area in an orderly fashion.
Transitivity
The student who understands this concept realizes that it is possible to determine the area of a surface by repeatedly laying, side-by-side and with no spaces, a single standard or non-standard unit. For example if the area of triangle A is > the area of triangle B, and the area of triangle B > the area of triangle C, then the area of triangle A > the area of triangle C.
Conservation
The student who understands this concept realizes that the area of a surface remains the same whether the surface is moved, transformed, or decomposed.
Additivity
The student who understands this concept realizes that the area of a shape is equal to the sum of the area of its parts.
Structure Associated with the Units of the Area of a Rectangle
The student who understands this concept realizes that the units of area of a rectangle must be juxtaposed in a two-dimensional space, without gaps or overlaps, to cover the rectangle in an array consisting of columns and rows.
Source: translated from Fiche de la 4e à la 6e année, Attribut aire, p. 2-3.
