E1.4 Describe and perform combinations of translations, reflections, and rotations up to 360° on a grid, and predict the results of these transformations.
Activity 1: Get Up and Turn!
This activity integrates concepts from the Spatial Sense and Number strands.
The teacher explains to students that they will represent fractions by rotating their bodies. The teacher asks them to stand up, face the front of the class, and rotate themselves to represent a quarter-turn, half-turn, or three-quarter turn, either clockwise or counterclockwise. The teacher can also ask students to associate the given fraction with the number of degrees (\(90^{\circ}\), \(180^{\circ}\), or \( 270^{\circ}\)) and the type of angle (square, flat, exterior or reflex).
The teacher then asks students to perform more complex rotations (for example \(\frac{1}{6}, \frac{1}{3}, \frac{7}{12}, \frac{15} {16}\) turn) from the same starting position. Before rotating, students should mentally compare the given fraction to a benchmark fraction (for example, \(\frac{1}{6}\) of a turn is less than a \(\frac{1}{4 }\) turn because if two fractions have the same numerator, the smaller fraction is the one with the larger denominator). Students can also indicate whether the angle is acute, obtuse, or reflex.
Source: translated from Guide d’enseignement efficace des mathématiques, de la 4e à la 6e année, Géométrie et sens de l'espace, Fascicule 2, p. 41.
Activity 2: Let's Transform!
Using a dynamic geometry application or software (for example, GeoGebra), have students create a sequence of shapes obtained through various successive transformations, number each image in order, and print out their work (see example below). Students should then exchange papers with another student and determine which transformations were used.
Example
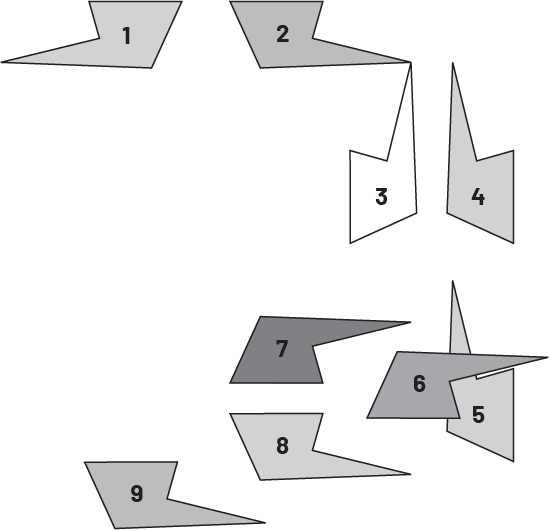
Source: translated from Guide d’enseignement efficace des mathématiques, de la 4e à la 6e année, Géométrie et sens de l'espace, Fascicule 2, p. 77.
