E1.3 Perform dilations and describe the similarity between the image and the original shape.
Skill: Describing the Similarity Between the Image and the Original Shape
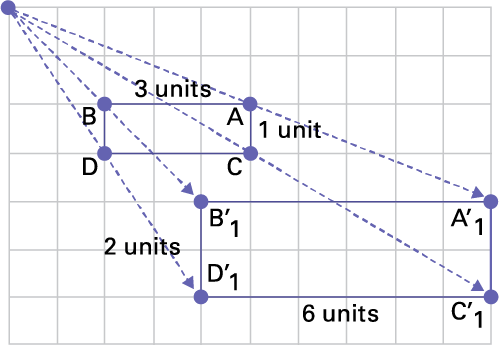
It is important for the student to realize that, unlike translations, reflections, and rotations, a dilation does not produce a congruent image, unless the dilation factor is 1 or −1.
A dilated image is similar to the original. In everyday language, a similar image means it simply resembles something else. In mathematics, similar has a very precise meaning. Similar figures have the same shape (angles are congruent) and their corresponding sides are proportional. If the width of a dilated rectangle is now twice as long, so too is its length.
Dynamic geometry applications are recommended tools for understanding how transformations behave in motion. In a dynamic environment, repositioning the point of dilation has an immediate impact, as does changing the scale factor.
Thus, when we say that shapes are similar, we emphasize that the image shows similarity with the original shape.
- Similarity. Having the same shape but not always the same size. Similar shapes have congruent angles and their corresponding sides are proportional.
- Image. Shape obtained following the transformation of a given original shape.
- Original shape. Figure on which a geometrical transformation is applied.
Source: The Ontario Curriculum. Mathematics, Grades 1-8 Ontario Ministry of Education, 2020.
Knowledge: Dilation
Transformation. A change in a figure that results in a different position, orientation, or size. Transformations include translations (slides), reflections (flips), rotations (turns), and dilations (reductions or enlargements).
Dilation. A transformation that enlarges or reduces a shape by a scale factor to form a similar shape.
Source: The Ontario Curriculum. Mathematics, Grades 1-8 Ontario Ministry of Education, 2020.
