E2.5 Show the relationships between the radius, diameter, and area of a circle, and use these relationships to explain the formula for measuring the area of a circle and to solve related problems.
Skill: Identifying and Using the Relationships between the Radius, Diameter and Area of a Circle to Explain the Formula for Measuring the Area of a Circle
Measuring the area of a circle directly (for example, by placing and counting square tiles and partial square tiles) is not a very accurate or practical method.
Visual proofs use the formulas for the area of a parallelogram or the area of a triangle to demonstrate the logic of the circle formula for area.
To explain the relationship between the radius and area of a circle, a circle can be divided into 16 equal sections, cut out, and then rearranged to form a parallelogram.
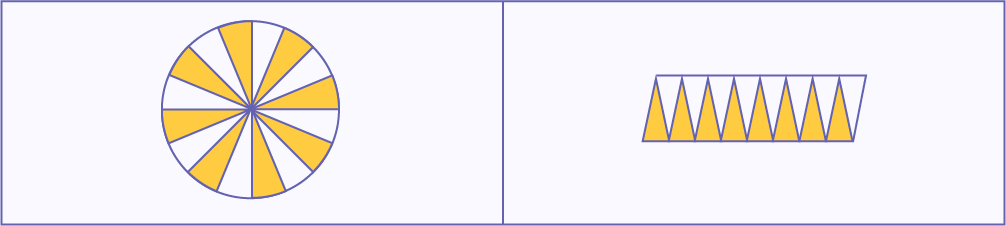
The height of this parallelogram is the radius of the circle (r) and its base is half the circumference of the circle.
Thus, A = \( r \times \frac{2 \pi r}{2}\) or \(\pi r^{2}\).
Source: adapted from The Ontario Curriculum. Mathematics, Grades 1-8 Ontario Ministry of Education, 2020.
Skill: Using the Relationships between Radius, Diameter and Area of a Circle to Solve Related Problems
When solving area-related problems, it is advantageous to work with objects that the student knows.
It is easier to use the radius to calculate the area than the diameter. When determining the radius from the area, students should be sure to first divide the value of the area by the value of pi. It is common for students to forget to multiply by two to find the diameter once the radius has been found from the area.
